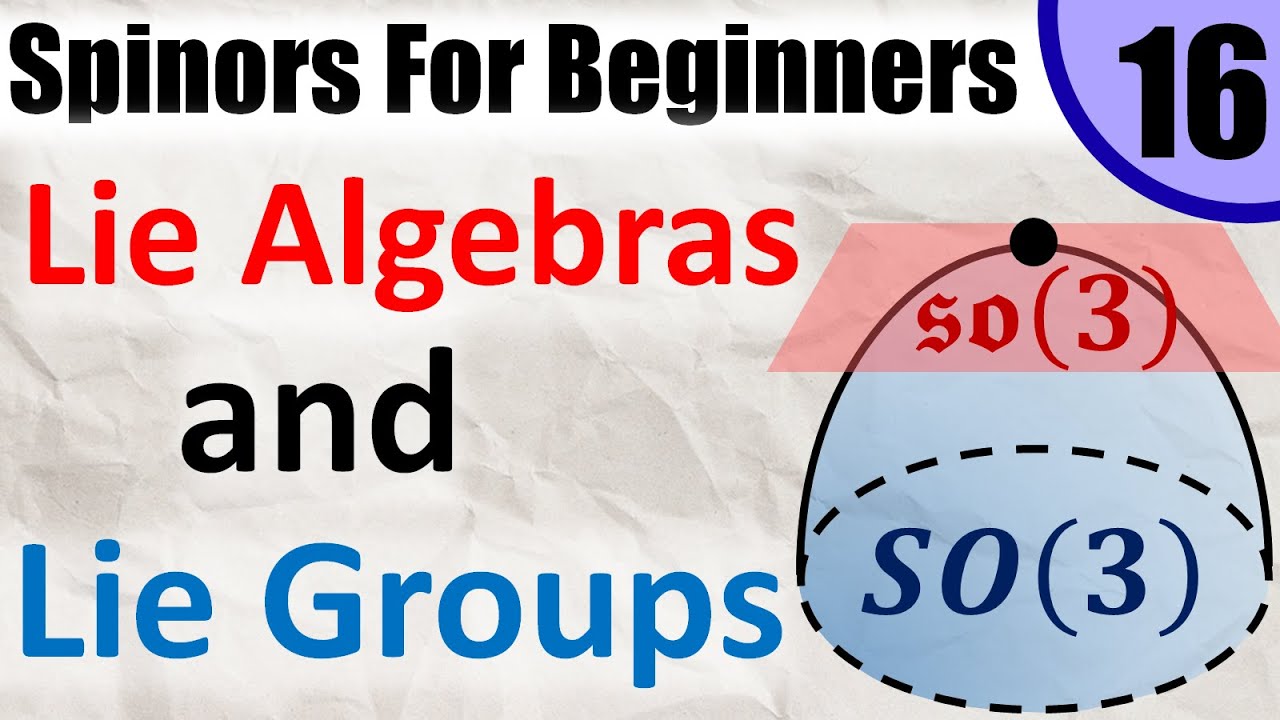
Spinors for Beginners 16: Lie Groups and Lie Algebras

Videos on momentum operator generating translations in quantum mechanics:

0:00 - Introduction
2:45 - Groups & Lie Groups
4:00 - Exponent of a so(3) Matrix
7:40 - Calculating so(3) generators
9:50 - Momentum generators translations
10:58 - so(3) traceless proof
13:35 - so(3) anti-symmetric proof
15:11 - Warning about matrix exponentials
16:43 - Lie Algebra Bracket
20:24 - Structure coefficients
21:48 - Lie Algebras as Tangent Spaces
23:30 - Lie Algebra Property Proofs
30:15 - Summary of so(3)
31:05 - Overview of so+(1,3)
32:50 - Spin-1 and Spin-1/2 representations
34:40 - Math vs Physics conventions

[eigenchris]

At 32:15, I messed up the 2nd last commutation relation on the bottom left. It should be [Ktz, Ktx] = - Jzx.

[richardneifeld]

Your videos keep getting better and better.

[Anonymous-kjcu]

I love your voice. Sounds like a robot.

[baruchspinoza]

Wow! A clear path through something that had appeared as a complex jungle. Thanks EigenChris.

[richardneifeld]

Excellent! Also, I find that mathematician's normally pro ide more comprehensible video lectures than physicists. As of they better understand the underlying principles. I put you in the mathematician lecturer class. Clear and insightful.

[pieterkok]

To find the generator from the group element, you apply the "standard" derivative rule to matrices. However, there are many types of derivatives one can define for matrices (for example Lie brackets, as in the Liouville/Von Neumann equation). Do we need additional justification (other than that it works in this case) for this choice of derivative?

Great series, by the way! 😀

[sair]

came from the joke videos, stayed for the spinors for Beginners 16: Lie Groups and Lie Algebras

[kikivoorburg]

Very excited for this section! I’ve vaguely heard of Lie Algebras (and that they matter in physics) but never had quite a clear picture of what was going on. You have an incredible ability to make complex topics digestible and understandable, so I’m sure you’ll fill those gaps for me!

Edit: I was not disappointed! It’ll take me a bit of time and a few rewatches to fully grasp it I’m sure, but wow have I already learnt so much! I finally get why “the Lie algebra is the tangent space of the Lie group” actually makes sense! Amazing work!!

[kousarshaheen]

Thanks for your videos, it helps me alot in my thesis on particle physics, kindly make sure when we reach particle physics

[breezy]

Great video! However, the summation indices at 8:38 should start at n=1 after taking the derivative.

[AkamiChannel]

Can't wait for the next video! Thanks!

[phitsf]

No offence, but I think your script combined with Taylor Swift doing the reading would make for a badass combination.

[sebastiandierks]

Really great video! Can't wait for the next episode. At 9:28, the group element R_zx should have the minus sign in front of the other sin function if I am not mistaken.

[rhke]

The only video I found that explains why Lie Algebra is important and has a use and how in the field of Quantum mechanics...that is also understandable. Thank you so much

[francoisfjag]

brilliant video. The best explanation I ever get on relationship between a Lie group and its corresponding algebra !!

[HelloWorld-lvwe]

I need a physics expert!

I am a layperson who enjoys physics, and I have a question about spinors. My understanding is that spinors are equivalent to space-time indices (indices on a 4D manifold). Do spinors ever exist on odd-dimensional manifolds? In other words, do spinors occur on even-dimensional manifolds only? I ask this because I’ve heard that bosons are “non-spinorial”, so I’m mainly curious to know whether the force fields are odd-dimensional. If I’m conceptualizing these things wrong, please tell me.

[cmilkau]

Actually, we only proved the properties of an algebra, but not the Jacobi identity to make it a Lie algebra (antisymmetry is obvious). You can prove that this identity always holds when the multiplication (Lie bracket) [a, b] looks like a commutator a·b - b·a and "·" is associative and distributes over +.

[DavidAspden]

Probably one of your best yet. The ground you covered was vast.

[enterprisesoftwarearchitect]

It’s a semester course in 30 minutes!!! ❤️❤️❤️

[Impatient_Ape]

Bravo! Clap! Clap! Clap! This is a really really really good introduction to Lie algebras for motivated undergrad math majors. Lucid, accurate, and well visualized. For those in the comments... a typical math major in the USA might not see this material during their bachelor's degree; however, it is usually part of the graduate math curriculum.