filmov
tv
Mastering Olympiad Algebra | Learn 3 Effective Problem-Solving Methods!
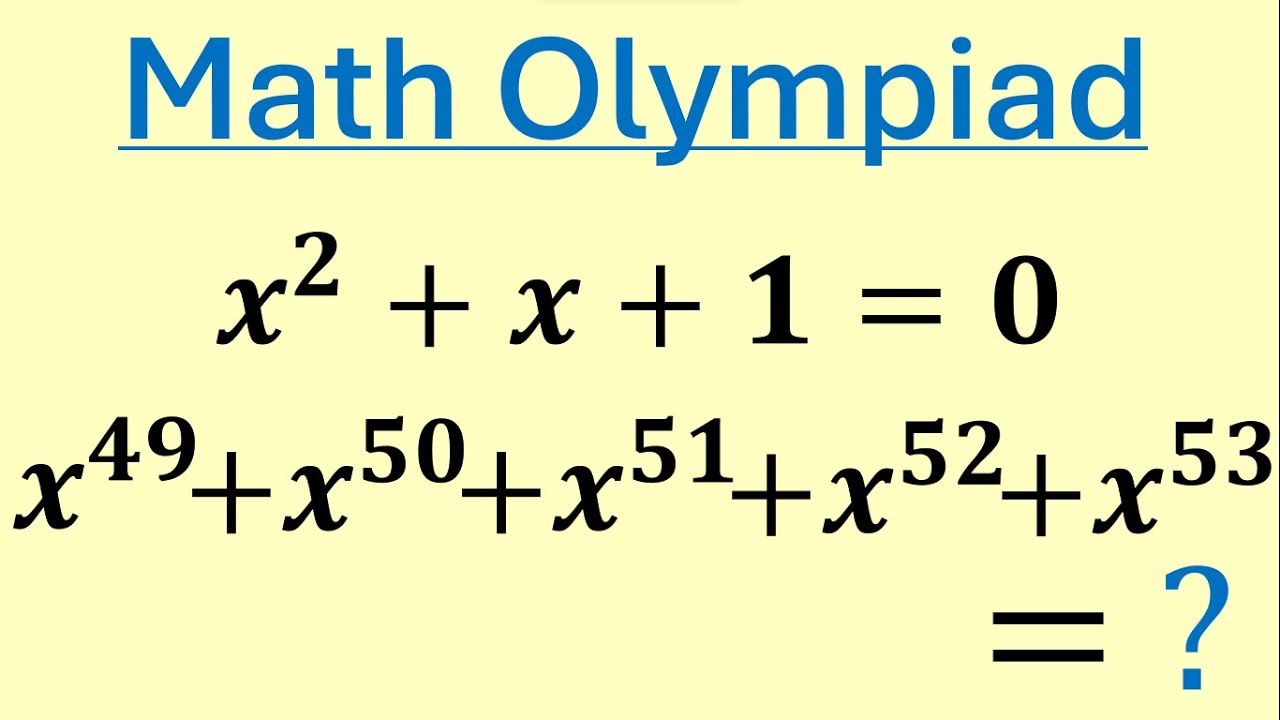
Показать описание
Mastering Olympiad Algebra | Learn 3 Effective Problem-Solving Methods!
Unlock the secrets to conquering Olympiad Algebra with not one, not two, but THREE powerful problem-solving methods! 🏅 In this video, we'll guide you through a journey of mathematical mastery, equipping you with the tools and techniques to solve complex algebraic problems that are often featured in Olympiad competitions. From elegant equations to creative approaches, these three methods will elevate your algebra game to new heights. Join us and let's crack Olympiad Algebra together! 🧮💡 #OlympiadAlgebra #MathCompetitions #ProblemSolving #AlgebraTips #MathChampion #Mathematics #MathOlympiad #AlgebraMastery #MathSkills #CrackTheCode #MathGenius
Topics covered:
Algebra Challenge
Expression
Simplifying Expressions
How to simplify expressions
Complex numbers
Cube root of unity
Math Olympiad
Algebra
Math Tricks
Algebraic identities
Algebraic manipulations
Substitutions
Complex number
Real Solutions
Complex solutions
Quadratic equations
Algebraic Challenging Equations
Difference of cubes
Math Olympiad Preparation
Math Tutorial
9 Key moments of this video:
0:00 Introduction
0:45 Algebraic identity
3:05 Algebraic manipulation
5:15 Squaring
6:18 Complex numbers
6:26 Quadratic formula
6:44 Complex solutions
7:40 Cube root of unity
9:35 Simplifying Expression
#OlympiadAlgebra
#MathCompetitions
#ProblemSolving
#AlgebraTips
#MathChampion
#Mathematics
#MathOlympiad
#AlgebraMastery
#MathSkills
#CrackTheCode
#MathGenius
#MathEducation
#MathEnthusiasts
#AlgebraPuzzles
#MathStrategies
#MathSolutions
#OlympiadPreparation
#MathProblems
#MathTutorial
#MathLearning
#algebra #math #mathematics #mathskills #matholympiadpreparation
Don't forget to subscribe and hit that notification bell to stay updated and smash that like button!
We'd love to hear from you! Did you manage to solve the equation? What other math problems would you like us to cover? Let us know in the comments below!
🎓 Happy learning, and see you in the next video! 🎉
Thanks for Watching !!
@infyGyan
Unlock the secrets to conquering Olympiad Algebra with not one, not two, but THREE powerful problem-solving methods! 🏅 In this video, we'll guide you through a journey of mathematical mastery, equipping you with the tools and techniques to solve complex algebraic problems that are often featured in Olympiad competitions. From elegant equations to creative approaches, these three methods will elevate your algebra game to new heights. Join us and let's crack Olympiad Algebra together! 🧮💡 #OlympiadAlgebra #MathCompetitions #ProblemSolving #AlgebraTips #MathChampion #Mathematics #MathOlympiad #AlgebraMastery #MathSkills #CrackTheCode #MathGenius
Topics covered:
Algebra Challenge
Expression
Simplifying Expressions
How to simplify expressions
Complex numbers
Cube root of unity
Math Olympiad
Algebra
Math Tricks
Algebraic identities
Algebraic manipulations
Substitutions
Complex number
Real Solutions
Complex solutions
Quadratic equations
Algebraic Challenging Equations
Difference of cubes
Math Olympiad Preparation
Math Tutorial
9 Key moments of this video:
0:00 Introduction
0:45 Algebraic identity
3:05 Algebraic manipulation
5:15 Squaring
6:18 Complex numbers
6:26 Quadratic formula
6:44 Complex solutions
7:40 Cube root of unity
9:35 Simplifying Expression
#OlympiadAlgebra
#MathCompetitions
#ProblemSolving
#AlgebraTips
#MathChampion
#Mathematics
#MathOlympiad
#AlgebraMastery
#MathSkills
#CrackTheCode
#MathGenius
#MathEducation
#MathEnthusiasts
#AlgebraPuzzles
#MathStrategies
#MathSolutions
#OlympiadPreparation
#MathProblems
#MathTutorial
#MathLearning
#algebra #math #mathematics #mathskills #matholympiadpreparation
Don't forget to subscribe and hit that notification bell to stay updated and smash that like button!
We'd love to hear from you! Did you manage to solve the equation? What other math problems would you like us to cover? Let us know in the comments below!
🎓 Happy learning, and see you in the next video! 🎉
Thanks for Watching !!
@infyGyan
Комментарии
 0:10:36
0:10:36
 0:08:23
0:08:23
 0:09:06
0:09:06
 0:12:05
0:12:05
 0:02:57
0:02:57
 0:03:06
0:03:06
 0:08:32
0:08:32
 0:06:48
0:06:48
 0:29:17
0:29:17
 0:03:12
0:03:12
 0:06:04
0:06:04
 0:00:56
0:00:56
 0:03:22
0:03:22
 0:13:01
0:13:01
 0:00:25
0:00:25
 0:02:28
0:02:28
 0:06:59
0:06:59
 0:00:58
0:00:58
 0:00:40
0:00:40
 0:00:59
0:00:59
 0:03:59
0:03:59
 0:16:08
0:16:08
 0:08:26
0:08:26
 0:06:33
0:06:33