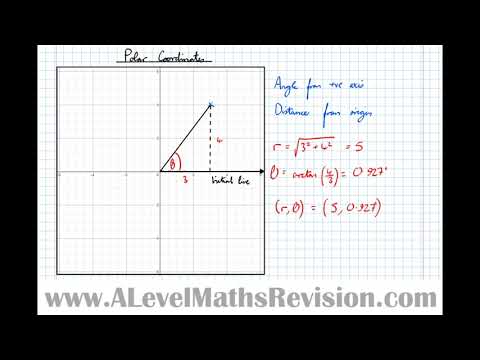
Intro to Polar Coordinates

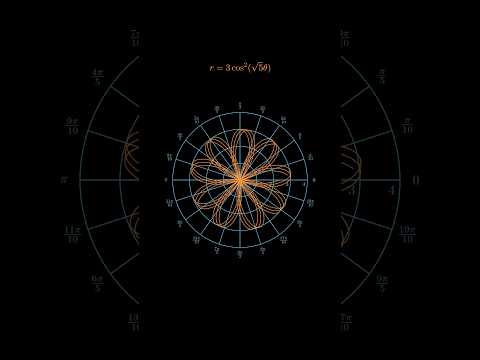
Polar Coordinates are great for functions with lots of periodic symmetry to them like sin and cos. In this video we will describe the new coordinate system, contrast it with the familiar Cartesian coordinate system, and derive the formulas to convert between Cartesian and Polar. Finally, we'll investigate what "gridlines" will become in the new coordinate system.

****************************************************
YOUR TURN! Learning math requires more than just watching videos, so make sure you reflect, ask questions, and do lots of practice problems!
****************************************************

****************************************************
Other Course Playlists:

***************************************************

► Want to learn math effectively? Check out my "Learning Math" Series:

►Want some cool math? Check out my "Cool Math" Series:

*****************************************************

*****************************************************

*****************************************************

This video was created by Dr. Trefor Bazett

BECOME A MEMBER:

MATH BOOKS & MERCH I LOVE:

[amber]

Your content is seriously just amazing, and I truly appreciate how much effort you put in to these videos, let alone your consistency. I've watched so many videos without commenting, so I just wanted you to know that it doesn't go unnoticed and I'm sure many others feel the same. Please keep it up!

[itsonlystars]

Finally after several decades, I understand how this all fits together! Thanks!

[carolinavendano]

Trefor, your great content has become the base for my university Calculus class. Now that we have to take our lectures remotely, resources like the ones you create have helped us understand all the concepts. My whole class is advised to watch your videos. Thank you so much.

[lifeforever]

The Only Video on Polar Coordinates ❤❤❤

[a.koomson]

Trefor, you're seriously doing God's work. The way you explain this concept makes it feel so tangible. From this lesson, I learned that the actual plane being examined remains fixed, however you can place different coordinate systems onto the plane, which will in effect change the way you describe different locations on the plane. The implications of this idea is so cool and mind opening. Thanks lots.

[pranavrohan]

best video to clear all your concepts on this cartesian and polar coordinates! thanks, Mr. Trefor that was a great video.

[yogeshpx]

Thanks a lot, Great job!! thanks for being my teacher

[jimmygore]

Once again I am commenting how amazing your lessons are, Students around the world will be better off and more knowledgeable because of you

[kaweaakuna]

You know what's crazy? I listen to you for a few seconds and get what I was looking👀 for💎 Again thanks💯 for helping me remember😂😂😂😂😂😂

[panagiota]

Another really helpful video!!! You truly reveal the beauty of maths. (P.S. where can I find more info about these special triangles in trigonometry? I found them fascinating :))

[kaweaakuna]

Apple pie + 😂😂😂😂😂😂😂 Cartesian🔥🔥🔥🔥🔥🔥 You don't even know what a blessing your channel has been in helping me out💯

[MohammadRezaFallahzade]

High Quality Content Like Always . Thank You.

[martincdt]

Really useful, thanks for the knowledge !

[viniciusdeobber]

Great content professor, thank you for helping me on calc 3

[akshaj]

recommended video for last minute preparation, covers the required knowledge within a span of 10mins gg

[petruspetrusuom]

Obviously you understand what you say.. which is quite rare. Good video.

[wolffang]

Thank you for the video clearing up a difficult concept!

[madhumithatk]

This is the best:-)Thanks a lot Mr.Trefor😍

[markkennedy]

If we use a polar coordinate system whose origin is either 1) moving with uniform velocity or 2) accelerating or 3) itself moving around another fixed point, can we use Newton's second law in the r hat and theta hat directions.

I suspect we can still do so in case 1) but not if it's accelerating in 2) and 3).

If not, how would we deal with such a system with an accelerating origin: I'm thinking something like a spinning ride on a carousel.

Hopefully you can comment on this.
Thanks.

[xczflls]

Very Nice, Please Dr. I want to ask you about the program that you used to illustrate the lessons how we can draw the angle same as you did, how do I make it move like this