Euler's Gem formula in arbitrary dimension

Here was the abstract:

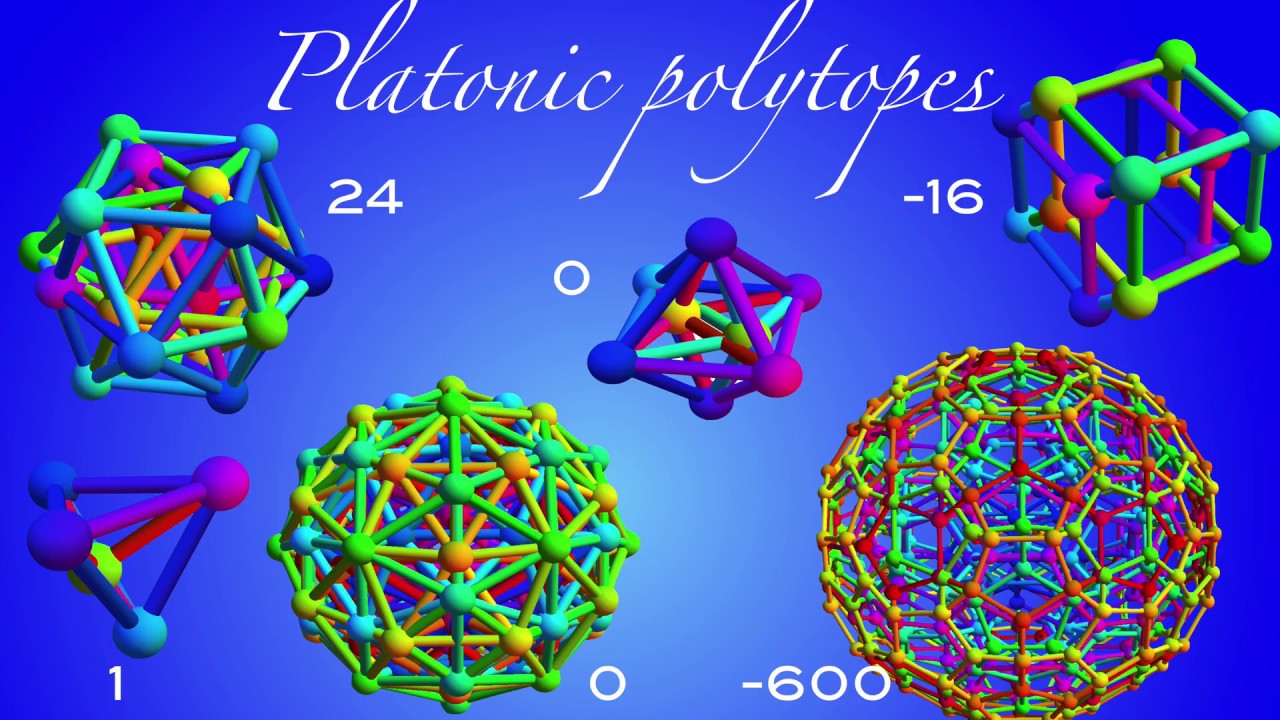
Just before Euler's day (2/7/18) it is appropriate to look at one of the most beautiful formulas in Mathematics, the so called Euler Gem v - e + f = 2. Less well known is that this formula has historically been proven wrong again and again, as counter examples have turned up, like Kepler solids. This was such an embarrassment for Mathematics, that Imre Lakatos suggested that theorems do to "evolve" like species, maybe never really reaching their ultimate final form. In this talk we aim to refute Lakatos' hypothesis and give a crystal clear proof of the formula in arbitrary dimensions: the Euler characteristic of a discrete sphere is 1 + (-1)^d. The Euler Gem case is d = 2. Understanding the proof does not require Euclidean space and is inductive. The key is to define in a precise way, and entirely combinatorially, what a discrete d-dimensional sphere is. The quest to do that within finite mathematics has only started in the 20th century with Hermann Weyl, and Euler plays also here an important role as he initiated linking topology with combinatorics. If time permits, the classification of all the Platonic solids in arbitrary dimension will be derived. While known in the case d = 2 already in ancient Greece, the higher dimensional case has been tackled first by rather unusual mathematicians: by Ludwig Schlaefli, who was a high school teacher at first or by Alicia Boole Stott, the princess of Polytopia.