filmov
tv
'Fermat's Enigma' By Simon Singh

Показать описание
Fermat's Enigma, written by Simon Singh, is a captivating and meticulously researched work that delves into the intriguing mathematical puzzle known as Fermat's Last Theorem. Singh skillfully combines historical accounts, mathematical explanations, and personal anecdotes to create a comprehensive narrative that engages both experts and laypeople alike.Singh's writing style is clear and accessible, making complex mathematical concepts understandable for readers with varying levels of mathematical expertise. He seamlessly weaves together historical and biographical elements, providing valuable context to the mathematical puzzle at the heart of the book. By introducing us to the lives of mathematicians such as Pierre de Fermat, Leonhard Euler, and Andrew Wiles, Singh humanizes the subject matter and brings it to life.The book begins with a detailed exploration of the enigmatic figure behind the theorem, Pierre de Fermat. Singh effectively paints a picture of Fermat as a passionate and enigmatic mathematician, whose marginal annotations in a book sparked a mathematical revolution. He also highlights Fermat's brilliance and the lasting impact of his contributions to number theory. This historical foundation serves as a solid backdrop for the subsequent exploration of the mathematical problem itself.As Singh dives into the mathematical intricacies of the theorem, he employs a skillful blend of logic and storytelling. He presents the readers with the problem itself and its historical significance, gradually introducing the necessary mathematical concepts and techniques. By interweaving historical anecdotes and personal experiences, such as his interviews with mathematicians working on the problem, Singh keeps the readers engaged and invested in the outcome.Furthermore, Singh adeptly captures the sense of suspense and urgency surrounding Fermat's Last Theorem. He discusses the various failed attempts and false proofs that emerged over the centuries, heightening the anticipation for a definitive solution. The book's structure builds up the tension, leading to the introduction of Andrew Wiles, the mathematician who would eventually crack the puzzle.The portrayal of Wiles is particularly compelling, as Singh depicts the immense dedication and intellectual struggle Wiles faced in his quest to solve Fermat's Last Theorem. Wiles's story serves as a powerful example of the pursuit of knowledge and the lengths to which individuals will go to unravel the mysteries of the universe. Singh effectively conveys the emotional and psychological toll that mathematical research can exact on its practitioners.In addition to the captivating storytelling, Singh also provides readers with a glimpse into the world of mathematics as a discipline. He emphasizes the collaborative nature of mathematical research, highlighting the importance of peer review and the exchange of ideas. Singh explores the role of mathematical societies and conferences in fostering collaboration and creating an environment conducive to groundbreaking discoveries.Overall, Fermat's Enigma is a masterful blend of history, biography, and mathematics. Simon Singh's engaging narrative style, combined with his meticulous research, allows readers to grasp the intricacies of Fermat's Last Theorem and appreciate the magnitude of its solution. Through his vivid storytelling, Singh successfully conveys the excitement, challenges, and beauty of mathematical exploration, making Fermat's Enigma an enriching and enjoyable read for both mathematicians and non-mathematicians alike.
Комментарии
 0:19:17
0:19:17
 0:09:31
0:09:31
 0:04:47
0:04:47
 0:11:14
0:11:14
 0:03:57
0:03:57
 0:13:36
0:13:36
 0:01:25
0:01:25
 0:04:53
0:04:53
 0:17:36
0:17:36
 0:01:44
0:01:44
 0:02:52
0:02:52
 0:01:29
0:01:29
 0:02:56
0:02:56
 0:00:52
0:00:52
 0:02:43
0:02:43
 0:07:22
0:07:22
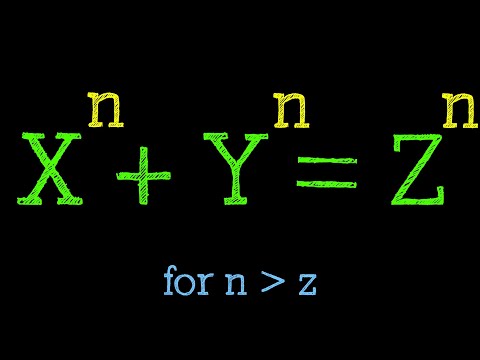 0:02:00
0:02:00
 0:05:14
0:05:14
 0:04:32
0:04:32
 0:10:46
0:10:46
 0:12:09
0:12:09
 0:16:06
0:16:06
 0:09:31
0:09:31
 1:20:21
1:20:21