filmov
tv
SHOW THAT THE FUNCTION w=e^x(cosy+i siny) is Analytic, Hence find the derivative #e^x(cosy+isiny)
Показать описание
Functions of Complex variables| w=e^x(cosy+i siny)| find f'(z).
0:06:32
SHOW THAT THE FUNCTION w=e^x(cosy+i siny) is Analytic, Hence find the derivative #e^x(cosy+isiny)
0:04:46
@btechmathshub7050S.T f(z)=e^x(cosy+isiny) is Holomorphic(Analytic) n find its derivative
0:02:18
Cauchy Riemann show that f(z) = e^x(cosy + isiny) is holomorphic
0:04:22
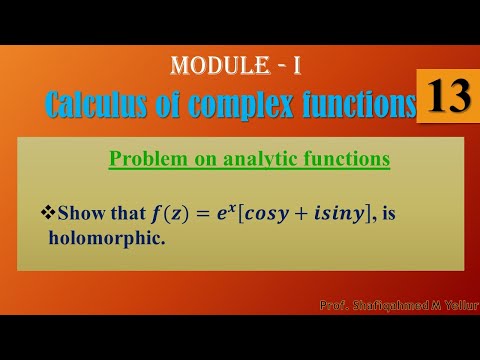
#13 || Problem#5 || Show that 𝒇(𝒛)=𝒆^𝒙 [𝒄𝒐𝒔𝒚+𝒊𝒔𝒊𝒏𝒚] is holomorphic || Complex Function || 18MAT4 ||...
0:06:54
@btechmathshub7050S.T u=e^x.cosy is harmonic. Find conjugate V n analytic function
0:09:48
Analytic: Show that the function f(z)=sin x cosy + icosx sinhy is an analytic at every point.
0:10:21
@btechmathshub7050If v=e^x(xsiny+ycosy) is given find analytic function
0:20:02
#24 || Problem#2 || 𝒖=𝒆^𝒙 𝒙𝒄𝒐𝒔𝒚−𝒚𝒔𝒊𝒏𝒚 ||Harmonic conjugate || Analytic function f(z) ||18MAT41 ||...
0:10:30
@btechmathshub7050U=e^x(xcosy-ysiny) find imaginary part of f- Milnes's Thomson Method
0:08:25
@btechmathshub7050If u-v=e^x(cosy-siny) find an analytic function.
0:07:40
If f(z) =u+iv is analytic and u-v =e^x (cosy-siny), find f(z) || complex analysis bsc maths
0:02:11
u = e raz power x ( x cosy- y siny) Laplace's eq. Find analytic fun. (C A - I- 7.H R BHAGAT.)
0:07:28
Easily explain how to find analytic function f(z) given real part by easy maths easy tricks
0:04:54
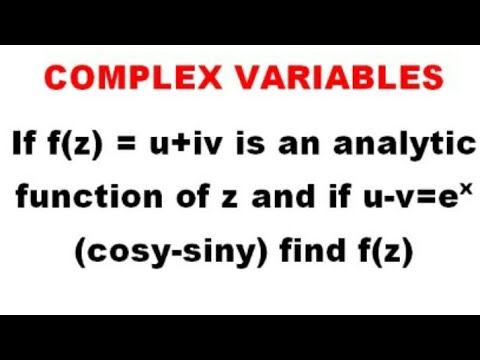
If u=e^x(xcosy-ysiny), then find the analytic function u+iv. || complex analysis ||
0:05:13
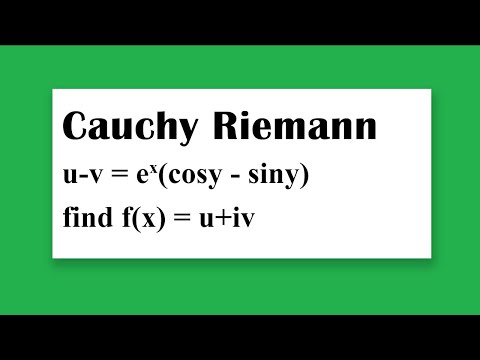
Cauchy Riemann u-v = e^x (cosy - siny) find f(x) = u+iv
0:24:41
If Cosy=xcos(a+y), show that y=cot^-1((xsina)/(xcosa-1)) and dy/dx =k/(1+x²-2xcosa) if k=sina
0:22:31
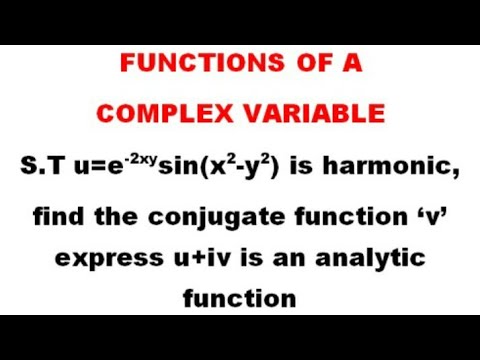
@btechmathshub7050U=e^-2xysin(x²-y²) is harmonic,find conjugate function and analytic function
0:01:29
Partial Derivatives of z =e^(xy)
0:09:18
If (u-v)=e^x(cosy-siny) then find analytic function f using Milne Thomson method || By Ankana Roy ||
0:06:16
How to Evaluate the Line Integral of a Vector Field
0:05:25
Complex Variable| Find constant if f(x) is analytic|
0:01:32
Partial Derivative of z = cos(xy)
0:00:26
Fortnite SWEATS in 2018 vs 2023…
0:32:03
Complex Analysis #2 (V.Imp.) | Checking Analytic Function | Verifying Cauchy Riemann Equations
Комментарии