filmov
tv
The Axiom of Choice | Epic Math Time

Показать описание
The axiom of choice states that the cartesian product of nonempty sets is nonempty. This doesn't sound controversial, and it might not even sound interesting, but adopting the axiom of choice has far reaching consequences in mathematics, and applying it in proofs has a very distinctive quality.
Special thanks to my Channel Supporters: Dru Vitale, RYAN KUEMPER, AlkanKondo89, John Patterson, Johann, Speedy, Zach Ager, Joseph Wofford, and Rainey Lyons.
My current equipment for making videos (affiliate links):
Special thanks to my Channel Supporters: Dru Vitale, RYAN KUEMPER, AlkanKondo89, John Patterson, Johann, Speedy, Zach Ager, Joseph Wofford, and Rainey Lyons.
My current equipment for making videos (affiliate links):
The Axiom of Choice
What's so wrong with the Axiom of Choice ?
How the Axiom of Choice Gives Sizeless Sets | Infinite Series
Death by infinity puzzles and the Axiom of Choice
The Axiom of Choice
The Axiom of Choice | Epic Math Time
Does math have a major flaw? - Jacqueline Doan and Alex Kazachek
How ISPs Violate the Laws of Mathematics
The Axiom of Choice: History, Intuition, and Conflict
What is...the axiom of choice?
Why we take the axiom of choice
Axiom of Choice, 13 Essence of Set Theory
The Axiom of Choice.
An impossible game at the heart of math
Set Theory | Lesson 9: Axiom of Choice [CC]
Ep -1: The Axiom of Choice
What is the Axiom of Choice?
Axiom Of Choice - Valeh - Beyond Denial - X DOT 25 Music
6. The Axiom of Choice
When mathematicians realize that the Axiom of Choice implies Banach-Tarski #math #axiomofchoice
Choice Functions & The Axiom of Choice | Nathan Dalaklis
The Axiom of Choice Part 1
Math400 - Functional Analysis - Section 0.1 - The axiom of choice
Ed Lopes - Axiom Of Choice (Official Video)
Комментарии
 0:32:47
0:32:47
 0:04:50
0:04:50
 0:13:20
0:13:20
 0:12:30
0:12:30
 0:08:50
0:08:50
 0:06:26
0:06:26
 0:05:20
0:05:20
 0:06:08
0:06:08
 0:08:24
0:08:24
 0:17:12
0:17:12
 0:00:57
0:00:57
 0:15:45
0:15:45
 0:06:41
0:06:41
 0:16:31
0:16:31
 0:09:24
0:09:24
 0:16:17
0:16:17
 0:00:53
0:00:53
 0:04:07
0:04:07
 0:13:41
0:13:41
 0:00:06
0:00:06
 0:03:26
0:03:26
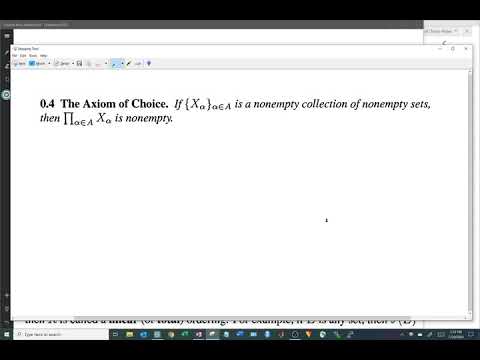 0:18:39
0:18:39
 0:50:46
0:50:46
 0:04:08
0:04:08