filmov
tv
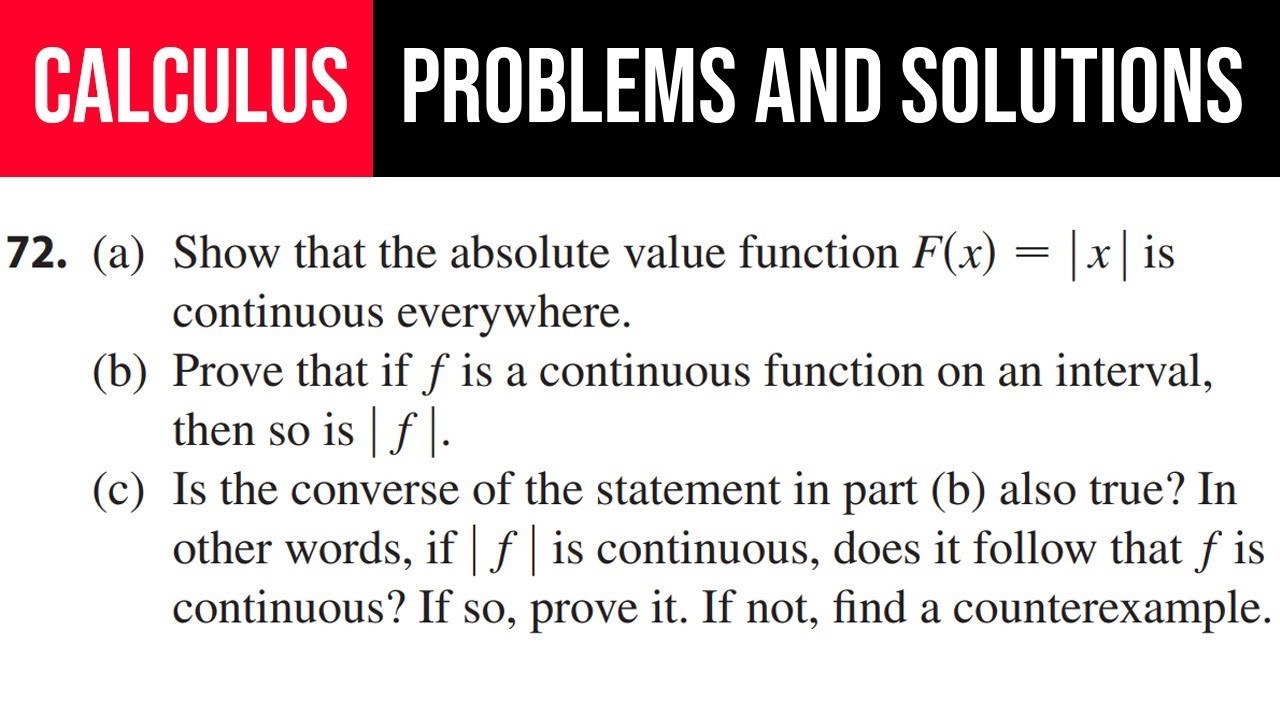
72. (a) Show that the absolute value function F(x)=|x| is continuous everywhere.
Показать описание
72.
(a) Show that the absolute value function F(x)=|x| is continuous everywhere.
(b) Prove that if f is a continuous function on an interval, then so is |f|.
(c) Is the converse of the statement in part (b) also true? In other words, if |f| is continuous, does it follow that f is continuous? If so, prove it. If not, find a counterexample.
Calculus: Early Transcendentals
Chapter 2: Limits and Derivatives
Section 2.5: Continuity
Problem 72
10/1/2024 - 5,263 Subscribers - 1,188,683 Views
(a) Show that the absolute value function F(x)=|x| is continuous everywhere.
(b) Prove that if f is a continuous function on an interval, then so is |f|.
(c) Is the converse of the statement in part (b) also true? In other words, if |f| is continuous, does it follow that f is continuous? If so, prove it. If not, find a counterexample.
Calculus: Early Transcendentals
Chapter 2: Limits and Derivatives
Section 2.5: Continuity
Problem 72
10/1/2024 - 5,263 Subscribers - 1,188,683 Views
 0:44:18
0:44:18
 0:06:59
0:06:59
 0:43:01
0:43:01
 0:01:01
0:01:01
 2:21:31
2:21:31
 0:04:57
0:04:57
 0:37:19
0:37:19
 0:37:18
0:37:18
 0:18:33
0:18:33
 0:37:23
0:37:23
 0:01:57
0:01:57
 0:36:39
0:36:39
 0:03:10
0:03:10
 0:09:18
0:09:18
 0:20:43
0:20:43
 0:34:57
0:34:57
 0:11:21
0:11:21
 0:34:33
0:34:33
 0:01:55
0:01:55
 0:03:16
0:03:16
 0:00:39
0:00:39
 0:10:02
0:10:02
 0:33:03
0:33:03
 0:01:10
0:01:10