The Finite Element Method (FEM) | Part 4: Transformation Matrix and Trusses

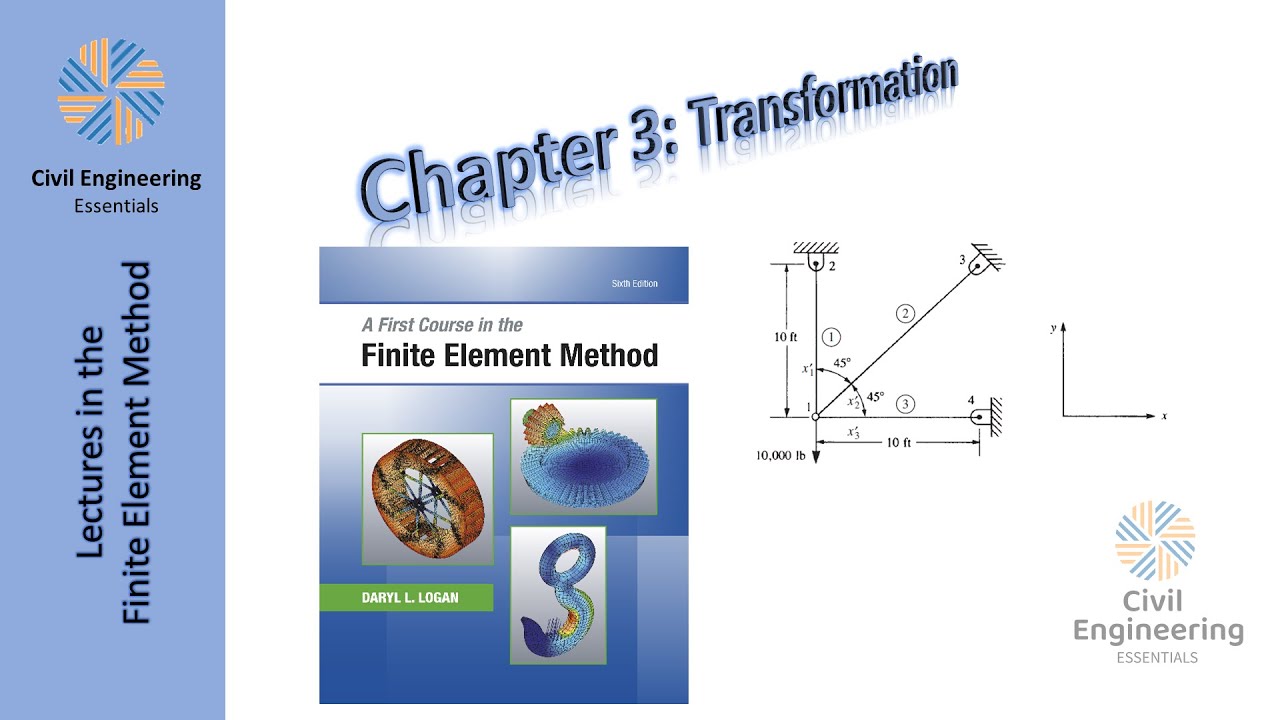
In this video, we will be checking out chapter 3 of the book "A first course in the finite element method". With emphasis on Transformation Matrices.
Learn the fundamentals of truss elements in tutorial. We'll cover the Transformation Matrix and use it to find stresses and global stiffness matrix with a step-by-step example to help you understand the concepts better. Don't forget to check the timestamps below for easy navigation!

Whether you're a beginner or an experienced engineer, this tutorial will provide you with a solid understanding of the direct stiffness method and how to apply it to solve structural analysis problems. So, sit back, relax, and let's get started!

You can join the ranks of our members via the link:

Timestamps:
0:00 Introduction
0:40 Transformation Matrix
22:20 Stresses
23:33 Example
36:39 Outro

#Finite_Element_Method
#FEM
#engineering
#science
#numerical_methods
#discretization
#interpolation
#numerical_integration
#software
#modeling
#analysis
#boundary_conditions
#a_first_course_in_the_finite_element_method
#daryl_l_logan

[donaldkhanye]

Hello Dr. CEE

What a ride this lecture has been! and although it may not seem so on the surface, for me this lecture and the example are well packed with core principles and the associated assumptions! There is so much to take-away, and there is so much to unpack. Let me settle for the CEE classic saying that ^_^ TRUSS elements do not care about LOCAL nodal forces and their corresponding nodal displacements in the local y, (v) directions. Nicely done Doctor and thank you.

The inclusion of the example (the juicy one you say ^_^) and the occasional “slow-playing” through select parts of the example was brilliantly done. It does help a lot to confirm and to make sense of the various theoretical aspects that had been learned; it also booster confidence on the application of what had been learned.

34:13 For the answer to the second part of the example. I first used the same process that is explained in this lecture and then I decided (for the heck of it, I mean why not?) to also double-check my answers to this second part by using the transformation relationship {f’} = [T]{f} …. boy oh boy I was in for it. I quickly (maybe not so quickly ^_^) learned that one must first separate the “STRUCTURE” GLOBAL nodal forces from the “ELEMENT” GLOBAL nodal forces especially where more than one element joins together at a common node like at node #1 in this case. In the end, all worked out and the answers from either process were matched as it was anticipated including the force signs.

36:55 I support the CEE idea to have the next video to be yet another example to cement the many cool and interesting ideas that were unpacked on this video. A lot was unpacked, so why not look at the various practical applications and illustrations of these techniques and/or processes in action if we can.

I have enjoyed this video and I am looking forward to the next videos

Regards, DK

[jesuscandea]

These lectures are awesome, and raised me a new question, how do i know in which case i should consider large displacements calculations? Thank you very much as always

[bilal.akkaya]

I think the solution has a problem at the length of the 2. element in the question, it must be 10*sqrt(2) from the pythagorean relation. Btw thank you for the tutorials, it helps really much. 🙏🙏