filmov
tv
Discrete Mathematical Structures, Lecture 3.4: Divisibility and primes

Показать описание
Discrete Mathematical Structures, Lecture 3.4: Divisibility and primes.
We say that an integer d divides n if n=dk for some integer k. A integer p greater than 1 is prime if p=ab implies either p=a or p=b. We prove that this is equivalent to the more classic "grade school definition" of p having only two (positive) divisors. We show that divisibility is transitive, and how this means that every positive integer is divisible by a prime. We state, without proof, the fundamental theorem of arithmetic, which says that every integer greater than 1 has a unique prime factorization. Though this seems obvious, we concluding by showing how this actually fails in some larger sets of numbers, such as the rational numbers and a set of algebraic integers, where the number 9 can be factored two ways: 9=3*3=(2+sqrt{-5})(2-sqrt{-5}).
We say that an integer d divides n if n=dk for some integer k. A integer p greater than 1 is prime if p=ab implies either p=a or p=b. We prove that this is equivalent to the more classic "grade school definition" of p having only two (positive) divisors. We show that divisibility is transitive, and how this means that every positive integer is divisible by a prime. We state, without proof, the fundamental theorem of arithmetic, which says that every integer greater than 1 has a unique prime factorization. Though this seems obvious, we concluding by showing how this actually fails in some larger sets of numbers, such as the rational numbers and a set of algebraic integers, where the number 9 can be factored two ways: 9=3*3=(2+sqrt{-5})(2-sqrt{-5}).
Discrete Mathematical Structures, Lecture 4.1: Binary relations on a set
Truth Table Tutorial - Discrete Mathematics Logic
Hasse Diagram with Example (Discrete Mathematics) Order relation & Lattice
Relations & Functions | 3rd Sem | CSE | Module-3 | Discrete Mathematical Structures | Session-4
Lecture 4 || Truth Table || Construction of Truth Table ||Discrete Mathematics
Lattice
Introduction to Function and Types of Function - Functions - Discrete Mathematics
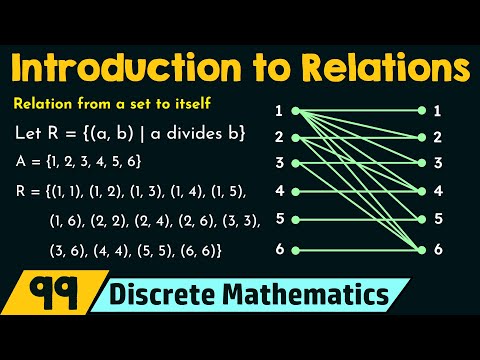
Introduction to Relations
Learn Relation & Function (Basic to Advanced level) Lecture-2| JEE(Main/Advance)/Class XII Topic
INTRODUCTION to SET THEORY - DISCRETE MATHEMATICS
RELATIONS - DISCRETE MATHEMATICS
Discrete Mathematics (Full Course)
Mathematical Induction Problem 1 - Logic - Discrete Mathematics
Types of Relations (Part 1)
Discrete Mathematical Structures, Lecture 1.1: Basic set theory
Discrete Mathematics Lecture 2 | Principle of Mathematical Induction By Dr.Gajendra Purohit
What is Discrete Mathematics?
Introduction to Discrete Mathematics
Equivalence Relation
Discrete Mathematics for Computer Science
Algebraic Structure|Group Theory|MODULO| Discrete Mathematics|Lecture 04| All University
Venn Diagram Problem 2 and 3 - Set Theory - Discrete Mathematics
Equivalence Classes
truth values and tables in fuzzy logic | truth values and truth tables | class 12 in hindi |shortcut
Комментарии
 0:41:30
0:41:30
 0:07:51
0:07:51
 0:02:42
0:02:42
 0:26:10
0:26:10
 0:11:38
0:11:38
 0:15:20
0:15:20
 0:10:55
0:10:55
 0:07:39
0:07:39
 1:02:11
1:02:11
 0:16:38
0:16:38
 0:15:36
0:15:36
 6:08:01
6:08:01
 0:13:30
0:13:30
 0:06:39
0:06:39
 1:00:20
1:00:20
 0:19:34
0:19:34
 0:02:30
0:02:30
 0:09:37
0:09:37
 0:06:29
0:06:29
 0:03:15
0:03:15
 0:29:49
0:29:49
 0:06:46
0:06:46
 0:07:19
0:07:19
 0:03:31
0:03:31