filmov
tv
Trigonometric substitution with sine (KristaKingMath)
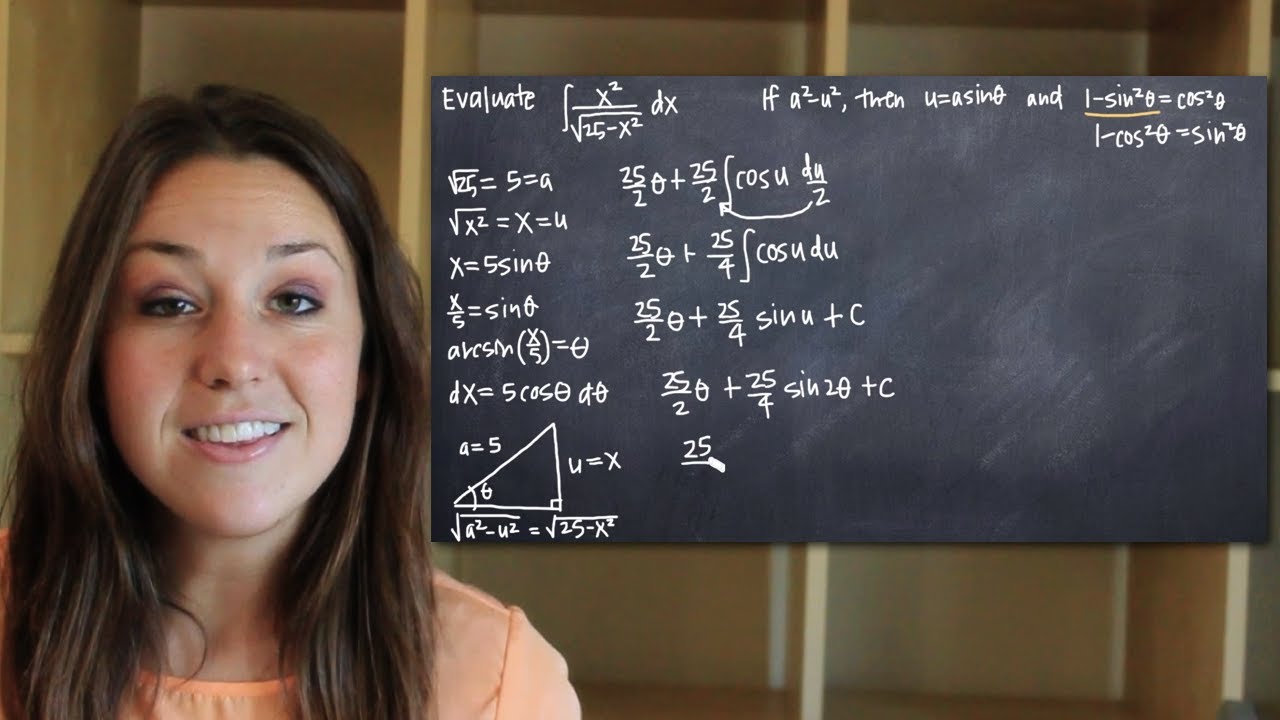
Показать описание
Learn how to use trigonometric substitution with a sine substitution to evaluate an integral.
● ● ● GET EXTRA HELP ● ● ●
● ● ● CONNECT WITH KRISTA ● ● ●
Hi, I’m Krista! I make math courses to keep you from banging your head against the wall. ;)
Math class was always so frustrating for me. I’d go to a class, spend hours on homework, and three days later have an “Ah-ha!” moment about how the problems worked that could have slashed my homework time in half. I’d think, “WHY didn’t my teacher just tell me this in the first place?!”
Trigonometric substitution with sine (KristaKingMath)
❖ Trigonometric Substitution with Sine and U-Substitution: A Step-by-Step Example ❖
Trigonometric Substitution Example 7 (KristaKingMath)
How to solve EVERY trigonometric substitution problem ever!
Trigonometric Substitution Example 4 (KristaKingMath)
Trigonometric Substitution Example 2 (KristaKingMath)
Trigonometric Substitution Example 6 (KristaKingMath)
Integrating a trig function using u-substitution!
Trigonometric Substitution Example 5 (KristaKingMath)
Trigonometric Substitution Example 3 (KristaKingMath)
Trig substitution - How to solve?
Trigonometric Substitution Integral (Trig Sub Integral Example)
Trigonometric substitution with tangent (KristaKingMath)
Integration By Trigonometric Substitution
Integrals of trigonometric functions, sin(mx)sin(nx) (KristaKingMath)
Trigonometric Substitution - Sine
Trig Substitution (Part 1: Using Sin)
Integration - HOW TO: Trigonometric Substitution (Beginner Level, Part 3)
Trigonometric substitution with secant (KristaKingMath)
Integrals of trigonometric functions, tan^msec^n, even n (KristaKingMath)
Trig substitution integration: x=a*sinθ, calculus 2
Trigonometric integrals - sin^mcos^n, odd n (KristaKingMath)
Integration By Trig Substitution (Sine Example 3)
Integration By Trig Substitution (Sine Example 2)
Комментарии
 0:19:01
0:19:01
 0:07:42
0:07:42
 0:14:23
0:14:23
 0:13:13
0:13:13
 0:09:40
0:09:40
 0:07:47
0:07:47
 0:10:47
0:10:47
 0:03:36
0:03:36
 0:08:17
0:08:17
 0:09:52
0:09:52
 0:39:24
0:39:24
 0:08:54
0:08:54
 0:17:29
0:17:29
 0:15:55
0:15:55
 0:04:30
0:04:30
 0:09:06
0:09:06
 0:06:03
0:06:03
 0:14:14
0:14:14
 0:13:55
0:13:55
 0:05:47
0:05:47
 0:24:04
0:24:04
 0:11:20
0:11:20
 0:06:54
0:06:54
 0:07:31
0:07:31