filmov
tv
Bessel's Equation - Ordinary Differential Equations | Lecture 29

Показать описание
In this lecture we summarize the Frobenius method in complete generality. We further discuss what happens when the indicial equation has repeated roots, which leads to a significantly more complex form for the second fundamental solution to the ODE. We then apply these methods to Bessel's equation, a famous second-order ODE that arises in many areas of applied mathematics. With Bessel's equation we demonstrate the complexity of identifying series solutions when there are repeated roots of the indicial equation.
* In this video we focus on the Bessel equation of 0th order, meaning that the parameter alpha is taken to be 0. You can work with other values of alpha and follow similarly to arrive at the solutions there too.
Two of my recent papers that use the Bessel equation and the functions that solve it (all using the standard notation of J_nu(x) ):
This course is taught by Jason Bramburger for Concordia University.
Follow @jbramburger7 on Twitter for updates.
* In this video we focus on the Bessel equation of 0th order, meaning that the parameter alpha is taken to be 0. You can work with other values of alpha and follow similarly to arrive at the solutions there too.
Two of my recent papers that use the Bessel equation and the functions that solve it (all using the standard notation of J_nu(x) ):
This course is taught by Jason Bramburger for Concordia University.
Follow @jbramburger7 on Twitter for updates.
Bessel's Equation - Ordinary Differential Equations | Lecture 29
Solution of Bessel's Equation | Ordinary Differential Equation | MSc Mathematics
Bessel Functions and the Frobenius Method
Solving the Bessel Equation (for general order of nu) using the Frobenius Method
Bessel Differential Equations: Simple solution of an example
Lecture 2020 10 14 Bessel's Equation and Function
Ordinary Differential Equations 06 Series Solutions : Bessel's Equation
Bessel's Equation - (Differential Equation)
Bessel Function x^2y''+xy'+(9x^2-4)y=0. Bessel Function of the Second kind.
Solving Bessel's differential equation using Frobenius Method part 1 of 2
Bessel Functions
Bessel's Equation | Numericals | Bessel's Differential Equation | Series solution of ODEs...
Addition formula for Bessel Function | Ordinary differential equation | MSc Mathematics
Solution of Bessel's differential equation leading to Bessel functions by easy maths easy trick...
Transform Equation to Bessel
Bessel's Integral formula | Ordinary differential equation | MSc Mathematics
Bessel function #differentialequationsbsc2ndyear #maths
Introduction to Bessel Function and Formula Discussion - Bessel Function - Engineering Mathematics 3
Properties of zeroes of Bessel's function | Ordinary differential equation | MSc Mathematics
Bessel and legendre function #maths #differentialequations
Bessel function
Bessel Function x^2y''+xy'+(x^2-1/9)y=0. Bessel Function of the first kind
Bessel's Differential Equation: Solution and it's Properties
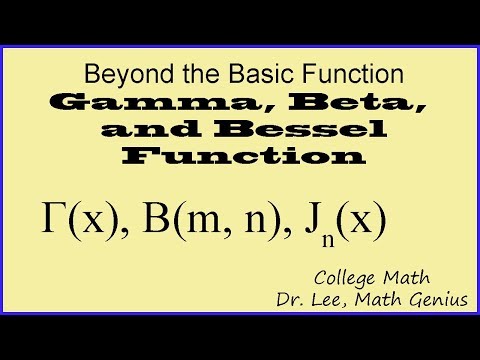
Gamma, Beta, and Bessel function. Solving differential equation (4)
Комментарии
 0:28:41
0:28:41
 0:36:39
0:36:39
 0:14:33
0:14:33
 0:32:56
0:32:56
 0:23:42
0:23:42
 0:45:54
0:45:54
 0:28:12
0:28:12
 0:04:50
0:04:50
 0:10:32
0:10:32
 0:13:53
0:13:53
 0:07:07
0:07:07
 0:05:31
0:05:31
 0:22:53
0:22:53
 0:18:19
0:18:19
 0:15:58
0:15:58
 0:11:50
0:11:50
 0:00:10
0:00:10
 0:10:04
0:10:04
 0:28:32
0:28:32
 0:00:11
0:00:11
 0:00:08
0:00:08
 0:05:49
0:05:49
 0:42:32
0:42:32
 0:08:01
0:08:01