filmov
tv
Solving Quadratic Congruences with Prime Modulus

Показать описание
Solving quadratic congruences with prime modulus using factoring and completing the square.
Solving Quadratic Congruences with Prime Modulus
How to solve a quadratic congruence when the modulus is NOT prime
How to solve a Quadratic Congruence modulo prime
Solving a Mod Equation with Prime Powers
What is a Quadratic Residue? - Definition and Example
How to solve a non-factorable quadratic congruence
How to solve quadratic congruence equation when modulo is not prime? #SoME2
Number Theory | Solving Quadratic Congruences with Hensel's Lemma
Number Theory | Quadratic Residues: Definition and Examples
Quadratic Congruence Composite Composite Modulus
Number Theory | A quadratic formula mod p??
Quadratic Residue (Part-1) Solution of Quadratic Congruence
How to solve Quadratic Congruence Equation
Quadratic Congruence with Composite Modulus 93
Solving standard quadratic congruence part- 7
Quadratic Congruence with Composite Modulus
QUADRATIC CONGRUENCE EXAMPLES | PROBLEMS #1
Solve a Quadratic Congruence Equation
Solve a Quadratic Congruence
Find least positive solution to the quadratic congruence (4x^2 + 7x +1)==0 (mod 11)
Solving congruences, 3 introductory examples
Number Theory | Solving Polynomial Congruences with Hensel's Lemma
Quadratic Residue (Part 13) Quadratic Congruence with Composite Moduli
Solve the Quadratic Congruence with Chinese Remainder Theorem
Комментарии
 0:13:41
0:13:41
 0:11:56
0:11:56
 0:08:14
0:08:14
 0:09:57
0:09:57
 0:04:53
0:04:53
 0:10:23
0:10:23
 0:03:16
0:03:16
 0:13:53
0:13:53
 0:04:44
0:04:44
 0:21:30
0:21:30
 0:07:18
0:07:18
 0:11:32
0:11:32
 0:03:16
0:03:16
 0:21:02
0:21:02
 0:09:49
0:09:49
 0:12:46
0:12:46
 0:04:39
0:04:39
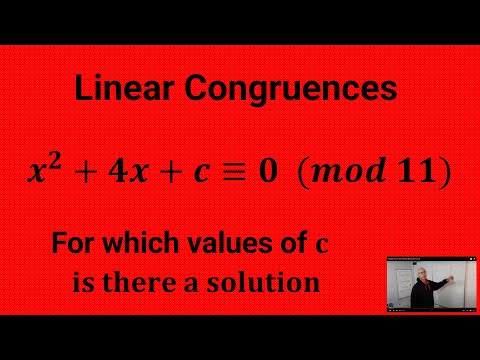 0:13:33
0:13:33
 0:06:32
0:06:32
 0:11:18
0:11:18
 0:03:51
0:03:51
 0:04:12
0:04:12
 0:24:44
0:24:44
 0:16:32
0:16:32