filmov
tv
If xyz=1, then prove that (1+x+y^-1)^-1 + (1+y+z^-1)^-1 + (1+z+y^-1)^-1 = 1.
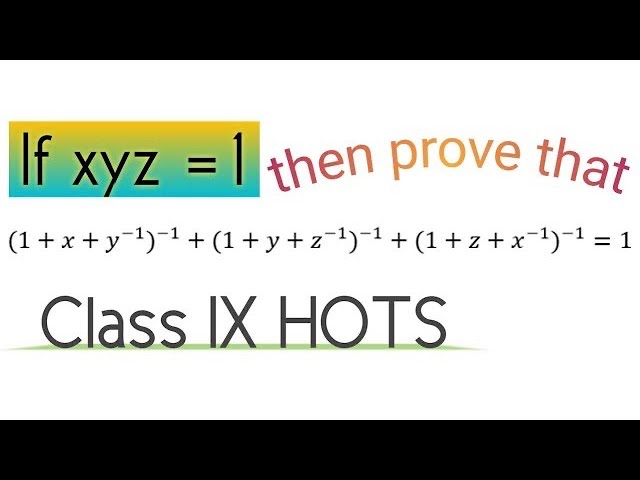
Показать описание
HOTS level question from Number System (Laws of Exponents) Class IX
0:09:41
If xyz=1, then prove that (1+x+y^-1)^-1 + (1+y+z^-1)^-1 + (1+z+y^-1)^-1 = 1.
0:01:47
If a^x=b,b^y=c & c^z=a, prove that xyz=1.| indices | class10
0:07:04
If xyz=1, then simplify (1+x+y^-1)^-1 + (1+y+z^-1)^-1 + (1+z+x^-1)^-1 #polynomial #class9
0:10:58
If xyz+1=0 then prove that 1/1-Z-X^-1 + 1/1-X-Y^-1 + 1/1-Y-Z^-1 =1 (CLASS 9/10 IMPORTANT QUESTION)
0:05:04
11. If xyz=1 then show that` (1+ x+ y^-1)^-1+ (1+y +z^-1)^-1+ (1+ z+x^-1)^- 1=1`
0:04:10
If xyz = 1, prove that: 1/(1 + x + y^-1) + 1/(1 + y + z^-1) + 1/(1 + z + x^-1) = 1
0:05:28
If XYZ = 1 then prove that (1+x+y^1) ^-1 + (1+y+z^1) ^-1 + (1+z+x^1) ^-1 = 1
0:02:26
If logx/(y-z) = logy/(z-x) = logz/(x-y) then prove that xyz = 1 | IITJEE Foundation Maths
0:03:43
If a = b^x, b = c^y, c = a^z then prove that xyz = 1
0:06:05
Indices ll SEE Question 2070 D ll IR Simkhada
0:02:50
If xyz=1 prove that (1+x+y^-1) ^-1+ (1+y+z^-1) ^-1+ (1+z+x^-1)^-1=1
0:02:38
If a = b^x , b = c^y , c = a^z then prove that xyz = 1 . | Algebra - Class 10 | Indices | C.Math |
0:02:56
If x^a=y , y^b =z , z^c=x, then prove that abc =1 | chapter 1 Number system class 9
0:16:10
If xyz+1=0 then prove that 1/1-Z-X^-1 + 1/1-X-Y^-1 + 1/1-Y-Z^-1 =1 (CLASS 9/10 IMPORTANT QUESTION)
0:05:39
xyz=1, find the value of 1/(1+x+1/y)+1/(1+y+1/z)+1/(1+z+1/x)
0:00:48
Prove That XYZ = 1 #Shorts
0:00:58
if a^x=b; b^y=c; c^z=a then prove that xyz=1
0:02:22
If `a^(x)=b, b^(y)=c,c^(z)=a,` prove that xyz = 1 where a,b,c are distinct numbers
0:07:19
If `x, y, z` are all different real numbers, then prove that `1/(x-y)^2+1/(y-z)^2+1/(z-x)^2=...
0:02:42
Prove that: xyz + 1 = 2yz. If x = log_(2a)(a), y = log_(3a)(2a) and z = log_(4a)(3a) || Log Function
0:03:29
If a^x=b^y=c^z and b²=ac then prove that y=2xz/x+z
0:20:03
Class 10 indices || indices || if abc=-1, prove that:1-a-b^-1 || if abc=1, prove that : 1÷1+a+b^-1
0:03:22
If U = e^xyz Prove that Ә^3u/ ӘxӘyӘz = (1+3xyz+x^2 y^2 z^2)e^xyz Partial Differentiation
0:03:00
If xyz=1,prove that (x+1/x)^2+(y+1/y)^2+(z+1/z)^2=4+(x+1/x)(y+1/y)(z+1/z).
Комментарии