filmov
tv
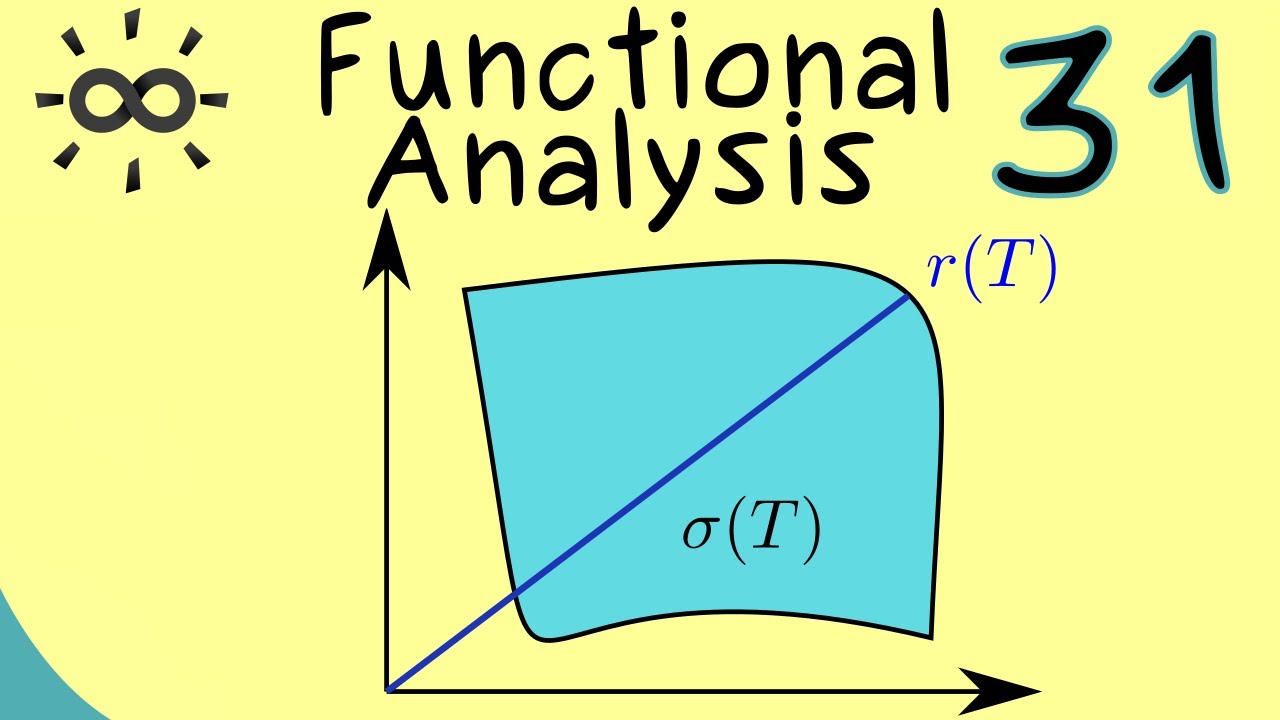
Functional Analysis 31 | Spectral Radius
Показать описание
Please consider to support me if this video was helpful such that I can continue to produce them :)
🙏 Thanks to all supporters! They are mentioned in the credits of the video :)
This is my video series about Functional Analysis where we start with metric spaces, talk about operators and spectral theory, and end with the famous Spectral Theorem. I hope that it will help everyone who wants to learn about it.
x
#FunctionalAnalysis
#VectorSpaces
#Mathematics
#LearnMath
#calculus
I hope that this helps students, pupils and others. Have fun!
(This explanation fits to lectures for students in their first and second year of study: Mathematics for physicists, Mathematics for the natural science, Mathematics for engineers and so on)
Functional Analysis 31 | Spectral Radius
Functional Analysis 30 | Properties of the Spectrum
Functional Analysis 34 | Spectral Theorem for Compact Operators [dark version]
Functional Analysis 31
Functional Analysis 33 | Spectrum of Compact Operators
Functional Analysis 28 | Spectrum of Bounded Operators
Functional Analysis 32 | Normal and Self-Adjoint Operators
#80: Defining spectral family associated with T
Glass: Back to basics (31) - Spectral energy distribution as a function of surface temperature
Approx&Stab - 5. Spectra and their approximation (C5-06): the spectral radius II
Functional Analysis 1 | Metric Space - How to Measure Distances?
Functional Analysis 19 | Hölder's Inequality
Functional Analysis 22 | Dual Spaces
Trying transition video for the first time 💙😂 || #transformation #transition #shorts #viralvideo...
Mathematics: How can point spectrum equal residual spectrum?
Functional Analysis 1 | Metric Space - How to Measure Distances? [dark version]
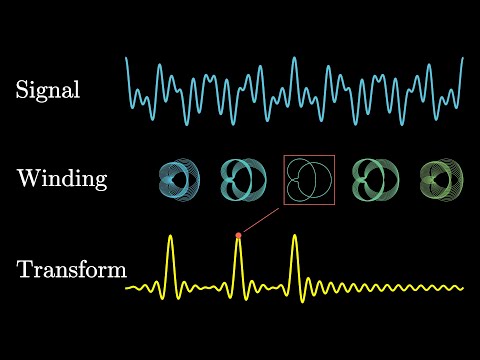
But what is the Fourier Transform? A visual introduction.
Lec 31 Heteronuclear spectral analysis
What is...the spectral theorem?
Doctorate program: Functional Analysis - Lecture 31: The closed graph theorem.
Spectral Theory - Module II - 7 .2. 3 Lemma (Domain of R_{\lambda})
Proof explanation: Spectral radius of operators
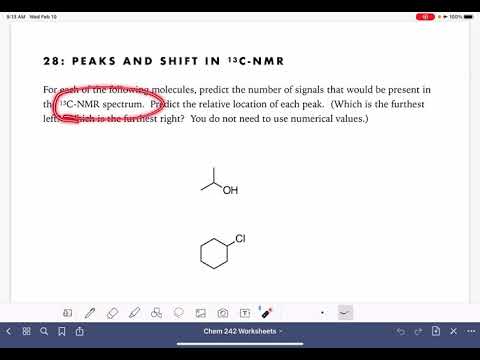
31a: Predicting C-NMR spectra from molecular structure
Пирковский А. Ю. Functional Analysis 2 (Operator Theory) 18.05.2021 ауд. 427
Комментарии
 0:11:12
0:11:12
 0:10:27
0:10:27
 0:09:21
0:09:21
 0:35:27
0:35:27
 0:11:03
0:11:03
 0:07:45
0:07:45
 0:07:19
0:07:19
 0:09:01
0:09:01
 0:02:12
0:02:12
 0:13:32
0:13:32
 0:05:59
0:05:59
 0:07:42
0:07:42
 0:09:17
0:09:17
 0:00:15
0:00:15
 0:01:35
0:01:35
 0:05:43
0:05:43
 0:20:57
0:20:57
 0:29:19
0:29:19
 0:11:49
0:11:49
 0:40:00
0:40:00
 0:09:01
0:09:01
 0:02:44
0:02:44
 0:03:17
0:03:17
 1:24:08
1:24:08