filmov
tv
A Nice Rational Puzzle | Can You Solve This Algebraic Equation?
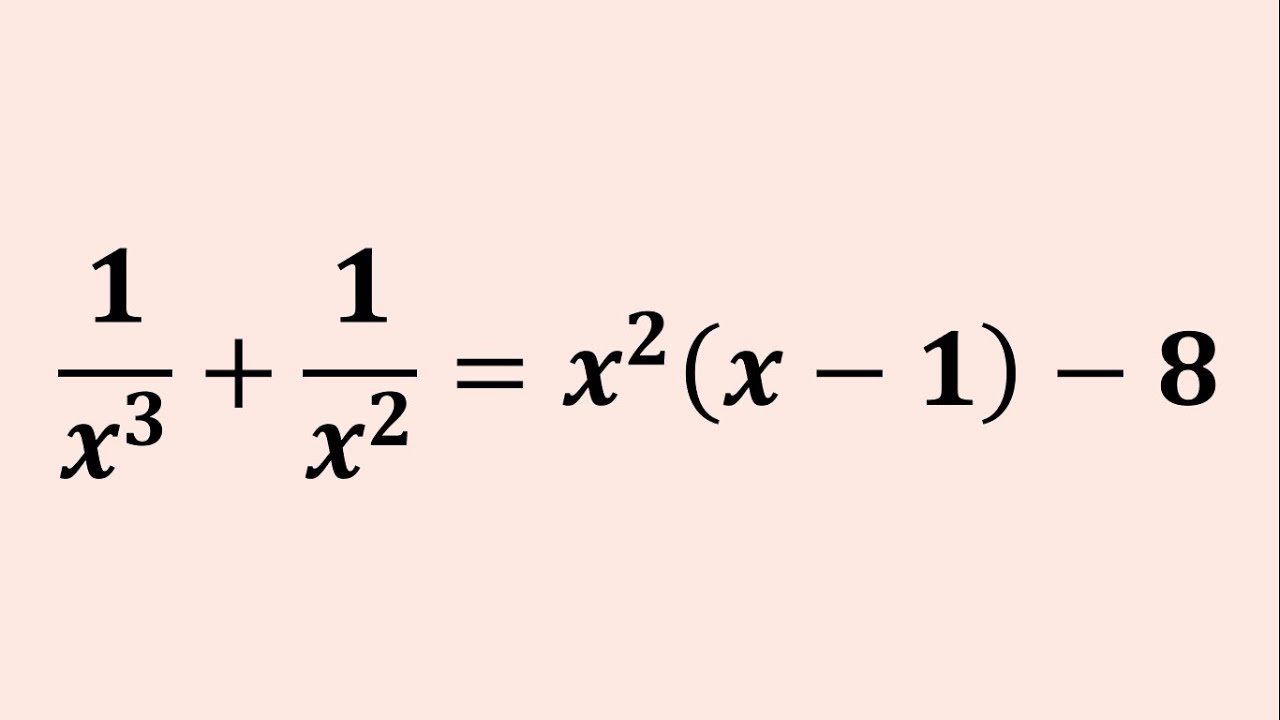
Показать описание
A Nice Rational Puzzle | Can You Solve This Algebraic Equation?
Welcome to another algebraic equation challenge! In this video, we tackle a nice rational equation using the powerful method of substitution. This problem is perfect for sharpening your algebra skills and preparing for competitive math exams.
Watch as we break down the steps to solve this equation, providing clear explanations and tips along the way. Whether you're a math enthusiast or a student aiming to excel in Olympiad competitions, this video will help you understand and master rational equations.
Don't forget to like, comment, and subscribe for more math challenges and solutions. Happy solving!
In this tutorial, you'll learn:
1- Fundamental concepts and definitions of rational equations
2- Step-by-step method of substitutions to solve rational equation
3- Common pitfalls and how to avoid them
4- Expert tips and tricks for solving problems quickly and accurately
5- Practice problems with detailed solutions
Additional Resources:
#matholympiad #rationalequations #mathtutorial #substitution #math #mathskills #learnmath #education #algebra
Join us as we unlock the secrets to excelling in rational equations and take your Math Olympiad prep to the next level. Don't forget to like, subscribe, and hit the bell icon for more Math Olympiad prep videos. Let's conquer those equations together!
Thanks for Watching!!
Welcome to another algebraic equation challenge! In this video, we tackle a nice rational equation using the powerful method of substitution. This problem is perfect for sharpening your algebra skills and preparing for competitive math exams.
Watch as we break down the steps to solve this equation, providing clear explanations and tips along the way. Whether you're a math enthusiast or a student aiming to excel in Olympiad competitions, this video will help you understand and master rational equations.
Don't forget to like, comment, and subscribe for more math challenges and solutions. Happy solving!
In this tutorial, you'll learn:
1- Fundamental concepts and definitions of rational equations
2- Step-by-step method of substitutions to solve rational equation
3- Common pitfalls and how to avoid them
4- Expert tips and tricks for solving problems quickly and accurately
5- Practice problems with detailed solutions
Additional Resources:
#matholympiad #rationalequations #mathtutorial #substitution #math #mathskills #learnmath #education #algebra
Join us as we unlock the secrets to excelling in rational equations and take your Math Olympiad prep to the next level. Don't forget to like, subscribe, and hit the bell icon for more Math Olympiad prep videos. Let's conquer those equations together!
Thanks for Watching!!
Комментарии
 0:09:18
0:09:18
 0:04:42
0:04:42
 0:04:39
0:04:39
 0:03:17
0:03:17
 0:11:41
0:11:41
 0:16:15
0:16:15
 0:00:40
0:00:40
 0:06:51
0:06:51
 0:00:16
0:00:16
 0:06:41
0:06:41
 0:01:44
0:01:44
 0:09:03
0:09:03
 0:07:50
0:07:50
 0:00:05
0:00:05
 0:00:42
0:00:42
 0:11:18
0:11:18
 0:09:57
0:09:57
 0:00:59
0:00:59
 0:08:03
0:08:03
 0:03:01
0:03:01
 0:00:13
0:00:13
 0:03:25
0:03:25
 0:09:04
0:09:04
 0:00:46
0:00:46