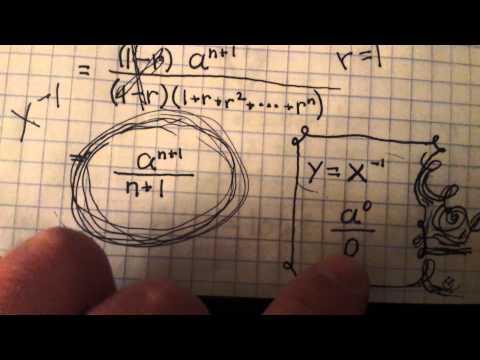
Fermat's unique method of integration.

🌟Support the channel🌟

🌟my other channels🌟

🌟My Links🌟

🌟How I make Thumbnails🌟

🌟Suggest a problem🌟

[xizarrg]

History of math, both of things the people did, as well as the math they developed, is always an interesting dive.

[TheLowstef]

For the second integral, you wrote it wrong in the beginning. Should've been 1/x^n and you just put x^n

[andrewdjang]

With regards to the n=1 case for negative exponents, I would love to see your take on historical methods for finding the “quadrature of the hyperbola”!

[ardan]

In the vein of integration before calculus, pascal's method of integrating the sine and cosine is fascinating and requires only geometry

[goodplacetostop]

13:56 Oh so that time, the margin was large enough for that proof?

[ericfielding]

Fun to watch - to get an inkling of what Fermat thought - and entirely sensible

[bjornfeuerbacher]

This looks like the reverse operation to the q(uantum) derivative?

[WTzReAdY]

5:43 this actually corresponds to the LIMIT of the sum of the geometrical series that otherwise would be 1-r^n instead of 1 in the numerator. This is not really mentionned and it is not entirely rigourus if I am understanding well... I guess we would retrieve the same expression in the end but I think it is worth mentionning.

[ianfowler]

So damn cool!! I first encountered this as an undergraduate in a history of mathematics course (back in the 70's). Just as remarkable (a nod to Fermat) is his method of finding tangents to polynomials as well as Descarte's method for finding tangents - double roots. Worthy of a video? All pre-Newton. Bloody marvelous. Long live infinite geometric series.

[siquod]

Isn't there a negative sign missing in the second integral result?

[salcanoman]

A question: In the second part we are working on (1/x^n), so why on the right part of the blackboard the integral is written for x^n ? Thanks.

[get]

Very interesting. Nicely illustrates that the limit has to be the same regardless of the sequence of simple functions.

[ianfowler]

Did you also know that any infinite series that you can prove converges by the ratio test intuitively tells you something very remarkable. Such a series behaves more and more and more like an infinite geometric series the further and further you go out in the series. The ratio |a(n+1) / a(n)| as n --> inf. approaches the magic value of |r| < 1 for an infinite geometric series. Long live convergent infinite geometric series.

[hugh]

You could also just take the limit of (1-q)/(1-q^(n+1)) using L'Hopital's Rule to get 1/(n+1) without bothering with the factorisation. Not sure that's any simpler though, both quite simple.

[topquark]

Can a similar approach be used on functions other than x^n? In this case a sequence of partition points X_n = (a, q a, q^2 a, ... ) is used to generate the rectangles. For other functions f(x) you would have to use a different sequence of partition points. So can this method be generalized?

[Cor]

There is another approach of this integral which makes use of the notion that the integral of x^n from a to b equals the area under the curve. If it is area, it must satify the basic properties of area (linearity, additivity, scale invariance, translation invariance and normalization of area, ie the integral of 1 from 0 to 1 must be 1. When these conditions are met, we will find the integral from 0 to a of x^n equals 1/(n+1) a^(n+1). One can proof this without any limiting proces, just finite calculations, for all rational numbers.

[mikek]

Good stuff! Can you make a video about Donald Knuth’s approach, where he uses Bachmann/Landau notation to define derivatives? Thanks!

[tomkerruish]

Is this the inspiration for q-analogs?

[bentationfunkiloglio]

This was so interesting and intuitive.

[TheEternalVortex]

Really cool that Fermat used this method, I suppose it was based on Archmedes 'method of exhaustion'? He probably also computed the lower bound of inner rectangles to ensure they got the same value.

So the general formula would be that ∫ f(t) dt = lim_{q->1} a (1 - q) (f(a) + f(aq) q + f(a q^2) q^2 + .... )
I wonder if there's any integrals that this makes easier than the "standard" Riemann sum. It seems like the extra q factors make it not work out nicely unless f power function.

For example, if we want to integrate e^t we get a (1 - q)(e^a + q e^(aq) + q^2 e^(aq^2) + ... ) and I'm not sure that you can do much with that