filmov
tv
Relativistic Momentum and Common Sense - Why Physics Theories are Counterintuitive
Показать описание
Momentum in Classical Mechanics looks different to Momentum in Special Relativity. But why is that?
Hey everyone, I'm back with another video! This time we're using Relativistic Momentum, and Classical Momentum, as examples to talk about why certain tricky to understand theories (e.g. Relativity and Quantum Mechanics) happen to be the best descriptors we have right now of the universe.
Firstly, let's start with the basic idea that classical physics is generally considered "intuitive" to most people. The objects described by this branch of physics end up behaving like our day-to-day experiences, and our expectations, tell us they should. But classical physics famously breaks down when considering objects moving at large velocities. Anything that moves at a considerable fraction of the speed of light needs a relativistic description. And special relativity really is mind-bending, introducing ideas that don't make intuitive sense to most people, such as the constancy of the speed of light, time dilation, length contraction, etc.
But here's the thing: before the advent of relativity, humans were not used to very fast-moving objects. So we did not have a good grasp of how objects should behave at those speeds, or even the fact that the speed of these objects made any difference.
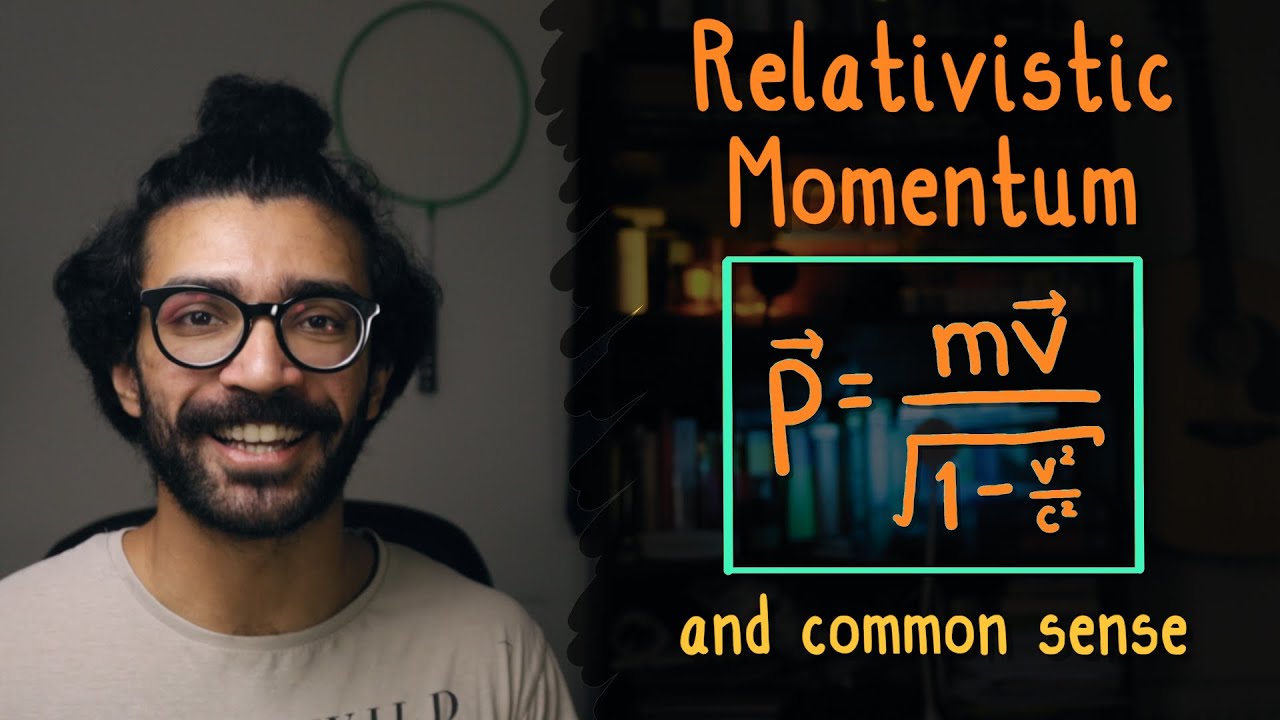
Interestingly, when we consider the expression for (special) relativistic momentum, we know that it's a good descriptor of the motion of objects moving at all speeds. And when we consider the motion of objects at speeds that we are used to in day-to-day life, we find that the special relativistic momentum expression actually reduces to the classical expression. In other words, at low velocities, classical and relativistic momentum looks the same! This is why classical physics derived the equation that p = mv (momentum = mass x velocity), rather than p = mv/sqrt(1-(v/c)^2), where c is the speed of light, taken to be a constant in relativity.
This kind of logic is used as a little test for many complicated theories by the way. If any theory is a complicated and counterintuitive one, we can ask the question. "does our theory reduce down to classical physics under the right conditions?". This is because in these specific conditions, classical physics is indeed a good description of the universe. So our weird new theory agreeing with this is only a good thing. If the two theories disagree, then this is not a huge problem - we need to try and work out why. When we apply this logic to quantum physics, a principle known as the Correspondence Principle pops up. In the limit of large quantum numbers, quantum systems must look like classical systems. In simplified terms, quantum mechanics describes very very small systems excellently. And this behaviour, when extended to much larger systems, must agree with the behaviour that we happen to observe - distinctly classical behaviour, which is why we came up with classical mechanics in the first place.
I also wanted to use a nice little analogy to represent our understanding of the universe from a classical perspective, and how subsequent mind-bending. "weird" theories encompassed classical physics and yet described even more of the universe. So I put in some trains!
Follow me on Instagram @parthvlogs. I'll see you soon!
Hey everyone, I'm back with another video! This time we're using Relativistic Momentum, and Classical Momentum, as examples to talk about why certain tricky to understand theories (e.g. Relativity and Quantum Mechanics) happen to be the best descriptors we have right now of the universe.
Firstly, let's start with the basic idea that classical physics is generally considered "intuitive" to most people. The objects described by this branch of physics end up behaving like our day-to-day experiences, and our expectations, tell us they should. But classical physics famously breaks down when considering objects moving at large velocities. Anything that moves at a considerable fraction of the speed of light needs a relativistic description. And special relativity really is mind-bending, introducing ideas that don't make intuitive sense to most people, such as the constancy of the speed of light, time dilation, length contraction, etc.
But here's the thing: before the advent of relativity, humans were not used to very fast-moving objects. So we did not have a good grasp of how objects should behave at those speeds, or even the fact that the speed of these objects made any difference.
Interestingly, when we consider the expression for (special) relativistic momentum, we know that it's a good descriptor of the motion of objects moving at all speeds. And when we consider the motion of objects at speeds that we are used to in day-to-day life, we find that the special relativistic momentum expression actually reduces to the classical expression. In other words, at low velocities, classical and relativistic momentum looks the same! This is why classical physics derived the equation that p = mv (momentum = mass x velocity), rather than p = mv/sqrt(1-(v/c)^2), where c is the speed of light, taken to be a constant in relativity.
This kind of logic is used as a little test for many complicated theories by the way. If any theory is a complicated and counterintuitive one, we can ask the question. "does our theory reduce down to classical physics under the right conditions?". This is because in these specific conditions, classical physics is indeed a good description of the universe. So our weird new theory agreeing with this is only a good thing. If the two theories disagree, then this is not a huge problem - we need to try and work out why. When we apply this logic to quantum physics, a principle known as the Correspondence Principle pops up. In the limit of large quantum numbers, quantum systems must look like classical systems. In simplified terms, quantum mechanics describes very very small systems excellently. And this behaviour, when extended to much larger systems, must agree with the behaviour that we happen to observe - distinctly classical behaviour, which is why we came up with classical mechanics in the first place.
I also wanted to use a nice little analogy to represent our understanding of the universe from a classical perspective, and how subsequent mind-bending. "weird" theories encompassed classical physics and yet described even more of the universe. So I put in some trains!
Follow me on Instagram @parthvlogs. I'll see you soon!
0:11:43
Relativistic Momentum and Common Sense - Why Physics Theories are Counterintuitive
0:15:23
70 | Relativity and common sense
0:09:25
The ultimate speed = c! Relativistic momentum and energy (VCE / A-level / IB physics)
0:44:13
Lecture 30 — Relativistic Momentum, Relative Velocity, and Energy
0:30:41
Relativistic Momentum: UNIZOR.COM - Relativity 4 All - Conservation
0:38:31
Relativistic Momentum and Energy and Relative Velocity in Special Relativity — Part 1
0:03:25
Classroom Aid - Relativistic Momentum
0:39:06
Relativistic Energy and Momentum: Explained
0:08:42
Relativistic Momentum | Physics with Professor Matt Anderson | M29-06
0:43:54
Relativistic Momentum
0:26:32
Relativistic momentum and energy relationships
0:05:21
Relativistic Momentum
0:12:29
PHYS 230 Chapter 5 Sec 8 - Relativistic Momentum
0:03:06
Momentum in Special Relativity | Four-Momentum
0:07:11
Relativity and Common Sense
0:05:41
Relativity 09.09. Relativistic Momentum
0:11:21
The Mass Shell (Relativistic Energy-Momentum-Mass Relation)
0:53:06
Introduction to Relativistic Momentum
0:10:32
Intro to Modern Physics: Relativistic Momentum
11:29:00
WSU: Special Relativity with Brian Greene
0:48:23
Modern Physics, Lecture 12: Relativistic Kinematics. Energy and Momentum.
1:12:59
general physics II, lecture 24 relativity and nature of energy and momentum
0:06:34
Physics 62 Special Relativity (12 of 43) Momentum of a Photon
0:32:46
Relativistic Momentum and Energy and Relative Velocity in Special Relativity — Part 2
Комментарии