How to Calculate Standard Deviation by Hand

How to calculate standard deviation by hand?

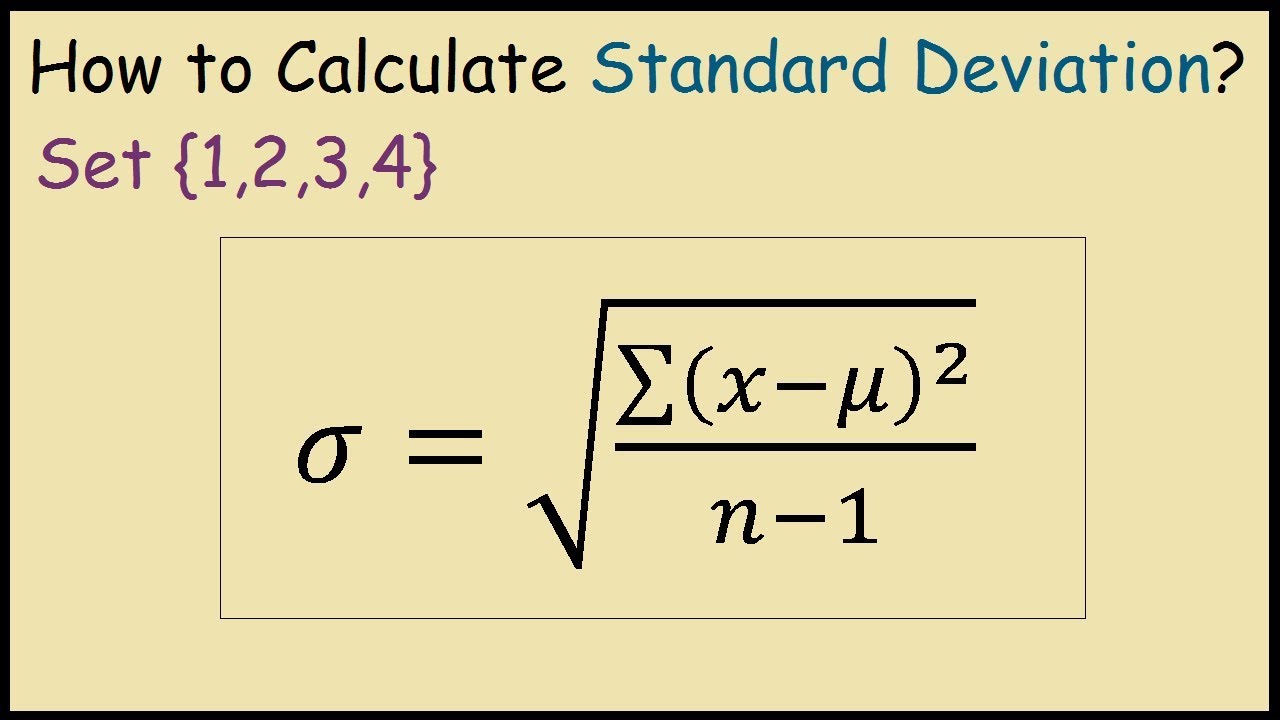
The first step is to calculate the average of the set of data values which is usually denoted by the symbol mu. The second step is to calculate (x - average)² for every data value where x is each data point. Lastly to work out n, count up how many data values there are in the data set.

Depending on how the mean is calculated the formula for standard deviation will differ, if the mean is calculated from the data set then the formula will have a n-1, if the mean is given in the question then the formula will only be divided by n.

Standard deviation is a measure of how spread out data values are from the mean, a low standard deviation indicates that the data is close to the mean while a high standard deviation number indicates that the data is spread out over a wide range of values.

Standard deviation mostly appears in the mathematical branch called statistics and is used in bell curves to plot a normal distribution. Often you will see the symbol sigma (σ) used to denote standard deviation.

After normalizing data it is expected that 68% of the data is within 1 standard deviation, 95% of the data is within 2 standard deviations and 99.7% of the data is within 3 standard deviations.

Music by Adrian von Ziegler

[nataliacampos]

Hell yeah, super easy to follow, thank you!