filmov
tv
Integral of ∫cot^2(x)dx
Показать описание
In this video, we find the integral or anti-derivative of cot^2(x). We approach this by first revising the derivative of cot(x), which equals -csc^2(x).
Then, by the Pythagorean Trigonometric identity: cot^2(x) + 1 = csc^2(x). We can rearrange this equation to:
cot^2(x) = csc^2(x) - 1
Realising that -csc^2(x) derivative of cot(x), we can write the above as:
cot^2(x) = -d/dx[cot(x)] - 1
Thanks for watching. Please give me a "thumbs up" if you have found this video helpful.
Please ask me a maths question by commenting below and I will try to help you in future videos.
Then, by the Pythagorean Trigonometric identity: cot^2(x) + 1 = csc^2(x). We can rearrange this equation to:
cot^2(x) = csc^2(x) - 1
Realising that -csc^2(x) derivative of cot(x), we can write the above as:
cot^2(x) = -d/dx[cot(x)] - 1
Thanks for watching. Please give me a "thumbs up" if you have found this video helpful.
Please ask me a maths question by commenting below and I will try to help you in future videos.
0:01:24
Integral of cot^2(x), calculus 2 tutorial
Integral of ∫cot^2(x)dx
0:09:19
integral cotx dx integral cot^2x dx integral cot^3x dx
0:02:26
Class 12th – Integral of cot x dx | Integrals | Tutorials Point
0:01:16
Integration of cot^2x dx
0:02:45
Evaluate the Integral sqrt(cot x) csc^2 x dx using the substitution rule
0:05:22
Integral of cot square x | Integration of cot square x into dx | Integration of cot^2x |
0:03:46
Integral of cot(x)csc^2 (x) dx (Integration by Parts)
0:03:51
L(integration 0 to t Sinx/xdx) =1/sCot^-1s || Integral transform Msc 3rd Semester mathematics ||
0:02:16
Integral of cot^5x, cot(x) and csc(x) approach, calculus 2 tutorial
0:02:33
176. Integral de cotangente al cuadrado. TRIGONOMETRICA. EJERCICIO RESUELTO.
0:03:36
Evaluate the following integrals: `intx^(2)cot^(-1)xdx`
0:01:23
integral of cot^2(x) dx
0:03:03
`int(cos8x-1)/(tan2x-cot2x)dx`
0:01:28
`int(tan^(2)x-cot^(2)x)dx=`
0:08:37
integration of cot^2x cosecx dx
0:01:55
Integral of cot^3(x), calculus 2 tutorial
0:02:01
`inte^(x)(1-cotx+cot^(2)x)dx=`
0:01:36
integral cot(2x) csc(2x) dx , cosecante por cotangente
0:03:22
integral of cot^3 x csc^2 xdx
0:05:56
Reduction Formula for Integral of ∫cot^n(x)dx
0:02:19
`int (x+ cot x)cot^(2) x dx`
0:03:43
integral por cambio de variable - integral de xcot^2(2x^2)dx
0:01:29
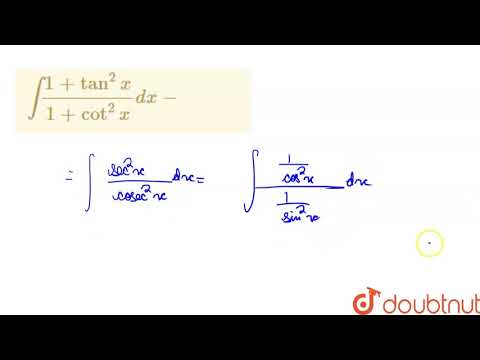
`int(1+tan^(2)x)/(1+cot^(2)x)dx-`
Комментарии