11-Topic: 7.2(i) Prove that l=rθ.

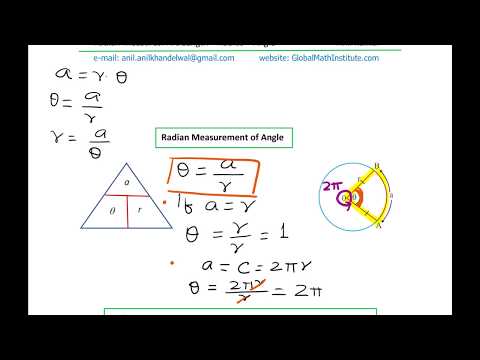
Topic: 7.2(i) To establish a rule l=rθ, where r is the radius of the circle,
l the length of a circular arc and θ the central angle measured in radius.

Radian measure of an angle (Circular System)
One radian is an angle subtended at the center of a circle by an arc whose length is equal to the radius of the circle.
#l=rθ.
#Sector
Measurement of angle in the sexagesimal system ( degree, minutes seconds)
Why a circle is divided into 360 degrees? Brief History
Trigonometry: Trigonometry is a branch of mathematics in which we study the length of sides and angles of a triangle and their relationship.

Angle: An angle is defined as the union of two rays with some common endpoint.
These rays are called arms of the angle and the common endpoint is called the vertex.

Angle in standard position: An angle is said to be in standard position if its initial
side is on the positive x-axis and the vertex is at the origin.

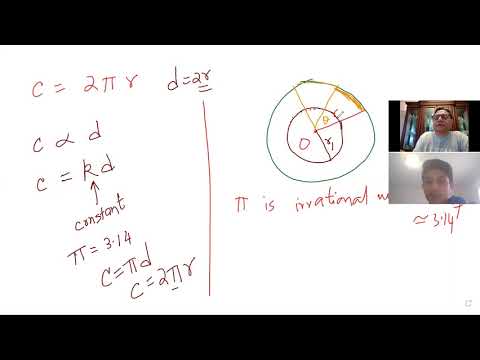
Degree: If the circumference of a circle is divided into 360 equal arcs then each arc
subtends the angle of 1 degree at the Centre of the circle.

[pragyayadavxls]

Thank you sir 🙏😊
And sir if we want to measure radius of moon through this formula then ( by assumption ) then r will be radius of earth and l will be distance from earth to moon ?
Am i right ...in phy

[keeplearningforeverfromany]

Dhanywad sir.. Sir Aap Pakistan se ho?