filmov
tv
Fractional Part Function | Floors & Fractional Parts

Показать описание
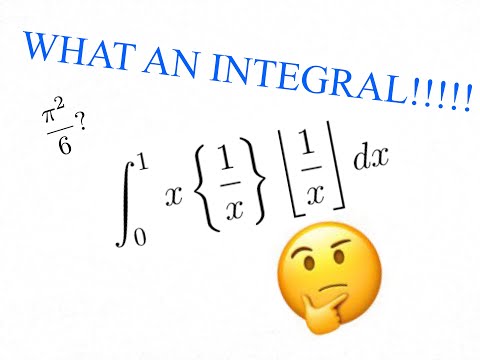
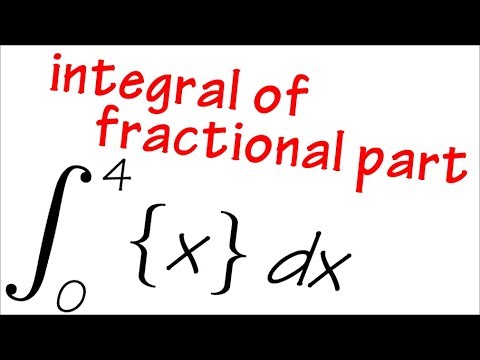
We present techniques for dealing with problems involving the floor and the fractional part function, focusing on the fractional part function. The techniques are varied and apply to many different problems including quadratic equations in the fractional part and floor function, equations that sums involving the fractional part function, and even integrals.
The key idea is to represent things in terms of integers.
#FractionalPartFunction #FloorFunction #OlympiadProblems
GET MY BOOK ON AMAZON!!
========================
"Number Theory Towards RSA Cryptography in 10 Undergraduate Lectures"
CHECK OUT OTHER TYPES OF VIDEOS:
================================
Improve Your Putnam Math Competition Performance:
------------------------------------------------------
Math Theorems:
------------------------------------------------------
GRE Math Subject Test:
------------------------------------------------------
Road to RSA Encryption:
-------------------------------------------------------
CHECK ME OUT ON THE INTERNET!!
==============================
Twitter: @mohamedomarphd
Instagram: profomarmath
And of course, subscribe to my channel!
The key idea is to represent things in terms of integers.
#FractionalPartFunction #FloorFunction #OlympiadProblems
GET MY BOOK ON AMAZON!!
========================
"Number Theory Towards RSA Cryptography in 10 Undergraduate Lectures"
CHECK OUT OTHER TYPES OF VIDEOS:
================================
Improve Your Putnam Math Competition Performance:
------------------------------------------------------
Math Theorems:
------------------------------------------------------
GRE Math Subject Test:
------------------------------------------------------
Road to RSA Encryption:
-------------------------------------------------------
CHECK ME OUT ON THE INTERNET!!
==============================
Twitter: @mohamedomarphd
Instagram: profomarmath
And of course, subscribe to my channel!
Комментарии
 0:21:36
0:21:36
 0:05:55
0:05:55
 0:09:35
0:09:35
 0:08:02
0:08:02
 0:10:39
0:10:39
 0:11:55
0:11:55
 0:06:09
0:06:09
 0:00:57
0:00:57
 0:00:16
0:00:16
 0:07:36
0:07:36
 0:03:14
0:03:14
 0:08:24
0:08:24
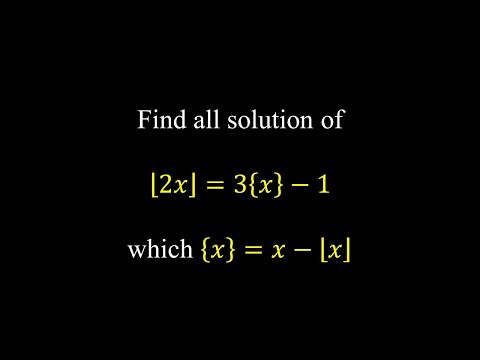 0:05:27
0:05:27
 0:06:41
0:06:41
 0:00:42
0:00:42
 0:07:10
0:07:10
 0:11:12
0:11:12
 0:06:26
0:06:26
 0:00:16
0:00:16
 0:03:20
0:03:20
 0:19:55
0:19:55
 0:00:58
0:00:58
 0:08:17
0:08:17
 0:27:21
0:27:21