Intersections and Composites of Galois Extensions are Galois (Algebra 3: Lecture 23 Video 3)

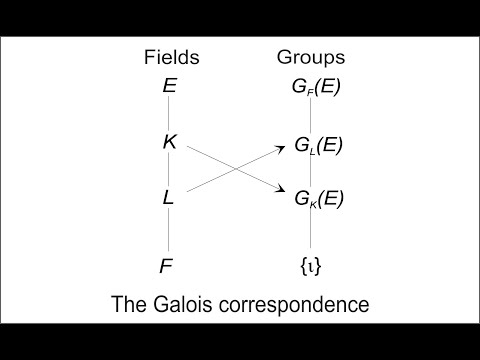
Lecture 23:  We started this lecture by discussing several Algebra Qualifying Exam problems that involve computing the splitting field of a polynomial over a finite field.  We proved a general result about the degree of the splitting field of x^n-1 over Fq[x].  We then started to discuss composites of field extensions.  We proved that if K/F is a Galois extension and F'/F is any extension, then KF'/F' is a Galois extension and its Galois group is isomorphic to the Galois group of K over K intersect F’.  As a consequence we saw a nice formula for the degree of KF' over F.  We saw an example that showed that it really is necessary for at least one of these two extensions over F to be Galois.  At the end of the lecture wee proved that intersections and composites of Galois extensions are Galois.