filmov
tv
Restriction of eigenfunctions to sparse sets on manifolds

Показать описание
Restriction of eigenfunctions to sparse sets on manifolds (videoconference)
Milabika Pramanik, University of British Columbia
August 28 2020 / 28 août 2020
Abstract:
Given a compact Riemannian manifold $(M, g)$ without boundary, we consider the restriction of Laplace-Beltrami eigenfunctions to certain subsets $Gamma$ of the manifold. How do the Lebesgue $L^p$ norms of these restricted eigenfunctions grow? Burq, Gerard, Szvetkov and independently Hu studied this question when $Gamma$ is a submanifold. In ongoing joint work with Suresh Eswarathasan, we extend earlier results to the setting where $Gamma$ is an arbitrary Borel subset of $M$. Here differential geometric methods no longer apply. Using methods from geometric measure theory, we obtain sharp growth estimates for the restricted eigenfunctions that rely only on the size of $Gamma$. Our results are sharp for large $p$, and are realized for large families of sets $Gamma$ that are random and Cantor-like.
Milabika Pramanik, University of British Columbia
August 28 2020 / 28 août 2020
Abstract:
Given a compact Riemannian manifold $(M, g)$ without boundary, we consider the restriction of Laplace-Beltrami eigenfunctions to certain subsets $Gamma$ of the manifold. How do the Lebesgue $L^p$ norms of these restricted eigenfunctions grow? Burq, Gerard, Szvetkov and independently Hu studied this question when $Gamma$ is a submanifold. In ongoing joint work with Suresh Eswarathasan, we extend earlier results to the setting where $Gamma$ is an arbitrary Borel subset of $M$. Here differential geometric methods no longer apply. Using methods from geometric measure theory, we obtain sharp growth estimates for the restricted eigenfunctions that rely only on the size of $Gamma$. Our results are sharp for large $p$, and are realized for large families of sets $Gamma$ that are random and Cantor-like.
 1:13:47
1:13:47
 1:11:41
1:11:41
 0:48:58
0:48:58
 1:01:06
1:01:06
 0:50:59
0:50:59
 1:52:20
1:52:20
 1:06:07
1:06:07
 1:13:09
1:13:09
 0:42:10
0:42:10
 0:34:59
0:34:59
 0:48:50
0:48:50
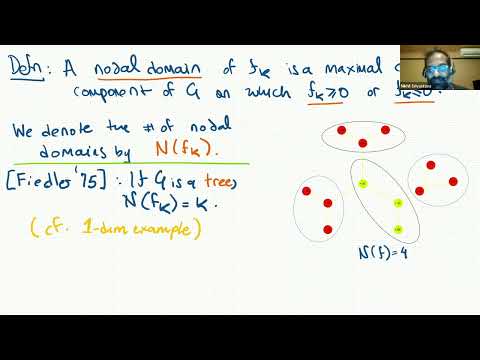 1:12:12
1:12:12
 1:22:00
1:22:00
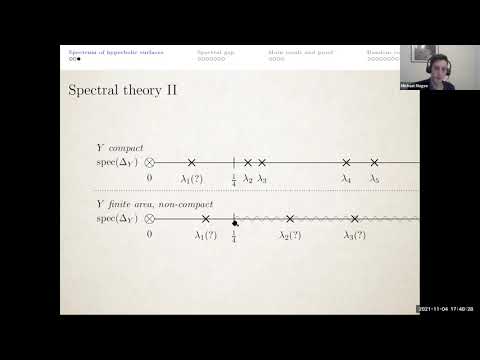 1:05:42
1:05:42
 0:53:21
0:53:21
 0:31:09
0:31:09
 0:51:49
0:51:49
 1:00:15
1:00:15
 0:45:50
0:45:50
 0:51:29
0:51:29
 1:06:57
1:06:57
 0:16:31
0:16:31
 1:03:47
1:03:47
 0:57:02
0:57:02