filmov
tv
Linear Programming 27: Optimality is no harder than feasibility

Показать описание
Linear Programming 27: Optimality is no harder than feasibility
Abstract: We give a third explanation for why finding an optimal solutions is no harder than finding a feasible solution, using the duality of linear programming.
This video accompanies the class "Linear Programming and Network Flows" at Colorado State University
We are following the book "Understanding and Using Linear Programming" by Jirí Matoušek and Bernd Gärtner
Our course notes are available at
Abstract: We give a third explanation for why finding an optimal solutions is no harder than finding a feasible solution, using the duality of linear programming.
This video accompanies the class "Linear Programming and Network Flows" at Colorado State University
We are following the book "Understanding and Using Linear Programming" by Jirí Matoušek and Bernd Gärtner
Our course notes are available at
 0:12:09
0:12:09
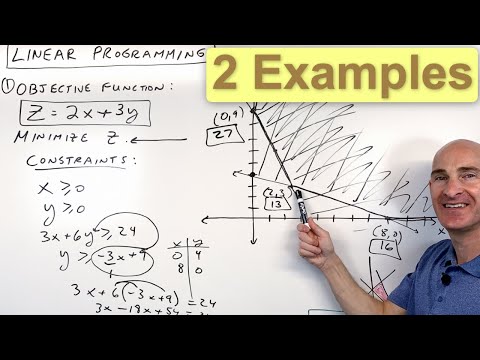 0:15:08
0:15:08
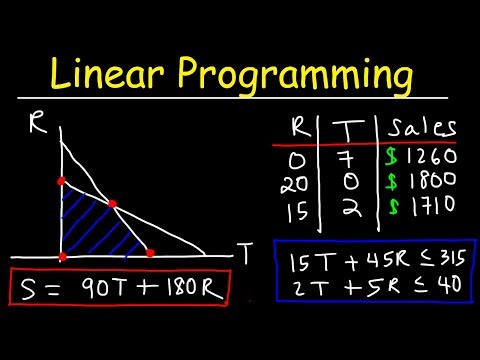 0:33:20
0:33:20
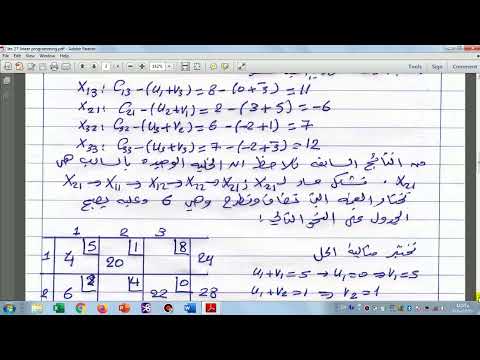 0:01:29
0:01:29
 0:05:27
0:05:27
 1:07:16
1:07:16
 0:09:03
0:09:03
 0:19:10
0:19:10
 0:41:41
0:41:41
 0:12:36
0:12:36
 0:30:27
0:30:27
 0:07:58
0:07:58
 0:14:27
0:14:27
 0:23:31
0:23:31
 0:09:16
0:09:16
 0:00:16
0:00:16
 0:06:44
0:06:44
 0:48:25
0:48:25
 0:01:51
0:01:51
 0:12:46
0:12:46
 1:00:52
1:00:52
 1:22:27
1:22:27
 1:27:13
1:27:13
 0:51:10
0:51:10