filmov
tv
Limits | Continuity of Inverse Functions and Intermediate Value Theorem and Finding Root | 8 |

Показать описание
In this video we will explain 2 concepts:
1. Continuity of an Inverse function with application
2.The intermediate value theorem
f k is a value between f(a) and f(b), i.e. either f(a) is smaller than k is smaller than f(b) or f(a) is larger than k is larger than f(b)
then there exists at least a number c within a to b i.e. c ∈ (a, b) in such a way that f(c) = k
This has an important consequence in finding root of a function.
If f is a function which is continuous at every point of the interval [a, b] and f(a) is smaller than 0, f (b) is larger than 0 then f (x) = 0 at some point x ∈ (a, b).
Lectures on Continuity
Limits | Introduction to the Concept of Limits | An Intuitive Approach | 1 |
Limits | One Sided Limits | 2 |
Limits | Infinite Limits and Vertical Asymptotes | 3 |
Limits | limits at Infinity and Horizontal Asymptotes |4 |
Limits | Concept of Continuity |5|
Limits | Continuous Functions on Open and Closed Intervals | 6 |
Limits | Continuity of Polynomials, Rationals and Composition | 7 |
This is useful for those who are preparing
1. Basics of MAEconomics entrance exam
2. BA Economics
3. BA Mathematics
4. Basic Calculus
1. Continuity of an Inverse function with application
2.The intermediate value theorem
f k is a value between f(a) and f(b), i.e. either f(a) is smaller than k is smaller than f(b) or f(a) is larger than k is larger than f(b)
then there exists at least a number c within a to b i.e. c ∈ (a, b) in such a way that f(c) = k
This has an important consequence in finding root of a function.
If f is a function which is continuous at every point of the interval [a, b] and f(a) is smaller than 0, f (b) is larger than 0 then f (x) = 0 at some point x ∈ (a, b).
Lectures on Continuity
Limits | Introduction to the Concept of Limits | An Intuitive Approach | 1 |
Limits | One Sided Limits | 2 |
Limits | Infinite Limits and Vertical Asymptotes | 3 |
Limits | limits at Infinity and Horizontal Asymptotes |4 |
Limits | Concept of Continuity |5|
Limits | Continuous Functions on Open and Closed Intervals | 6 |
Limits | Continuity of Polynomials, Rationals and Composition | 7 |
This is useful for those who are preparing
1. Basics of MAEconomics entrance exam
2. BA Economics
3. BA Mathematics
4. Basic Calculus
 0:13:17
0:13:17
 0:11:05
0:11:05
 0:22:07
0:22:07
 0:07:53
0:07:53
 0:07:52
0:07:52
 0:05:30
0:05:30
 0:06:35
0:06:35
 0:10:06
0:10:06
 0:06:06
0:06:06
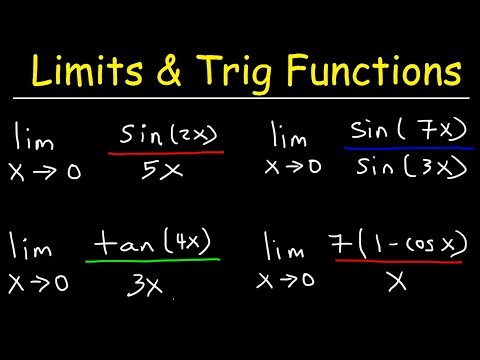 0:15:23
0:15:23
 0:02:55
0:02:55
 0:08:47
0:08:47
 0:20:44
0:20:44
 0:24:34
0:24:34
 0:00:43
0:00:43
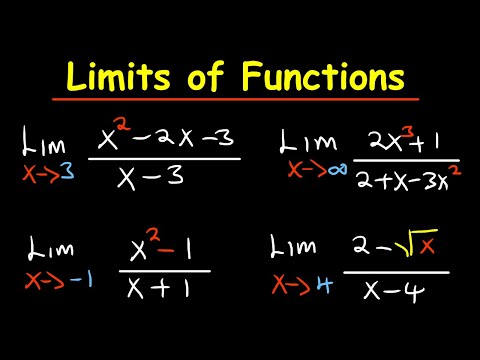 0:15:09
0:15:09
 0:07:06
0:07:06
 0:16:54
0:16:54
 0:19:19
0:19:19
 0:23:39
0:23:39
 0:19:24
0:19:24
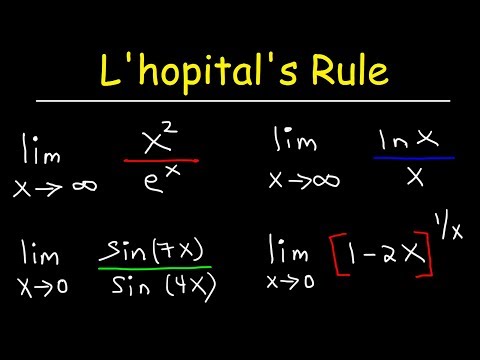 0:13:09
0:13:09
 0:16:20
0:16:20
 0:10:07
0:10:07