A-Level Pure Maths Chapter 3: Equations & Inequalities *PAST EXAM QUESTION*

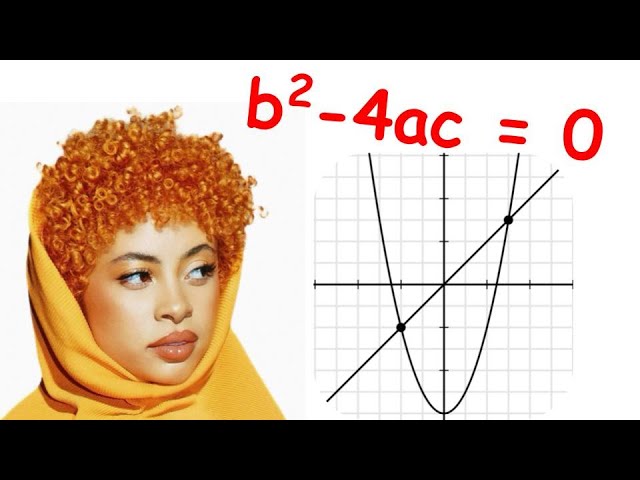
In this AS and A-Level Maths Equations and Inequalities tutorial, we delve into a tricky past exam question that requires us to solve a pair of quadratic/linear simultaneous equations. We want use the discriminant to show that a quadratic equation in the form x^2 + 8kx + k = 0 must be satisfied, for some non-zero value of k. Then in part two of the video we solve to find k, using the method of completing the square. There is a really nice trick, meaning we only get one solution- even though quadratic simultaneous equations normally yield two solutions! Our mission is then to go back to our original simultaneous equations with our nrewly-discovered value of k, and solve them to find a single pair of solutions for x and y. Join us as we break down the problem step by step and unlock the mathematical beauty behind it.

📌 Key Points Covered:

Solving quadratic simultaneous equations
Discriminant of a quadratic equation
Completing the square

[mancubsofficial]

Fantastic video once again! I managed to complete part A by myself, but wasn't sure how to start B as I hadn't heard of the term "equal roots" before. However, after you explained it, I realised that it's the same thing as a repeated root, and I was able to complete that part of the question on my own, and got the right answer for B! However, I hadn't thought of completing the square for the next part, so I tried factorising (and failed miserably) but once I saw you do it, it made more sense. This is a really nice problem with quadratic simultaneous equations! :)

[bababoeye]

Sir, I do not appreciate being click baited . I saw ice spice and did not see her in the video :(

[Bonita]

Sir, stop using our form room please :]