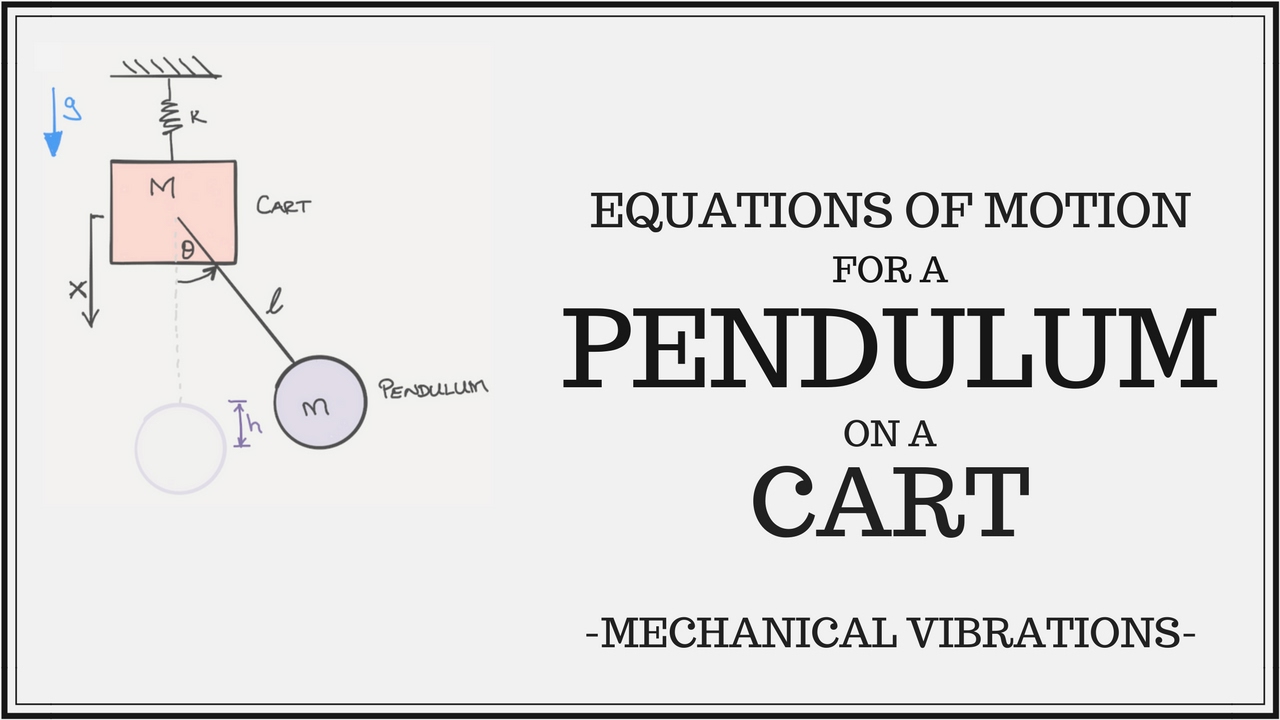
Equations of Motion for a Pendulum on a Cart (2DOF) Using Method of Lagrange's Equations

Deriving the equations of motion for a pendulum attached to a cart using the method of Lagrange's Equations. Two degree of freedom system.

[deorum]

Everybody can be a hero without wearing a cape.You are one of them thank you so much.

[CyberFraming]

truly a hero for all of us. thank you very much for making an elegant and understandable video

[lucasdure]

best video of mechanical vibration that i've ever seen

[Zxymr]

Thanks a lot for this lecture; the explanation was very clear and I'm planning to run a numerical simulation.
Just out of curiosity, you mentioned in 0:30 that the weight of the system can be ignored, even though the displacement x is in the same axis as gravity. Does this mean that x=0 is not the resting displacement when the spring is unloaded, but when it is loaded by the system?

[atreysolanki]

@Freeball  Sir but X is about/from the equilibrium position so if we are taking that position as our reference point then (M+m)*g*x should be considered in the potential energy... because X will be below the reference point.because X is the additional displacement from the equilibrium position

[leonardoparra]

Been watching your videos for sometime now to go back to the basics continuously... Do you have recommended books you use for conceptual references?


I want to dive into more examples/problems. Derivation for me is usually to understand all the influencing parameters, and quantify what I already have speculated, but i don't like getting bogged down in them for way too long.


When I fix a frame on a garage door, I don't go about deriving how to add adjustments to bolster rigidity. I just drill some wooden rods with the right fasteners in the right places if you know what I mean.


Anyways, I've been trying to combine different concepts for applications in aerospace & structural design.


Current books/material, are less textbooky (which are awesome btw & i love penguin classics) :


-The New Science of Strong Materials, J.E. Gordon
- Structures : Or Why Things Don't Fall Down, J.E. Gordon
- Success through Failure: The Paradox of Design, Henry Petroski

[diegohcsantos]

So it's, somehow, implied that the spring has a "infinity stiffness" in the horizontal?

[wenvpi]

why you didnt use the gravitational potencial of the mass M for the expresion V?

[hug_me_plz]

thank for your video. I totally understand these system by lagragian. theta is the changing value by time. It helps me a lot

[rafaelcostelo]

how does the potential energy equation differ if there was another spring connected underneath the cart to another datum point?

[azmyin]

Sir given these two equation can we now use small angle approximations to do modal analysis and find natural frequencies?

[kennethgoodall]

How would this be written in matrix form, or is it impossible to do so as it is everything's far to coupled

[ibrahimalkhazali]

Thanks a lot, plz what app you use for writing

[eigenme]

hey I wonder if you can do a brief video on tuned mass dampers, where the spring and mass are moving horizontally, and the pendulum attached below, thanks a lot!

[hakunamatata]

Sir, hi. I have a question. If we take the datum the very top of the sistem h becomes as h = -x-l*cos(theta) which changes the equation. What am I doing wrong?

[sulamitsihombing]

sir, i want to ask something. this is just a dummy question, but i really don't get about the potential energy. i've always learned that we need a fulcrum to decide the position of thing. i don't get why you wrote the position of the pendulum as l(1-cos theta) and not x+lcos theta just like the position of the pendulum (xm). thank you

[artsyengineer]

Hi! Thanks for the nice video! I was wondering how you would go about solving this problem assuming small angles?

[bavrined]

Hello. How can you define the function of angle in such problems? I mean, what's the function of angle, derivative of which we take in order to find general 'dot'-equations of the coordinates?

[ayoube_baalla]

Thanks a lot sir
I need the solution of this equations

[karansaini]

How we can Linearized both equation of motion ?