Rigid Body Kinematics Introduction | Rotation Matrix Relating Frames in 3D | Direction Cosine Matrix

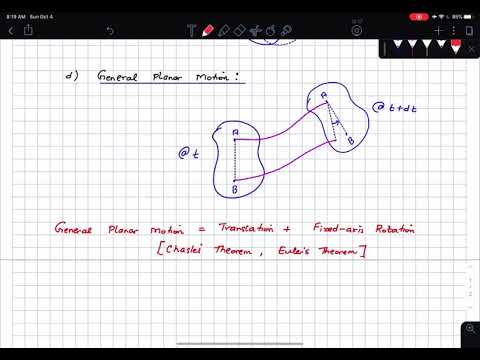
Space Vehicle Dynamics 🛰 Lecture 12: Rigid body kinematics. Rotation matrices. Direction cosine matrix. To describe the orientation or attitude of a rigid body compared to a reference frame, we need to first consider a frame attached to the rigid body, then go about describing how the body-fixed frame is related to the inertial frame. Basic concepts first in 2D, then in 3D. The direction cosine matrix [C], sometimes abbreviated as the DCM, relates the frames (the triad of unit vectors) but also vectors in each frame. We discuss the direction cosine matrix on its own, before we discuss how to describe it in terms of 3 Euler angles in a later lecture, for example, yaw, pitch, and roll.

► Next: Euler Angles for Aerospace | Yaw, Pitch, Roll

► Previous, Rigid System of Particles | From Discrete Particles to the Continuous Limit of an Extended Mass Distribution

► More lectures posted regularly

► Dr. Shane Ross, aerospace engineering professor, Virginia Tech
Background: Caltech PhD | worked at NASA/JPL & Boeing
Research website for @ProfessorRoss

► Follow me on Twitter

► Lecture notes (PDF)

► All course videos (playlist)

► Reference:
Schaub & Junkins, Analytical Mechanics of Space Systems, 4th edition, 2018

- Typical reference frames in spacecraft dynamics
- Mission analysis basics: satellite geometry
- Kinematics of a single particle: rotating reference frames, transport theorem
- Dynamics of a single particle
- Multiparticle systems: kinematics and dynamics, definition of center of mass (c.o.m.)
- Multiparticle systems: motion decomposed into translational motion of c.o.m. and motion relative to the c.o.m.
- Multiparticle systems: imposing rigidity implies only motion relative to c.o.m. is rotation
- Rigid body: continuous mass systems and mass moments (total mass, c.o.m., moment of inertia tensor/matrix)
- Rigid body kinematics in 3D (rotation matrix and Euler angles)
- Rigid body dynamics; Newton's law for the translational motion and Euler’s rigid-body equations for the rotational motion
- Solving the Euler rotational differential equations of motion analytically in special cases
- Constants of motion: quantities conserved during motion, e.g., energy, momentum
- Visualization of a system’s motion
- Solving for motion computationally

#RigidBodyKinematics #RotationMatrix #Rotation #RigidBody #Kinematics #DirectionCosineMatrix #Rigidity #InertiaMatrix #MomentOfInertia #InertiaTensor #EngineeringDynamics #SystemOfParticles #ConservationOfEnergy #WorkedProblem #WorkedExample #Dumbbell #CenterOfMass #MatlabTutorial #Tutorial #Lecture #Matlab #NewtonsLaws #ConservationOfMomentum #SolutionStrategy #ProblemSolving #ProblemSolvingStrategy #TransportTheorem #RotatingFrame #InertialFrame #VectorDerivative #SatelliteGeometry #RotatingFrames #SpacecraftDynamics #SpaceVehicle #AttitudeDynamics #SpaceVehicleDynamics #RigidBodyDynamics #dynamics #LawsOfMotion #engineering #aerospace #ElonMusk #spacetravel #SpaceX #Boeing #Satellite #Satellites #SpaceDomainAwareness #NewtonsLaw #NewtonsLawofMotion #EquationOfMotion #Newtons2ndLaw #NewtonianMechanics #AOE3144 #Caltech #NASA #VirginiaTech #engineering #dynamics #mechanics #physics #mathematics #science #aerospace #mechanicalengineering #spacecraft #openaccess #OnlineCourse #technology #robotics #space #spaceindustry #math #biomechanics #vehicledynamics #simulation #aerodynamics #innovation #NewtonEuler #SingleDegreeofFreedom #LinearMotion #NonlinearDynamics #DynamicalSystems #AppliedMath #ChaosTheory #Bifurcation #DifferentialEquations #mathematics #Newton #math #FreeCourses #OnlineCourse #Lagrangianpoints #Lyapunov #VectorField #GraphicalMethod #FixedPoints #EquilibriumPoints #NonlinearODEs #StablePoint #UnstablePoint #Stability #LinearStability #StabilityAnalysis #VectorField #Pendulum #Poincare​ #mathematicians #maths #mathstudents #mathematician #mathfacts #mathskills #mathtricks

[jiyanbaranbukun]

Thanks a lot Professor, very clear and intuitive explanation!

[sahhaf]

Thank you very much, Dr. Ross. This was an excellent presentation.

[tabhashim]

At 12:56 Shouldn’t the parameterized C matrix switch the negative on the sin. Because looking at it from a transformation point of view, the n1 vector will have to shrink in the x direction, and go up in the y direction. But based off of this matrix, it would go down. I guess what I am trying to say is that this is for a CW not CCW rotation which is what is shown

[julianer]

Hey Dr.Shane, Thanks a lot for the video! But I have one problem, I feel like my basic knowledge of vectors is hindering me to understand kinematics. How far do I have to relearn vectors so I can understand kinematics? Can you give me any advice or help? Thanks in advance 👍 I’m in my first year at uni studying mechanical engineering and I‘m lost atm.

[markengel]

Pray tell:
Which ‘book” do you refer to?

[FLMKane]

So um...my prof back in the day was very adamant that frames ate attached to a body, points are NOT.
What's your opinion about that?

[TriThom]

Why is that not a vector? A vector could consist of multiple other vectors?

[bidhayakgoswami]

9.38 min: You could have written [b1 b2]=[ n1 n2] [ C ] where n1, n2, b1, b2 are column vectors (basis vectors) and C is the matrix of cosines, . 'Vectrix' is neither a good nor a correct way to write or teach. Any vector (say v) with representation u=[ u1 u2] in frame 2 will become C.u in frame 1. As u and Cu are of same length, C'C=I. Therefore, C is in SO(3).
It has an eigenvalue equal to 1 (an invariant direction, axis of rotation in this case) and a pair of complex conjugate eigenvalues (invariant subspace, plane perpendicular to axis of rotation).