Ex-2A // Q.n 19-20 /Infinite series // Sequence and their limits //

Показать описание

sequence and their limit definition of sequence and their limit bounded sequence range of sequence phenite and infinite sequence convergence sequence definition of limit the limit of a second when it exist is unique coaches definition of limit of convergence couch is General principle of convergence every conversion sequence is bounded oscillatory sequence divergent sequence divergent sequence theorem unbounded bounded diabetes conversation limit theorem for the limit theorem further limit theorem fundamental theorems on limit combination property of limit combination property of modulus Theorem excuse principle excuse principle squeeze principal squeeze theorem some useful limits application of ratio test resut test ratio test monotonic conversion sequence convergent theorem monotonic sequence monotonic conversion sequence
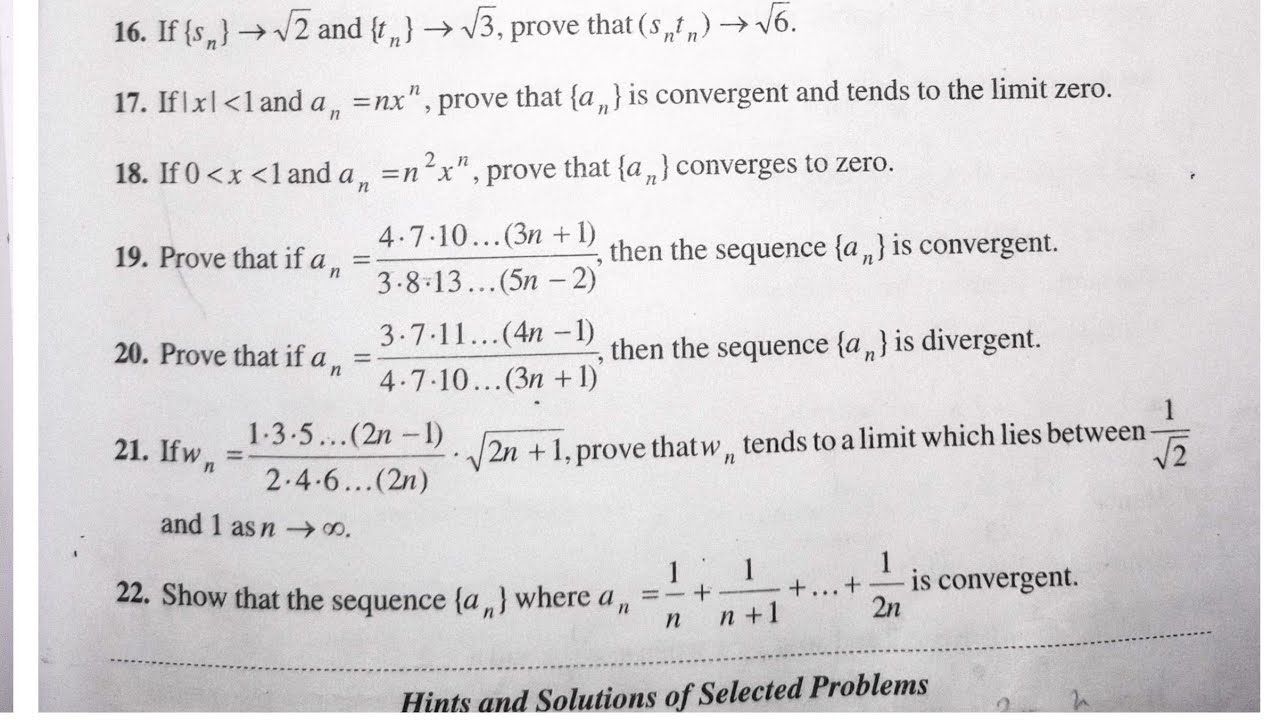
`EXERCISE 2 (A) 1. Construct a sequence of real numbers which is bounded but not convergent (1) convergent but not monotonic fm bounded but not monotonic 2. Prove that a motonic increasing sequence tends to limit or tends to infinity 3. Show that a convergent sequence is bounded. Discuss if the converse is true for u general er a monotonie sequence 4. Do the ratiomul numbers lying between 0 and 1 forma sequence 5. Give an example of a convergent sequence of rational numbers such that the limit is a Talsum number (1) animational number 1 2 3 6. Find 1 + 1 2 7. Prove that LL 8. Show that the following sequences are convergent 1 +2 +3 + + ++ 1+2+3+... fila 2 1) 9. Prove that it then 10. Prove that it and it - then - 11. Prove that to converges to implies to converges total What can you say about the converse? 12. Prove that the sequence(a Jeliverges lo zero where a = `.3. Promethat the women whosca terma 2 Contverpes to the limit 2 DI D- 21 14. Find the limit of the following sequences 1 ( 307 (111 ) On ) an +5 2x +1 15. Find the limit of the following sequences whose nth termes are (1) X in IV 11 (1 IV (1) vi) ` iv) 2+1-3 (vii) 1 ( vain 16. I and to prove that 27 Kanda = ` prove that is convergent and end to the limit zero 16. IOC can prove that converges over 47 10.30 19. Prove diatif then the sequence 4. Jis convergent 3.8.13 3.8 13.12 3.711... 14 -1 20. Prove that it then the scquence Jis divergent 47 10.3+ 21.11 2. prove them. Indsto limit which lies between 2.4.1.12) and I am 1 1 22. Show at the sequence where IN CURVINEN +1 21.Construct a sequence of real numbers which is (i) bounded but not convergent (ii) convergent but not monotonic (iii) bounded but not monotonic 2.Prove that a monotic increasing sequence tends to a limit or tends to infinity. 3.Show that a convergent sequence tends to a limit or tends to infinity. or a monotonic science. 4.Do the rational numbers lying between 0 and 1 form a sequence? 5.Give an example of a convergent sequence of rational numbers such that the limit is (i) a rational number (ii) an irrational number. 6.Find Lt_(-1)(1)/(n)((1)/(n)+(2)/(n))+(2)/(n)+(4)/(n)(ix ) cos(n pi)/(2)+(1)/(n) (x) sqrt(n)sin(n pi)/(2). 16. f' is sqrt(3) prove that (_(n))rarr sqrt(6). 17. ||x|1 and a_(n)=n^(2) prove that (a_(n))| is convergent and tends to the limit zero. 18.If |1 and a_(n)=n^(2)x^(n) prove that (a_(n))| is convergent. 19.Prove that if a_(n)=(4*7*10*(3n+1))/(4*70*1...(3n+1))/(4*70*(n+1) )/(4.70*1...(3n+1))/(4*70*1...(3n+1))/(4*70*((3n+1))/(4*70* (+1))/(4*70*( pi)) then the sequence (a_(n)| is comergent. 21.If w_(n)=(-3.5...(2n-1))/(2.4 *6...(2n-1))*sqrt(2n+1).prove that _(n)=(1)/(x)-(1)/(n+1)+...+(1 )/(2x) is`scu 19. Prove 20. Prove 3. Show that a convergentaquence is handed ora monotonic stice 4. Do the rational mumbers lying between formaGreatemple of a convergent sequence of rational members such as the limits a tubal number (animational number 11 Find te 21. If W and 1 7. Phone that I 21. Show IT Show that the following sequences are convergent 11.2.3 D (it) 1 0 9. Prove that - then 10. Prove that if : Ound if -0 then = 0 1+ 4. То 11. Provet What can you say about the converse? 12. Prove that the sequence ver converes to zero where `13.Prove that the sequence whose nn a_(n)=(4n^(2)+1)/(2n^(2)-30)(n2) conveges to the imit. 14.Find the limit o f the following sequences (i) (n^(2)+2)/(n^(2)-2) (ii) (2n^(2)-1)/(n^(2)-2) (iii ) (4n^(3)+3n-7)/(n^(2)-n^(2)+5) (iii) (4n^(3)+3n-7)/(n^(2)- n^(2)+1) 15.Find the limit of the following sequences whose n terms are (i) (n)/(n+1) (ii) (n)/(n+1) (iii) (20 ^(2)+3)/(n^(2)+4) (iv) (n^(2))/(n+2) (1)/(n)+(-1)^(n) ( vi) (-1)^(n)(n(n)/(2))/(n^(2)) 16.If (x_(n)) sqrt(2) and (h_(n))^( 2) 16.If (x_(n)) sqrt(2) and (n(n pi)/(2)) 16.If f(x_(n)) sqrt(2) and (n(n)/(n ))/(n^(2)) 16.If f(x_(n))rarr sqrt(3) prove that (a_(n))

#laljiprasadsolutioninfiniteseries
#laljiprasadsolution #infiniteseries #infiniteserieslaljiprasadsolution#realanalysislaljiprasad#laljiprasadarealanalysis#laljoorasadinfiniteseries #laljiprasadsequenceandseries