filmov
tv
Lie groups: Modular function

Показать описание
This lecture is part of an online graduate course on Lie groups.
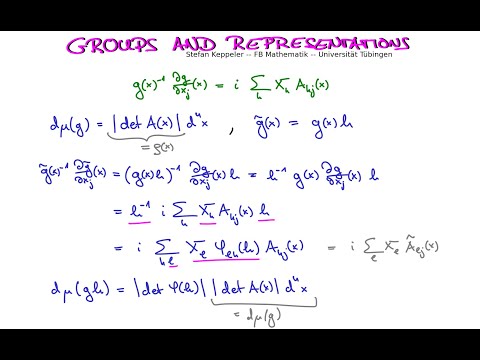
We discuss the modular function of a Lie group, which controls the relation between right and left invariant measures. We show how to use it to tell when a homogeneous space G/H has a G-invariant measure.
I will pause the lectures on Lie groups after this lecture, but will probably continue them sometime later.
We discuss the modular function of a Lie group, which controls the relation between right and left invariant measures. We show how to use it to tell when a homogeneous space G/H has a G-invariant measure.
I will pause the lectures on Lie groups after this lecture, but will probably continue them sometime later.
Lie groups: Modular function
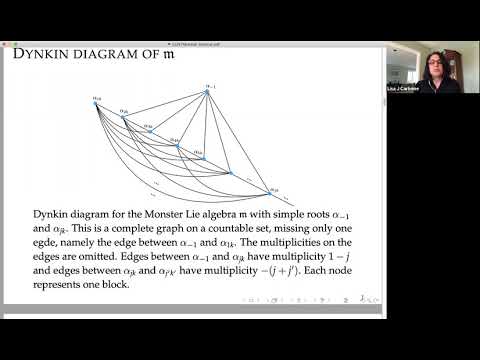
'Constructing Lie group analogs for infinite dimensional Lie algebras', Lisa Carbone, NYGT...
Lie groups: Introduction
Lie groups: Haar measure
Lie groups: Positive characteristic is weird
[Lie Groups and Lie Algebras] Lecture 9. Examples of highest weight modules
Haar measure 7 - right invariance and modular function
What is...modular representation theory?
Why greatest Mathematicians are not trying to prove Riemann Hypothesis? || #short #terencetao #maths
Introduction to Poisson Lie groups, Lie bialgebras, and their quantization I
Gerhard Hiss: Representation theory for groups of Lie type II
Yu. N. Kuznetsova. Multipliers on Lie groups generated by a distinguished Laplacian. 03.11.2023
Hilbert modular forms -II: L-functions - A. Raghuram
Weakly Modular Functions | The Geometry of SL2,Z, Section 1.4
Lie group
Group theory, abstraction, and the 196,883-dimensional monster
Cyclic Cohomology for Proper Lie Group Actions
Counting points on the E8 lattice with modular forms (theta functions) | #SoME2
Modular forms on exceptional groups
Webinar - Lie groups and special holonomy - Simon Salamon
Minoru Wakimoto, Mock modular forms and representation theory of affine Lie superalgebras
Benedict Gross, On the conjectures of Gan, Gross, and Prasad
Evgeny Ferapontov--Differential equations for modular forms and Jacobi forms
Matrix Lie Groups: Lecture 1 part 2: complex and quaternion
Комментарии
 0:28:57
0:28:57
 0:56:47
0:56:47
 0:36:14
0:36:14
 0:24:16
0:24:16
 0:21:08
0:21:08
 1:16:05
1:16:05
 0:05:56
0:05:56
 0:14:48
0:14:48
 0:00:38
0:00:38
 0:59:12
0:59:12
 0:54:35
0:54:35
 1:18:14
1:18:14
 1:18:57
1:18:57
 0:23:13
0:23:13
 0:12:48
0:12:48
 0:21:58
0:21:58
 0:53:17
0:53:17
 0:22:48
0:22:48
 0:51:43
0:51:43
 1:03:18
1:03:18
 0:26:12
0:26:12
 0:57:56
0:57:56
 0:59:37
0:59:37
 0:25:39
0:25:39