How to Find the Derivative of a^x from First Principles

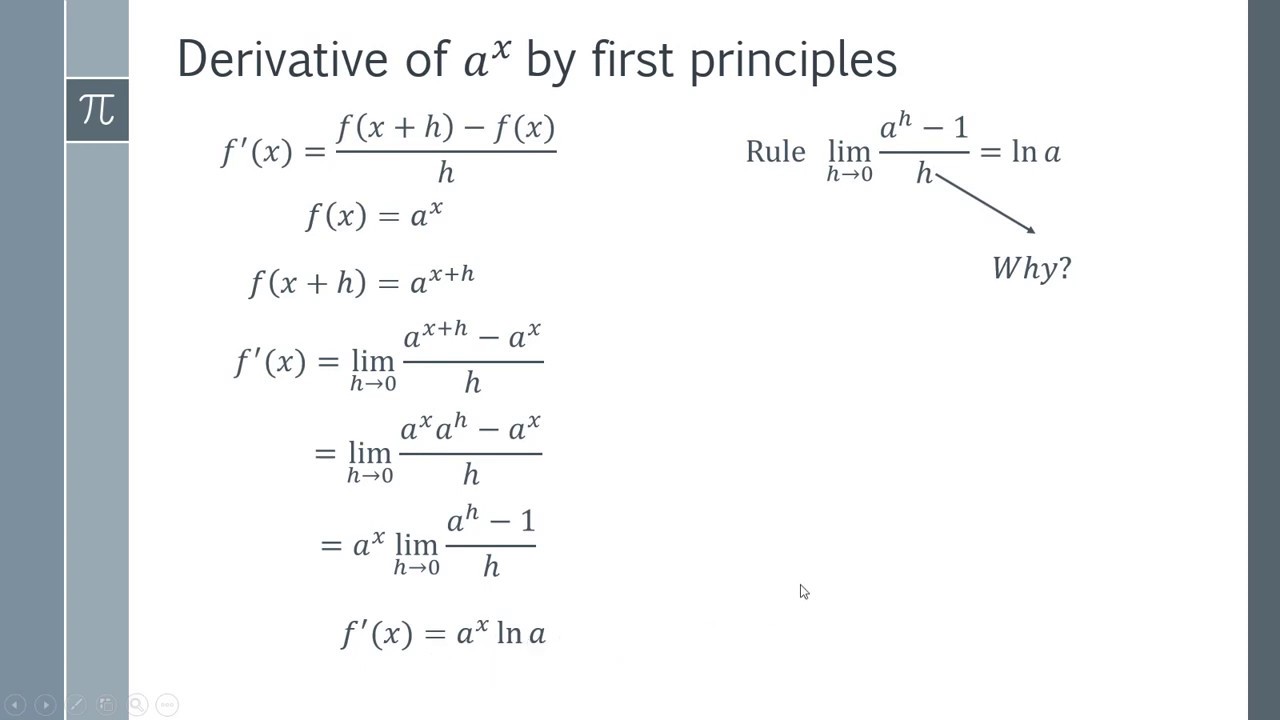
In this video I will give you a full proof of the derivative of a^x from first principles. This derivative is useful as it proves the derivative of any constant raised to the power of x such as 2^x and 3^x and so on.

[mistermonster]

How do you plan to use l’hopitals when you don’t actually know the derivative of e^(f(h)) or does this assume knowledge of differentiating e^x

[mokouf]

It can be done with substitution, which won't cause circular reasoning.
d/dx (a^x) = lim(h->0) (a^(x+h) - a^x)/h = a^x lim(h->0) (a^h -1)/h
u = u(h) = a^h -1, h = log_a(u+1) = ln(u+1)/ln(a)
u(0) = a^0 - 1 = 1-1 = 0
d/dx (a^x) = a^x lim(u->0) u ln(a)/ln(u+1) = a^x ln(a) lim(u->0) 1/ln(u+1)^1/u = a^x ln(a) / ln(e) = a^x ln(a)

[mokouf]

Can you find that limit withOUT using L'Hospitals rule?

[Levi--messi]

best explanation i could find on internet!!

[labibbidabibbadum]

It does feel a bit weird needing to rely on an advanced feature of calculus to show how calculus can be derived from first principles. But a thoroughly clear and well presented explanation nonetheless.

[Mphalliwell]

say you are differentiating e^x by first principles, then you end up multiplying e^x by the limit of (e^h-1)/h. How then can you use l'hopitals rule, as you assume the derivative of e^x is e^x

[deependraagarwal]

Thank you . It was a great explanation

[destruidor]

I hate this sort of prove where the people use l’hopitals to prove …so my question is simpler…so if I understood the prove was only possible after l’hopital rule was proved so you need to use something advance to prove something that should be the basis of calculus ( 1st principle) it sounds very strange.

[oidbio]

using L’Hopital’s rule is cheating…it’s circular logic. using the derivative to a^h to show the derivative of a^h…no way man. 😂

if h is going to 0 then (ln a)h is going to zero so do a substitution.