filmov
tv
find roots of a polynomial function using synthetic division and rational root theorem.

Показать описание
Question: Find all the zeros of f(x) = 9x^3 + 15x^2 - 30x + 18. Enter the zeros separated by commas. Enter the exact value, not decimal approximations.
To receive credit for this question, the following steps must be presented in the solution:- Step 1: List all potential rational zeros of the function using Rational Zero Theorem.- Step 2: Use the remainder theorem to determine whether the potential rational zeros are roots of the function. If the remainder is 0, the candidate is a zero. If the remainder is not 0, discard the candidate.- Step 3: Divide the polynomial function by the root obtained in the step 2 using synthetic division or long division. A quotient will be drived at this step.- Step 4: If the quotient is a quadratic, go to the step 5. If the quotient is not a quadratic, repeat the steps 2 & 3 until the quotient is a quadratic.- Step 5: Use the quotient to find the remaining zeros by factoring or the quadratic formula.(Step by step walkthrough would be appreciated)
------------------------
Answered By:
Doug C.
Math Tutor with Reputation to make difficult concepts understandable
------------------------
------------------------
About: Wyzant Ask an Expert offers free answers to your toughest academic and professional questions from over 65,000 verified experts. It’s trusted by millions of students each month with the majority of questions receiving an answer within 1 hour of being asked. If you ever need more than just an answer, Wyzant also offers personalized 1-on-1 sessions with experts that will work with you to help you understand whatever you’re trying to learn.
 0:02:43
0:02:43
 0:12:18
0:12:18
 0:03:22
0:03:22
 0:05:08
0:05:08
 0:25:31
0:25:31
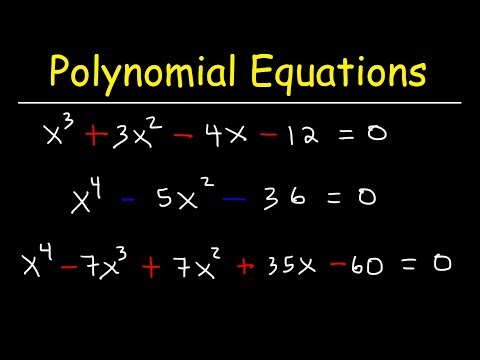 0:14:19
0:14:19
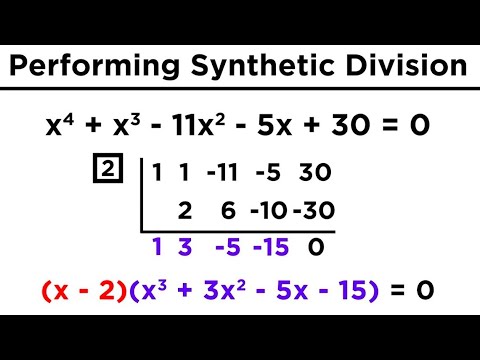 0:09:22
0:09:22
 0:01:00
0:01:00
 1:07:31
1:07:31
![[Tagalog] Finding roots](https://i.ytimg.com/vi/DXNxUgLstJc/hqdefault.jpg) 0:16:46
0:16:46
 0:08:02
0:08:02
 0:17:16
0:17:16
 0:03:11
0:03:11
 0:37:37
0:37:37
 0:26:42
0:26:42
 0:02:24
0:02:24
 0:05:35
0:05:35
 0:11:01
0:11:01
 0:06:02
0:06:02
 0:05:59
0:05:59
 0:09:08
0:09:08
 0:04:06
0:04:06
 0:08:22
0:08:22
 0:05:06
0:05:06