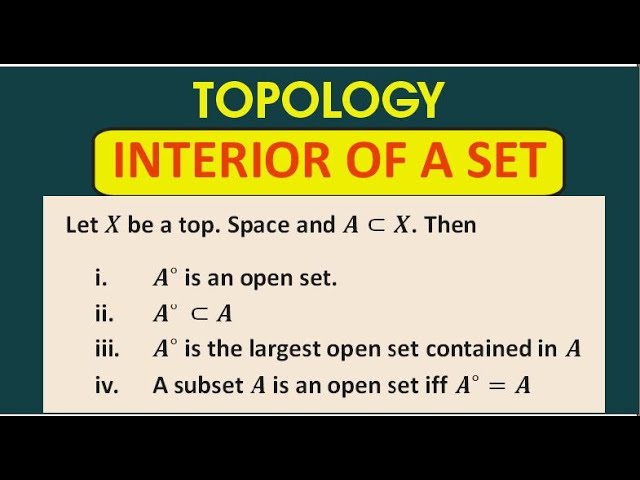
T14 : TOPOLOGY || Interior Of A Set Is The Largest Open Set Contained In A / Open Set

preview_player
Показать описание
#InteriorOfASet #TOPOLOGY #InteriorOfASet #LargestOpenSet #ContainedInA #openset
#Topology #LimitPoints #AccumulationPoint #ClusterPoint #t12 #LimitPointsOfatopologicalSpace #Properties #DerivedSets
#Closure #ClosureOfASubsetOfATopSpace
Topology

T1: TOPOLOGY ||Definition/Example/Topological Space/Open Sets Of a Topological Space/Topology-I

T2: TOPOLOGY || Definition/Example/co-finite Topology/Finite complement/Topological Space/Open Sets

T3: TOPOLOGY || Definition/Intersection of two topologies on X is a topology on X/Topological Space

T4: TOPOLOGY || Closed Sets Of a Topological Space/Definition/Examples

T5 : TOPOLOGY || Closed Sets/A subset A of a top. space X is open in X iff X-A is closed in X

T6 : TOPOLOGY || Closed Sets/In the Real Space (R,u) every singleton subset of R is a Closed Set

T7 : TOPOLOGY || Closure of a Set/Definition/Examples/Mathematical Notation/Closed Sets

T8:TOPOLOGY||Closure of a Set/A is Subset of Cl(A)/Cl(A) is smallest closed set containing A/A=cl(A)

T9 : TOPOLOGY || Closure Of Set/Some Properties Of Closure Of Subsets Of A Top. Space/Closed Sets

T10 : TOPOLOGY || Properties Of Closure On Intersection Of Two Subsets Of A Space/ Examples

  1. SimplyDoMaths
Рекомендации по теме
T14 : TOPOLOGY 0:09:59

T14 : TOPOLOGY || Interior Of A Set Is The Largest Open Set Contained In A / Open Set

T16 : TOPOLOGY 0:07:57

T16 : TOPOLOGY || Interior Of A Set / Important Properties with Proof-2/ Open Set

T15 : TOPOLOGY 0:09:22

T15 : TOPOLOGY || Interior Of A Set / Important Properties with Proof / Open Set

OVERVIEW OF OPEN,CLOSED,INTERIOR 0:00:47

OVERVIEW OF OPEN,CLOSED,INTERIOR AND CLOSURE SET OF A TOPOLOGY

Topology | (Lecture 0:13:39

Topology | (Lecture -12) | interior operator in topology in hindi

T17 : TOPOLOGY 0:06:07

T17 : TOPOLOGY || X - int(A) = cl(X-A) Interior and Closure Of A Set In Topological Space

Interior,exterior & frontier 0:36:22

Interior,exterior & frontier of a set in topological space|Definition,examples & theorems||L...

Topological Spaces, Lec#19, 0:36:40

Topological Spaces, Lec#19, Interior of a Set is its Largest Open Subset

Examples of interior 0:01:01

Examples of interior point of a set in a topology | The Mathematics Outlet | Lec 11

Int (A) is 0:05:53

Int (A) is the largest open set of A

Topology | Topology 1:01:10

Topology | Topology lecture 5 | Interior point and interior of a set - interior point in topology

IntA is the 0:04:02

IntA is the largest open set contained in A || Topology || Theorem

Interior point of 0:10:12

Interior point of a set and interior of a set in topological space

Interior of a 0:07:53

Interior of a Set A is Largest Open Subset of A

Metric Spaces | 0:06:40

Metric Spaces | Lecture 44 | Intersection of Interior of Two Sets

Interior of A 0:03:35

Interior of A is largest open subset of A in metric space- Theorem

Interior and exterior 0:14:28

Interior and exterior of a set | Boundary of a set | Examples | Lecture 15 || Set Topology

interior of set 0:09:24

interior of set A is equal to union of all open set which contain in A| theorem proof |

Theorems on interior 0:05:09

Theorems on interior of a set part 2

A interior is 0:08:48

A interior is the largest Open subset Of A | Point set Topology | SAINI CLASSES

Set A is 0:05:56

Set A is open if and Only if A is equal to A interior | Point Set Topology | SAINI CLASSES

Topology/(Lecture -26)/closure of 0:06:53

Topology/(Lecture -26)/closure of a set related theorem/closure of A is smallest set containing A.

IntA is the 0:01:31

IntA is the union of all open sets contained in A ||Topology ||Theorem

topology | (Lecture-09)| 0:07:46

topology | (Lecture-09)| Let (X,T) be a topological space and let A subset of X then A° is open set