filmov
tv
Laplace transform of e^(-at) sinwt

Показать описание
Laplace transform of e^(-at) sinwt
#LaplaceTransformOfe^(-at)sinwt
#LaplaceTransformOfe^(-bt)sinwt
#LaplaceTransformOfe^(-ct)sinwt
#LaplaceTransformOfe^(-dt)sinwt
#LaplaceTransformOfe^(-et)sinwt
#LaplaceTransformOfe^(-ft)sinwt
#LaplaceTransformOfe^(-gt)sinwt
#LaplaceTransformOfe^(-ht)sinwt
#LaplaceTransformOfe^(-it)sinwt
#LaplaceTransformOfe^(-jt)sinwt
#LaplaceTransformOfe^(-kt)sinwt
#LaplaceTransformOfe^(-lt)sinwt
#LaplaceTransformOfe^(-mt)sinwt
#LaplaceTransformOfe^(-nt)sinwt
#LaplaceTransformOfe^(-ot)sinwt
#LaplaceTransformOfe^(-pt)sinwt
#LaplaceTransformOfe^(-qt)sinwt
#LaplaceTransformOfe^(-rt)sinwt
#LaplaceTransformOfe^(-st)sinwt
#LaplaceTransformOfe^(-tt)sinwt
#LaplaceTransformOfe^(-ut)sinwt
#LaplaceTransformOfe^(-vt)sinwt
#LaplaceTransformOfe^(-wt)sinwt
#LaplaceTransformOfe^(-xt)sinwt
#LaplaceTransformOfe^(-yt)sinwt
#LaplaceTransformOfe^(-zt)sinwt
#LaplaceTransformOfe^(-at)sinwt=w/[(a+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-bt)sinwt=w/[(b+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-ct)sinwt=w/[(c+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-dt)sinwt=w/[(d+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-et)sinwt=w/[(e+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-ft)sinwt=w/[(f+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-gt)sinwt=w/[(g+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-ht)sinwt=w/[(h+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-it)sinwt=w/[(i+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-jt)sinwt=w/[(j+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-kt)sinwt=w/[(k+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-lt)sinwt=w/[(l+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-mt)sinwt=w/[(m+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-nt)sinwt=w/[(n+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-ot)sinwt=w/[(o+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-pt)sinwt=w/[(p+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-qt)sinwt=w/[(q+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-rt)sinwt=w/[(r+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-st)sinwt=w/[(s+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-tt)sinwt=w/[(t+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-ut)sinwt=w/[(u+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-vt)sinwt=w/[(v+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-wt)sinwt=w/[(w+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-xt)sinwt=w/[(x+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-yt)sinwt=w/[(y+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-zt)sinwt=w/[(z+s)^(2)+w^(2)]
#L[e^(-at)sinwt]=w/[(a+s)^(2)+w^(2)]
#L[e^(-bt)sinwt]=w/[(b+s)^(2)+w^(2)]
#L[e^(-ct)sinwt]=w/[(c+s)^(2)+w^(2)]
#L[e^(-dt)sinwt]=w/[(d+s)^(2)+w^(2)]
#L[e^(-et)sinwt]=w/[(e+s)^(2)+w^(2)]
#L[e^(-ft)sinwt]=w/[(f+s)^(2)+w^(2)]
#L[e^(-gt)sinwt]=w/[(g+s)^(2)+w^(2)]
#L[e^(-ht)sinwt]=w/[(h+s)^(2)+w^(2)]
#L[e^(-it)sinwt]=w/[(i+s)^(2)+w^(2)]
#L[e^(-jt)sinwt]=w/[(j+s)^(2)+w^(2)]
#L[e^(-kt)sinwt]=w/[(k+s)^(2)+w^(2)]
#L[e^(-lt)sinwt]=w/[(l+s)^(2)+w^(2)]
#L[e^(-mt)sinwt]=w/[(m+s)^(2)+w^(2)]
#L[e^(-nt)sinwt]=w/[(n+s)^(2)+w^(2)]
#L[e^(-ot)sinwt]=w/[(o+s)^(2)+w^(2)]
#L[e^(-pt)sinwt]=w/[(p+s)^(2)+w^(2)]
#L[e^(-qt)sinwt]=w/[(q+s)^(2)+w^(2)]
#L[e^(-rt)sinwt]=w/[(r+s)^(2)+w^(2)]
#L[e^(-st)sinwt]=w/[(s+s)^(2)+w^(2)]
#L[e^(-tt)sinwt]=w/[(t+s)^(2)+w^(2)]
#L[e^(-ut)sinwt]=w/[(u+s)^(2)+w^(2)]
#L[e^(-vt)sinwt]=w/[(v+s)^(2)+w^(2)]
#L[e^(-wt)sinwt]=w/[(w+s)^(2)+w^(2)]
#L[e^(-xt)sinwt]=w/[(x+s)^(2)+w^(2)]
#L[e^(-yt)sinwt]=w/[(y+s)^(2)+w^(2)]
#L[e^(-zt)sinwt]=w/[(z+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-at)sinwt
#LaplaceTransformOfe^(-bt)sinwt
#LaplaceTransformOfe^(-ct)sinwt
#LaplaceTransformOfe^(-dt)sinwt
#LaplaceTransformOfe^(-et)sinwt
#LaplaceTransformOfe^(-ft)sinwt
#LaplaceTransformOfe^(-gt)sinwt
#LaplaceTransformOfe^(-ht)sinwt
#LaplaceTransformOfe^(-it)sinwt
#LaplaceTransformOfe^(-jt)sinwt
#LaplaceTransformOfe^(-kt)sinwt
#LaplaceTransformOfe^(-lt)sinwt
#LaplaceTransformOfe^(-mt)sinwt
#LaplaceTransformOfe^(-nt)sinwt
#LaplaceTransformOfe^(-ot)sinwt
#LaplaceTransformOfe^(-pt)sinwt
#LaplaceTransformOfe^(-qt)sinwt
#LaplaceTransformOfe^(-rt)sinwt
#LaplaceTransformOfe^(-st)sinwt
#LaplaceTransformOfe^(-tt)sinwt
#LaplaceTransformOfe^(-ut)sinwt
#LaplaceTransformOfe^(-vt)sinwt
#LaplaceTransformOfe^(-wt)sinwt
#LaplaceTransformOfe^(-xt)sinwt
#LaplaceTransformOfe^(-yt)sinwt
#LaplaceTransformOfe^(-zt)sinwt
#LaplaceTransformOfe^(-at)sinwt=w/[(a+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-bt)sinwt=w/[(b+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-ct)sinwt=w/[(c+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-dt)sinwt=w/[(d+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-et)sinwt=w/[(e+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-ft)sinwt=w/[(f+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-gt)sinwt=w/[(g+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-ht)sinwt=w/[(h+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-it)sinwt=w/[(i+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-jt)sinwt=w/[(j+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-kt)sinwt=w/[(k+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-lt)sinwt=w/[(l+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-mt)sinwt=w/[(m+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-nt)sinwt=w/[(n+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-ot)sinwt=w/[(o+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-pt)sinwt=w/[(p+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-qt)sinwt=w/[(q+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-rt)sinwt=w/[(r+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-st)sinwt=w/[(s+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-tt)sinwt=w/[(t+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-ut)sinwt=w/[(u+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-vt)sinwt=w/[(v+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-wt)sinwt=w/[(w+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-xt)sinwt=w/[(x+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-yt)sinwt=w/[(y+s)^(2)+w^(2)]
#LaplaceTransformOfe^(-zt)sinwt=w/[(z+s)^(2)+w^(2)]
#L[e^(-at)sinwt]=w/[(a+s)^(2)+w^(2)]
#L[e^(-bt)sinwt]=w/[(b+s)^(2)+w^(2)]
#L[e^(-ct)sinwt]=w/[(c+s)^(2)+w^(2)]
#L[e^(-dt)sinwt]=w/[(d+s)^(2)+w^(2)]
#L[e^(-et)sinwt]=w/[(e+s)^(2)+w^(2)]
#L[e^(-ft)sinwt]=w/[(f+s)^(2)+w^(2)]
#L[e^(-gt)sinwt]=w/[(g+s)^(2)+w^(2)]
#L[e^(-ht)sinwt]=w/[(h+s)^(2)+w^(2)]
#L[e^(-it)sinwt]=w/[(i+s)^(2)+w^(2)]
#L[e^(-jt)sinwt]=w/[(j+s)^(2)+w^(2)]
#L[e^(-kt)sinwt]=w/[(k+s)^(2)+w^(2)]
#L[e^(-lt)sinwt]=w/[(l+s)^(2)+w^(2)]
#L[e^(-mt)sinwt]=w/[(m+s)^(2)+w^(2)]
#L[e^(-nt)sinwt]=w/[(n+s)^(2)+w^(2)]
#L[e^(-ot)sinwt]=w/[(o+s)^(2)+w^(2)]
#L[e^(-pt)sinwt]=w/[(p+s)^(2)+w^(2)]
#L[e^(-qt)sinwt]=w/[(q+s)^(2)+w^(2)]
#L[e^(-rt)sinwt]=w/[(r+s)^(2)+w^(2)]
#L[e^(-st)sinwt]=w/[(s+s)^(2)+w^(2)]
#L[e^(-tt)sinwt]=w/[(t+s)^(2)+w^(2)]
#L[e^(-ut)sinwt]=w/[(u+s)^(2)+w^(2)]
#L[e^(-vt)sinwt]=w/[(v+s)^(2)+w^(2)]
#L[e^(-wt)sinwt]=w/[(w+s)^(2)+w^(2)]
#L[e^(-xt)sinwt]=w/[(x+s)^(2)+w^(2)]
#L[e^(-yt)sinwt]=w/[(y+s)^(2)+w^(2)]
#L[e^(-zt)sinwt]=w/[(z+s)^(2)+w^(2)]
Комментарии
 0:11:43
0:11:43
 0:13:19
0:13:19
 0:14:02
0:14:02
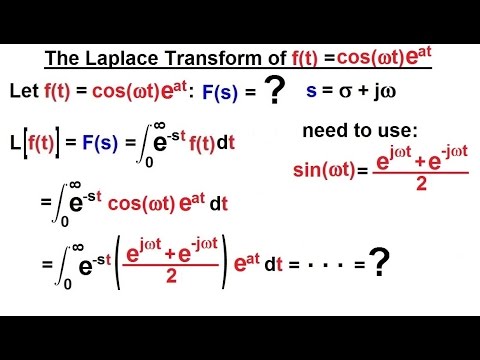 0:07:10
0:07:10
 0:08:53
0:08:53
 0:08:28
0:08:28
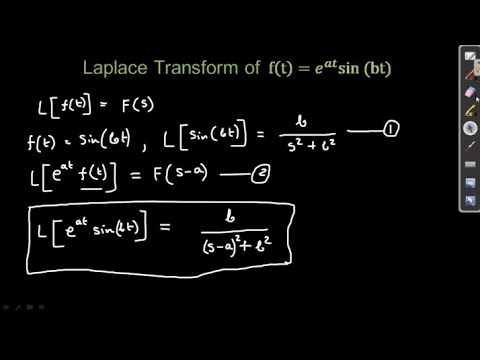 0:04:02
0:04:02
 0:02:18
0:02:18
 0:15:47
0:15:47
 0:06:28
0:06:28
 0:06:48
0:06:48
 0:08:11
0:08:11
 0:01:45
0:01:45
 0:11:40
0:11:40
 0:00:17
0:00:17
 0:04:18
0:04:18
 0:09:20
0:09:20
 0:09:51
0:09:51
 0:00:31
0:00:31
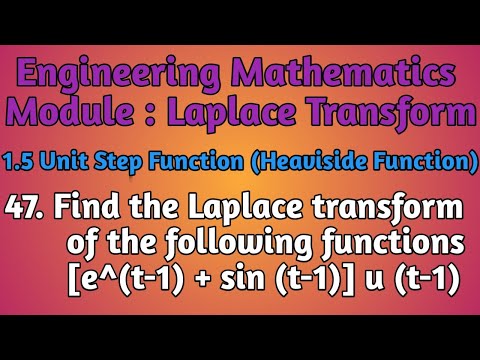 0:08:08
0:08:08
 0:00:15
0:00:15
 0:12:21
0:12:21
 0:01:46
0:01:46
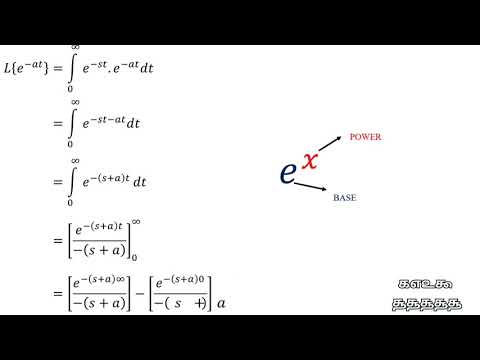 0:01:36
0:01:36