filmov
tv
AlgTop16: Rational curvature of polytopes and the Euler number
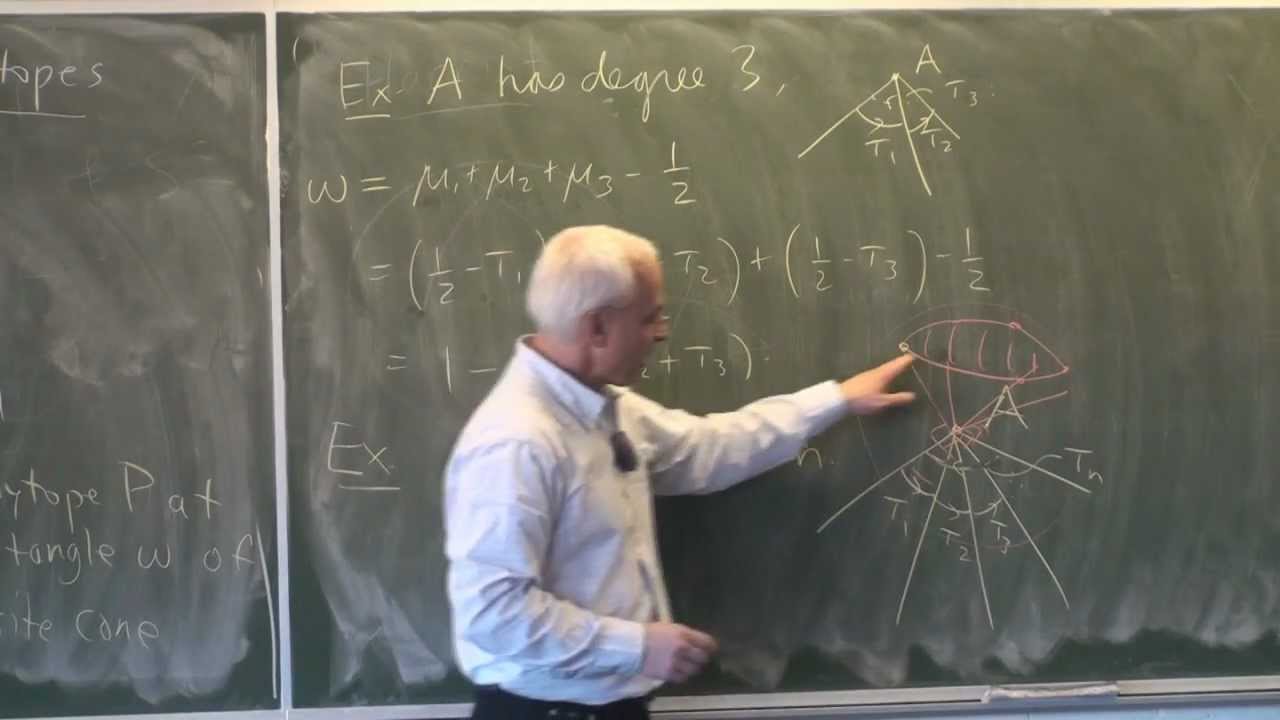
Показать описание
We show that the total curvature of a polyhedron is equal to its Euler number. This only works with the rational formulation of curvature, using an analog of the turn angle suitable for the 2 dimensional sphere. We treat Harriot's theorem on the area of a spherical polygon.
This is the first video in the 16th lecture of this beginner's course in Algebraic Topology, given by N J Wildberger at UNSW.
Video Contents:
00:00 Introduction
00:40 Harriott's theorem
02:14 Ex. A has degree 3
04:20 Ex. A has degree 4
07:00 Tangles of opposite Cone at vertex A has internal tangles
10:47 Theorem. IF A polygon P has vertex A with tangles of facesat A.
11:47 Tetrahedron
14:40 Ex.2 Cube
15:50 Ex.3 Octahedron
16:29 Ex.4 Cosahedron
17:40 Ex.5 Dodecahedron
20:00 Problem 18. Computing directly the curvature at a vertex
32:09 Problem 19. Compute directly the curvatures
************************
Here are the Insights into Mathematics Playlists:
Here are the Wild Egg Maths Playlists (some available only to Members!)
************************
This is the first video in the 16th lecture of this beginner's course in Algebraic Topology, given by N J Wildberger at UNSW.
Video Contents:
00:00 Introduction
00:40 Harriott's theorem
02:14 Ex. A has degree 3
04:20 Ex. A has degree 4
07:00 Tangles of opposite Cone at vertex A has internal tangles
10:47 Theorem. IF A polygon P has vertex A with tangles of facesat A.
11:47 Tetrahedron
14:40 Ex.2 Cube
15:50 Ex.3 Octahedron
16:29 Ex.4 Cosahedron
17:40 Ex.5 Dodecahedron
20:00 Problem 18. Computing directly the curvature at a vertex
32:09 Problem 19. Compute directly the curvatures
************************
Here are the Insights into Mathematics Playlists:
Here are the Wild Egg Maths Playlists (some available only to Members!)
************************
AlgTop16: Rational curvature of polytopes and the Euler number
AlgTop16: Rational curvature of polytopes and the Euler number
Rational curvature of a polytope | Algebraic Topology | NJ Wildberger
AlgTop15: Rational curvature of a polytope
Zero total curvature
Rational curvature, winding and turning | Algebraic Topology | NJ Wildberger
Tessellabilities, Reversibilities, and Decomposabilities of Polytopes (1) Jin Akiyama
Decompositions of Ehrhart h*-Polynomials for Rational Polytopes (Andrés R. Vindas Meléndez)
Classification of combinatorial surfaces (II) | Algebraic Topology | NJ Wildberger
Algebraic Topology Lecture 15
Algebraic Topology: L10, homology functor, 9-27-16, part 2
An algebraic ZIP proof of the classification | Algebraic Topology | NJ Wildberger
Duality for polygons and the Fundamental theorem of Algebra | Algebraic Topology | NJ Wildberger
Classification of combinatorial surfaces (I) | Algebraic Topology | NJ Wildberger
The Klein bottle and projective plane | Algebraic Topology | NJ Wildberger
Relative Mass in Surfaces of Constant Curvature (Part 4)
AlgTop19: An algebraic ZIP proof
The perspective image of a square III --- the solution | FamousMathProbs18c | N J Wildberger
Alexandru Dimca: Betti numbers of hypersurfaces and defects of linear systems I
Applications of Euler's formula and graphs | Algebraic Topology | NJ Wildberger
AlgTop20: The geometry of surfaces
AlgTop25: More on the fundamental group
GSS Fall 2017 - Kerrek Stinson: A randomized algorithm for enumerating zonotope vertices
AlgTop17: Classification of combinatorial surfaces I
Комментарии
 0:35:28
0:35:28
 0:35:28
0:35:28
 0:50:23
0:50:23
 0:50:23
0:50:23
 0:00:08
0:00:08
 0:48:19
0:48:19
 0:17:02
0:17:02
 0:31:16
0:31:16
 1:00:21
1:00:21
 0:30:31
0:30:31
 0:08:04
0:08:04
 0:42:23
0:42:23
 0:45:36
0:45:36
 0:50:32
0:50:32
 0:39:42
0:39:42
 0:31:13
0:31:13
 0:42:23
0:42:23
 0:27:58
0:27:58
 1:06:31
1:06:31
 0:42:23
0:42:23
 0:43:54
0:43:54
 0:34:56
0:34:56
 1:15:17
1:15:17
 0:50:32
0:50:32