Problems on Trains Shortcuts Tips and Tricks(Question 1) | Bank PO Aptitude Exam

Transcription
=============

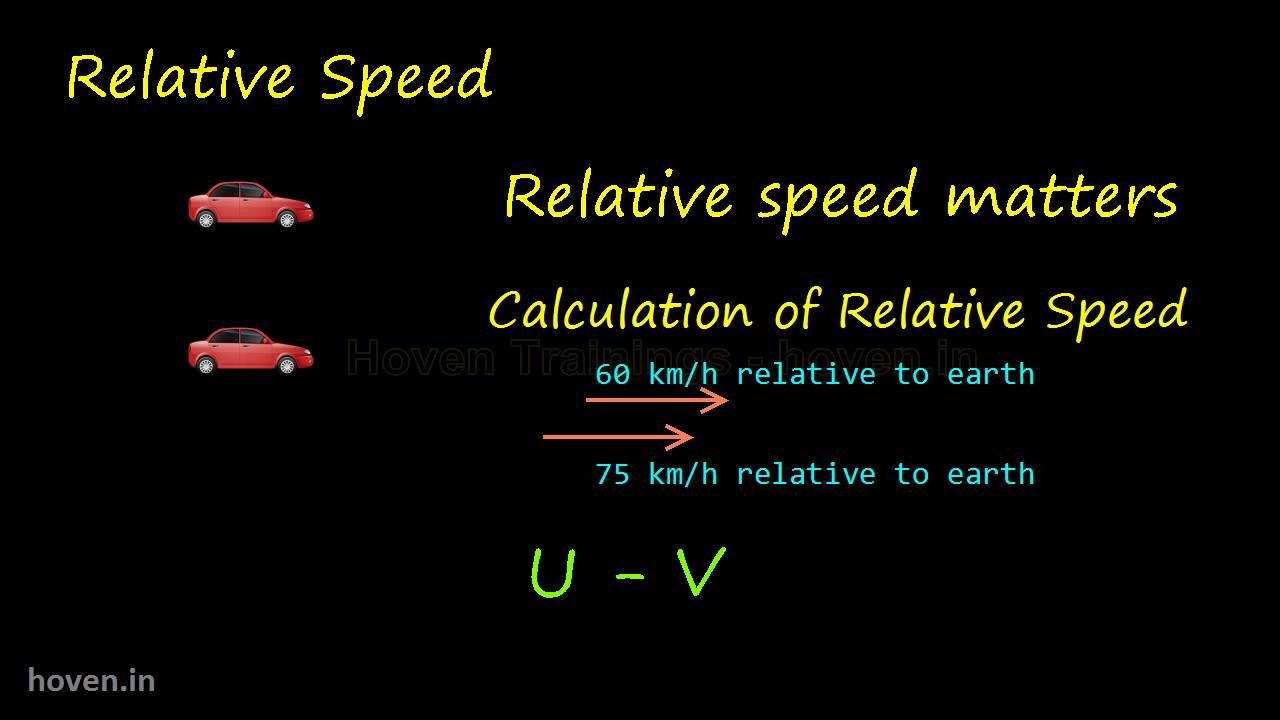
Two trains are travelling in same direction at 60 and 75 kilometer per hour.
The faster crosses a man sitting in the slower train in 30 sec.
What is the length of the longer train?
Suppose this is the slower train running at 60.
Suppose this is the man sitting in this train.
The man is also travelling at 60.
Let us suppose this is the faster train.
Let its length be L.
We have to determine this length.
The relative speed between the faster train and the man is 75 minus 60 equal to 15.
Convert the speed to meters per second.
The distance covered in 1 second would be 25 upon 6 meters.
This is the definition of speed.
So the distance covered in 30 seconds would be 125 meters.
This is the length of the train.
Let me explain the shortcut that can be used to solve this question.
First of all you need to know about relative speed.
What do we mean when we say a car is travelling at 30?
The 30 is the relative speed with respect to the ground, or earth.
Is n't it?
Suppose there is another car also travelling at 30.
Here also the speed of 30 is relative to the earth.
The second car cannot overtake the first car because it has no speed advantage over the first.
It is moving at zero km per hour with respect to the first.
If an object has to move relative to another, then it must have a relative speed.
A car can travel distances on earth because it has a relative speed with respect to earth.
Let us see how to calculate the relative speeds.
Suppose this object is moving at 60.
Let us suppose this object also moves in the same direction at 75.
We can see that the net speed is of 15 only.
The net or relative speed is the difference between the speeds of the two objects.
For objects moving in the same direction, the relative speed is the difference.
Net relative speed between two objects in the same direction is the difference.
The relative speed of two objects moving in opposite direction is the sum of their speeds.
Suppose this is a train of length L.
Also suppose that the train is moving at V meters per second.
Let this be some object.
This object is moving at a speed of U towards the right side.
The train will cross the object completely if it covers a relative distance of L.
The distance L will be covered at a relative speed of V minus U.
When the distance is relative, the speed must also be relative.
We can now write a formula.
We know distance is speed into time.
So we can easily write this equation.
Remember this shortcut.
Let us now use the shortcut formula for solving our question.
This is the shortcut formula that we derived just now.
Putting the values in this formula, we get our answer of 125 meters.
What happens if the trains moved in opposite directions?
In that case the relative speed would be the sum of the speeds.
The answer would change to eleven twenty-five.
If the man also walked in the train at 6.
Then the relative speed would be 75 minus 60 minus 6 equal to 9.
In that case the answer would be 75 meters.