filmov
tv
Abstract Alg, Lec 25A: Ring Integral Domains, Fields, Ring Characteristic, Nilpotents & Idempotents

Показать описание
Abstract Algebra, Lecture 25A.
(0:00) The Great Tau-Shaped Superstorm of April 2018.
(4:08) Lecture summary (make sure you study more complicated examples before Lecture 26).
(5:57) Review the idea of the group of units of a commutative ring and examples.
(9:03) Review definition of zero-divisor (consider an example of finding solutions of a polynomial equation in different rings), integral domain (no zero-divisors, so multiplicative cancellation law holds), field, basic examples and properties.
(21:21) Characteristic of a ring and basic theorems.
(27:33) Nilpotent and idempotent elements in a ring and basic examples and facts.
AMAZON ASSOCIATE
As an Amazon Associate I earn from qualifying purchases.
Abstract Alg, Lec 25A: Ring Integral Domains, Fields, Ring Characteristic, Nilpotents & Idempote...
Abstract Algebra, Lec 25B: Ring Ideals, Factor (Quotient) Rings, Prime Ideals, Maximal Ideals
Abstract Algebra, Lec 26A: Polynomials over a Commutative Ring and over a Field, Division Algorithm
Abstract Algebra: Mod p Irreducibility Test, Field Constructed as a Factor Ring of a Polynomial Ring
Showing that an Element from a Ring is Idempotent
Ring Homomorphisms | Modern Algebra
Abstract Algeba: L23, rings and integral domains, 10-31-16
Abstract Algebra, Lec 24B: Subrings, Subring Test, Groups of Units, Integral Domains, Fields
Zero Divisors and Unit of a Ring
Abstract Algebra: Abelian Groups of Order 108 and 32, Ring Examples, Ring Definition
Idempotent, Nilpotent, Units ( Part-3 )
Characteristics of Ring in urdu/hindi| ring theory| Abstract Algebra| Jester Mathematician
Lecture 25, MTH 204A (Section B)
Characteristics of a Ring
AR05 Ring Homomorphisms, Kernels, and Ideals
Algebra: ring and field theory. Lecture 2, math 100 c, UCSD
# 13 Nilpotent Element / Ring Theory
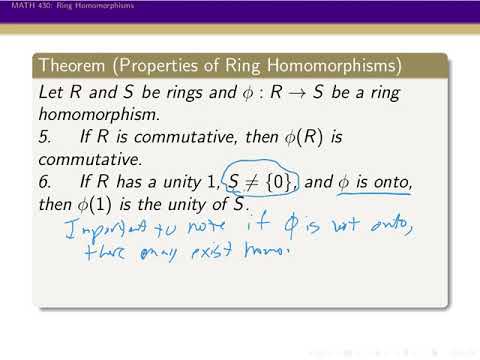
MATH 430 — 20: Ring Homomorphisms
Idempotent, Nilpotent, Units ( Part-1)
Commutative algebra 25 Artinian rings
Fields and Integral Domains
NBHM PhD MATHEMATICS - 2021 | Abstract Algebra, Question - 15; Section - A | Idempotent Elements
Abstract Algebra Exam 1 Review Problems and Solutions
Chapter 27: Integral Domains and Fields
Комментарии
 0:33:49
0:33:49
 0:30:19
0:30:19
 0:37:30
0:37:30
 1:04:11
1:04:11
 0:03:08
0:03:08
 1:13:02
1:13:02
 0:51:20
0:51:20
 0:29:10
0:29:10
 0:12:50
0:12:50
 1:00:00
1:00:00
 0:11:51
0:11:51
 0:03:01
0:03:01
 1:12:13
1:12:13
 0:04:55
0:04:55
 0:31:42
0:31:42
 1:23:52
1:23:52
 0:13:41
0:13:41
 0:35:53
0:35:53
 1:01:03
1:01:03
 0:21:24
0:21:24
 0:07:18
0:07:18
 0:01:54
0:01:54
 1:22:00
1:22:00
 0:23:07
0:23:07