filmov
tv
An algebraic infinitesimal approach to product and chain rules | FMP 22c | N J Wildberger

Показать описание
In this video we give the algebraic framework for a general infinitesimal approach to the Derivative of Faulhaber, valid for a general field F. We rely both on the notion of a dual complex number over F, and the idea of a bi-polynumber. Dual complex numbers are 2 x 2 matrices that incorporate and algebraic infinitesimal epsilon. Bi-polynumbers are data structures that capture the idea of a polynomial function of two variables, but crucially the notion of "variable" here is replaced rather by specific data structures.
With these preliminaries, we introduce the important Derivative theorem. This is a somewhat novel way of stating exactly what it is that happens when we take a Derivative, but without limits, and without "real numbers". Then we derive the Product Rule and the Chain Rule, again in a very general context.
These important directions will be developed at greater length and with lots of examples in the Algebraic Calculus Two online course.
************************
Here are the Insights into Mathematics Playlists:
Here are the Wild Egg Maths Playlists (some available only to Members!)
************************
With these preliminaries, we introduce the important Derivative theorem. This is a somewhat novel way of stating exactly what it is that happens when we take a Derivative, but without limits, and without "real numbers". Then we derive the Product Rule and the Chain Rule, again in a very general context.
These important directions will be developed at greater length and with lots of examples in the Algebraic Calculus Two online course.
************************
Here are the Insights into Mathematics Playlists:
Here are the Wild Egg Maths Playlists (some available only to Members!)
************************
Комментарии
 0:29:41
0:29:41
 0:08:31
0:08:31
 0:08:37
0:08:37
 0:39:32
0:39:32
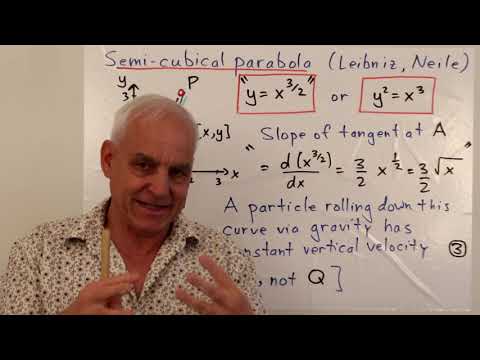 0:33:44
0:33:44
 0:03:01
0:03:01
 0:00:51
0:00:51
 0:03:17
0:03:17
 0:30:17
0:30:17
 0:01:00
0:01:00
 0:19:34
0:19:34
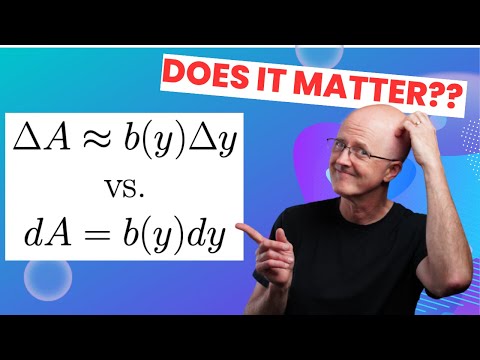 0:19:50
0:19:50
 0:06:00
0:06:00
 0:38:08
0:38:08
 0:00:28
0:00:28
 0:00:09
0:00:09
 0:09:33
0:09:33
 0:17:05
0:17:05
 0:33:54
0:33:54
 0:03:29
0:03:29
 0:01:26
0:01:26
 0:11:48
0:11:48
 0:27:03
0:27:03
 0:24:15
0:24:15