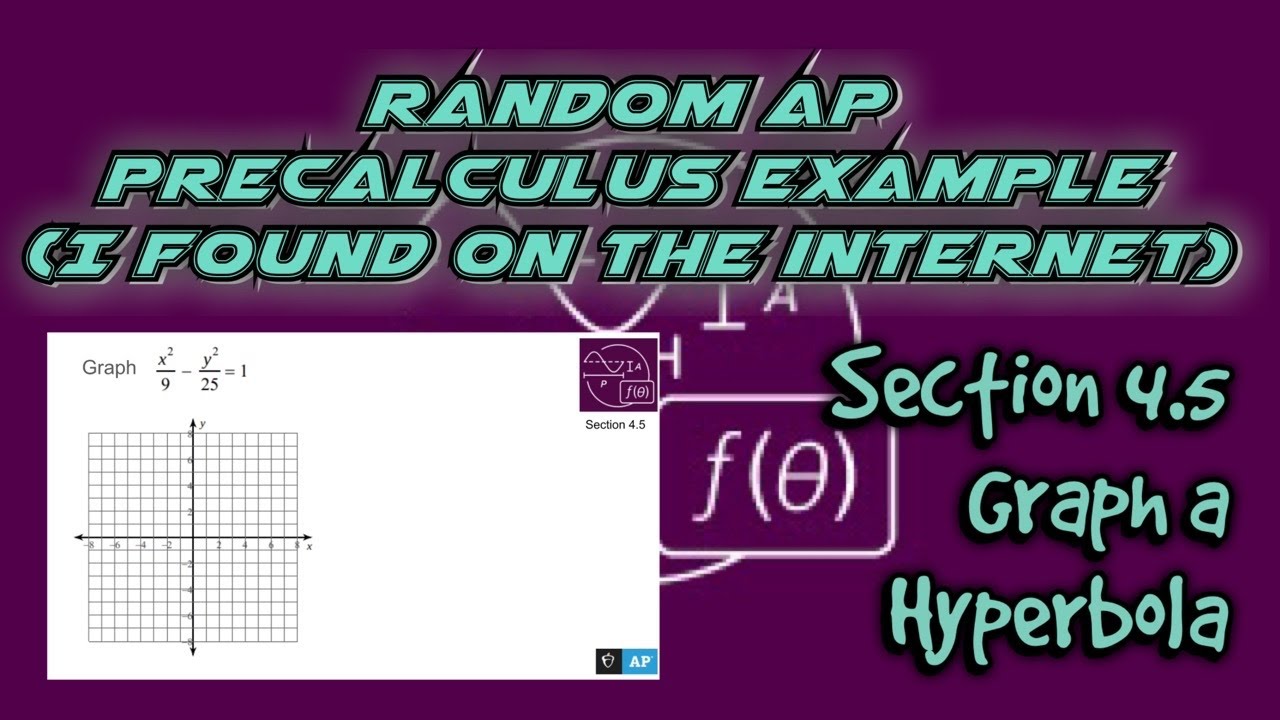
AP Precalculus Section 4.5 Example: Graph a Hyperbola (Example 1)

NOTE: Unit 4 is not included in the official AP Precalculus exam, but is still included in the curriculum.

Random AP Precalculus Problems (I found on the Internet). These are not official AP Collegeboard examples, but they will definitely get the job done!

To graph a hyperbola, follow these steps:

1. **Identify the Center**: Determine the center of the hyperbola, given by the coordinates \( (h, k) \).

2. **Determine the Asymptotes**: Find the equations of the asymptotes. The asymptotes pass through the center of the hyperbola and have slopes determined by the eccentricity of the hyperbola.

3. **Calculate the Vertices**: Determine the vertices of the hyperbola, which lie on the major axis. The distance from the center to each vertex is the length of the semi-major axis.

4. **Determine the Foci**: Find the foci of the hyperbola, which lie on the major axis. The distance from the center to each focus is \( c \), where \( c^2 = a^2 + b^2 \) and \( a \) is the length of the semi-major axis and \( b \) is the length of the semi-minor axis.

5. **Sketch the Hyperbola**: Sketch the hyperbola using the vertices, foci, and asymptotes as guides. The hyperbola will open either vertically or horizontally, depending on whether \( a \) or \( b \) is greater.

6. **Plot Additional Points**: Plot additional points on the hyperbola by using the equation of the hyperbola. You can choose values for \( x \) and solve for \( y \), or vice versa.

7. **Draw the Curve**: Connect the plotted points to draw the hyperbola.

8. **Label Key Points**: Label the center, vertices, foci, and any other significant points on the hyperbola.

By following these steps, you can accurately graph a hyperbola.

The Topics covered in AP Precalculus are...

1.1 Change in Tandem
1.2 Rates of Change
1.3 Rates of Change in Linear and Quadratic Functions
1.4 Polynomial Functions and Rates of Change
1.5 Polynomial Functions and Complex Zeros
1.6 Polynomial Functions and End Behavior
1.7 Rational Functions and End Behavior
1.8 Rational Functions and Zeros
1.9 Rational Functions and Vertical Asymptotes
1.10 Rational Functions and Holes
1.11 Equivalent Representations of Polynomial and Rational Expressions
1.12 Transformations of Functions
1.13 Function Model Selection and Assumption Articulation
1.14 Function Model Construction and Application
2.1 Change in Arithmetic and Geometric Sequences
2.2 Change in Linear and Exponential Functions
2.3 Exponential Functions
2.4 Exponential Function Manipulation
2.5 Exponential Function Context and Data Modeling
2.6 Competing Function Model Validation
2.7 Composition of Functions
2.8 Inverse Functions
2.9 Logarithmic Expressions
2.10 Inverses of Exponential Functions
2.11 Logarithmic Functions
2.12 Logarithmic Function Manipulation
2.13 Exponential and Logarithmic Equations and Inequalities
2.14 Logarithmic Function Context and Data Modeling
2.15 Semi-log Plots
3.1 Periodic Phenomena
3.2 Sine, Cosine, and Tangent
3.3 Sine and Cosine Function Values
3.4 Sine and Cosine Function Graphs
3.5 Sinusoidal Functions
3.6 Sinusoidal Function Transformations
3.7 Sinusoidal Function Context and Data Modeling
3.8 The Tangent Function
3.9 Inverse Trigonometric Functions
3.10 Trigonometric Equations and Inequalities
3.11 The Secant, Cosecant, and Cotangent Functions
3.12 Equivalent Representations of Trigonometric Functions
3.13 Trigonometry and Polar Coordinates
3.14 Polar Function Graphs
3.15 Rates of Change in Polar Functions
4.1 Parametric Functions
4.2 Parametric Functions Modeling Planar Motion
4.3 Parametric Functions and Rates of Change
4.4 Parametrically Defined Circles and Lines
4.5 Implicitly Defined Functions
4.6 Conic Sections
4.7 Parametrization of Implicitly Defined Functions
4.8 Vectors
4.9 Vector-Valued Functions
4.10 Matrices
4.11 The Inverse and Determinant of a Matrix
4.12 Linear Transformations and Matrices
4.13 Matrices as Functions
4.14 Matrices Modeling Contexts

I have many informative videos for Pre-Algebra, Algebra 1, Algebra 2, Geometry, Pre-Calculus, and Calculus. Please check it out:

/ nickperich

Nick Perich
Norristown Area High School
Norristown Area School District
Norristown, Pa

#APPrecalculus
#PreCalcProblems
#MathMinds
#AdvancedPreCalc
#TrigTales
#PrecalcPuzzles
#FunctionFiesta
#GraphGoals
#CalcReady
#PreCalcLife
#AlgebraicAdventures
#DerivativeDreams
#IntegrationInsights
#MathematicsMagic
#PreCalcReview
#PrecalcConcepts
#LogarithmLove
#TrigonometryTips
#MathMastermind
#APCalcPrep
#Mathematics
#MathMinds
#Math
#Maths