filmov
tv
This integral is actually one of your favorite constants

Показать описание
Complete solution development for this cool integral resulting in Apery's constant.
My complex analysis lectures:
If you like the videos and would like to support the channel:
You can follow me on Instagram for write ups that come in handy for my videos and DM me in case you need math help:
My LinkedIn:
Advanced MathWear:
My complex analysis lectures:
If you like the videos and would like to support the channel:
You can follow me on Instagram for write ups that come in handy for my videos and DM me in case you need math help:
My LinkedIn:
Advanced MathWear:
Integration and the fundamental theorem of calculus | Chapter 8, Essence of calculus
Math Integration Timelapse | Real-life Application of Calculus #math #maths #justicethetutor
I really like this integral
Use of Integration in REAL LIFE | why should we learn Integration? #integration #class12 #calculus
So much to learn/review in one integral!!!
Prove this, a double integral that equals to a single integral
You need to try this integral
This MAGICAL Contour Integral Is Perfect... (5K SPECIAL!)
INTRODUCTION TO INTEGRATION (BASIC FORMULAS)
This Infinite Integral Tower Actually Converges? Sorta...
MIT Integration Bee 2006 Regular Season, Round 1
Introduction to integral calculus | Accumulation and Riemann sums | AP Calculus AB | Khan Academy
The *Complex* Integral of (-1)^x
The p-integral Proof (type 1 improper integral)
One crazy looking integral
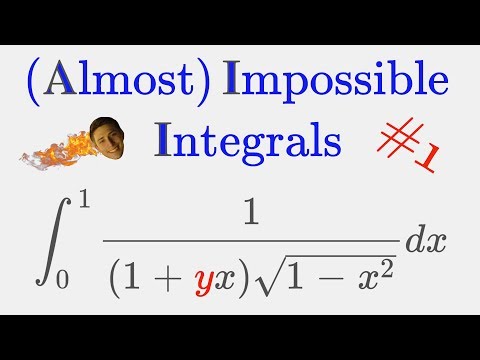
A Powerful Elementary Integral [ (Almost) Impossible Integrals #1 ]
A Cambridge Integral Experience
What my calculus 2 students did to this integral!
Can you solve this integral?
Understanding Integration (1 of 2: Different axes, methods of evaluating definite integrals)
a pervasive integration trick
🔥Seems difficult, but actually an EASY integral problem! #calculus #integration #math
Integration Tricks (That Teachers Won't Tell You) for Integral Calculus
What is Integration? Finding the Area Under a Curve
Комментарии
 0:20:46
0:20:46
 0:00:09
0:00:09
 0:18:17
0:18:17
 0:00:12
0:00:12
 0:36:39
0:36:39
 0:12:42
0:12:42
 0:16:51
0:16:51
 0:14:32
0:14:32
 0:30:54
0:30:54
 0:13:08
0:13:08
 0:46:20
0:46:20
 0:04:52
0:04:52
 0:00:51
0:00:51
 0:08:31
0:08:31
 0:13:06
0:13:06
 0:17:53
0:17:53
 0:29:03
0:29:03
 0:00:25
0:00:25
 0:19:56
0:19:56
 0:09:44
0:09:44
 0:00:55
0:00:55
 0:00:47
0:00:47
 0:11:26
0:11:26
 0:08:18
0:08:18