filmov
tv
A recent extension to Thevenin’s theorem and solution to ‘equivalent circuit riddle’

Показать описание
A recent extension to Thevenin’s theorem and solution to ‘equivalent circuit riddle’
Thevenin's theorem Solved Example | Electric Circuits | Network Analysis | Network Theory
Salsa Night in IIT Bombay #shorts #salsa #dance #iit #iitbombay #motivation #trending #viral #jee
Thevenin and Norton Theorem with Examples
Trying transition video for the first time 💙😂 || #transformation #transition #shorts #viralvideo...
IIT Bombay Lecture Hall | IIT Bombay Motivation | #shorts #ytshorts #iit
That's Why Mohit Sir Called 'God Of Mathematics'| Puzzle Brain teaser | #competishun ...
Girls Hostel Madness😂❤️ #shorts #short #girls #hostellife
Tough times Never last 😊✌️ #delhipolice #motivation
SIMULATION OF THEVENINS THEOREM USING PSPICE TOOL
EXPOSING LIES OUR PARENTS TOLD US GROWING UP?
This chapter closes now, for the next one to begin. 🥂✨.#iitbombay #convocation
How to melt iron in seconds 😲 I Step down transformer #shorts #experiment #science #physics
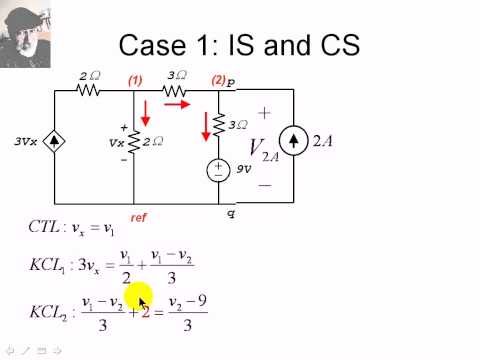
Thevenin's Theorem Problem 2 using the Nodal Method #circuittheory #thevenintheorem #nodalanaly...
Bridge circuits; Thevenin's theorem; Examples
Thevenin's and Norton's Theorems 16
New Paper on Vacuum Tube Circuit Analysis via Thevenin Equivalent Circuits (no paywall!)
AC Thévenin Equivalent Circuits
(2nd DRAFT) Final Tutorial on Thevenin and Norton Equivalents
Thevenins Theorem
How to eat Roti #SSB #SSB Preparation #Defence #Army #Best Defence Academy #OLQ
Electricité - Modéle Equivalent de Thévenin (MET)
Taking a Shortcut (Thevenin's Theorem) - Dr. McPheron Explains Ep. 12
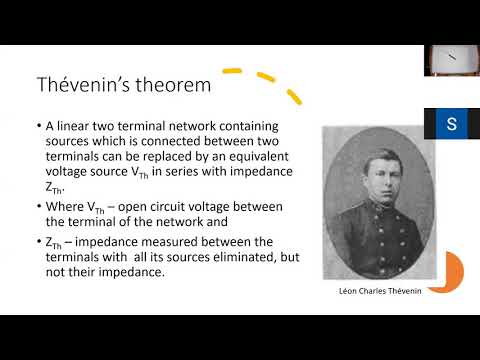
Thevenin\'s theorem
Комментарии
 0:17:56
0:17:56
 0:12:30
0:12:30
 0:00:14
0:00:14
 0:03:46
0:03:46
 0:00:15
0:00:15
 0:00:12
0:00:12
 0:00:19
0:00:19
 0:00:11
0:00:11
 0:00:22
0:00:22
 0:13:56
0:13:56
 0:00:53
0:00:53
 0:00:16
0:00:16
 0:01:00
0:01:00
 0:00:54
0:00:54
 0:38:24
0:38:24
 0:03:31
0:03:31
 0:03:18
0:03:18
 0:42:56
0:42:56
 0:32:49
0:32:49
 0:38:03
0:38:03
 0:00:16
0:00:16
 0:09:41
0:09:41
 0:02:56
0:02:56
 0:23:43
0:23:43