filmov
tv
Area of a Sector How to Find (Formula Radians)

Показать описание
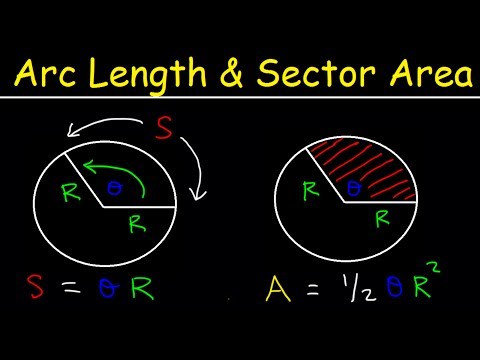
Learn how to find the Area of a Sector using radian angle measures in this free math video tutorial by Mario's Math Tutoring. We discuss what a sector is as well as the formula for finding the area of a sector with a central angle in radians.
0:06 What is a Sector
0:38 Formula for Area of a Sector
1:10 Example 1 Find Area of Sector with Radius 4 in and Central Angle of pi/6.
2:23 Example 2 Find Area of Sector with Radius 10 cm and Central Angle 150 Degrees.
2:53 Convert from Degrees to Radians
Related Videos:
Converting Degrees to Radians
Finding Area of a Sector Using Proportions
Looking to raise your math score on the ACT and new SAT?
Check out my Huge ACT Math Video Course and my Huge SAT Math Video Course for sale at
* Organized List of My Video Lessons to Help You Raise Your Scores & Pass Your Class. Videos Arranged by Math Subject as well as by Chapter/Topic. (Bookmark the Link Below)
For online 1-to-1 tutoring or more information about me see my website at:
0:06 What is a Sector
0:38 Formula for Area of a Sector
1:10 Example 1 Find Area of Sector with Radius 4 in and Central Angle of pi/6.
2:23 Example 2 Find Area of Sector with Radius 10 cm and Central Angle 150 Degrees.
2:53 Convert from Degrees to Radians
Related Videos:
Converting Degrees to Radians
Finding Area of a Sector Using Proportions
Looking to raise your math score on the ACT and new SAT?
Check out my Huge ACT Math Video Course and my Huge SAT Math Video Course for sale at
* Organized List of My Video Lessons to Help You Raise Your Scores & Pass Your Class. Videos Arranged by Math Subject as well as by Chapter/Topic. (Bookmark the Link Below)
For online 1-to-1 tutoring or more information about me see my website at:
GCSE Maths - Area of a Sector and Length of an Arc of a Circle (Circles Part 3) #108
Area of a Sector How to Find (Formula Radians)
Arc Length of a Circle Formula - Sector Area, Examples, Radians, In Terms of Pi, Trigonometry
How do we Find the Area of a Sector of a Circle? | Don't Memorise
Area of a Sector
How To Find The Area Of A Circle's Sector
Area of a Sector
HOW TO FIND ARC LENGTH AND SECTOR AREA OF CIRCLES | GEOMETRY
Finding the Area of a Sector
Area of a sector given a central angle | Circles | Geometry | Khan Academy
The Maths Prof: Area of Circle Sector
Circle Sectors - Areas and Arc Lengths | Grade 6+ Series | GCSE Maths Tutor
Perimeter and Area of a Sector
How to FIND THE AREA OF A SECTOR IN A CIRCLE
Arc Length and Area of a Sector in a Circle - SAT Math Part 42
Trigonometry - Find the area for a sector of a circle
Area of a Segment in a Circle
Radians, Arc Length & Sector Area of a Circle - [2-21-1]
Finding the area of a sector
Q308a, Arc length and Area of Sector of a circle
Sectors : finding the area of a sector using a calculator
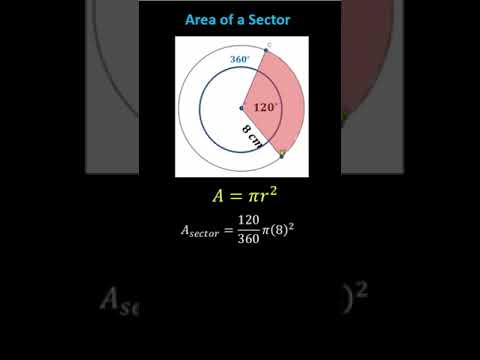
Area of a Sector Using Degrees
Area of a Sector and Arc Length
Length of an Arc, Area of a Sector (Circles) [IB Math AI SL/HL]
Комментарии
 0:05:39
0:05:39
 0:01:41
0:01:41
 0:15:57
0:15:57
 0:03:17
0:03:17
 0:04:14
0:04:14
 0:03:57
0:03:57
 0:03:23
0:03:23
 0:11:32
0:11:32
 0:11:21
0:11:21
 0:02:26
0:02:26
 0:04:48
0:04:48
 0:20:20
0:20:20
 0:12:10
0:12:10
 0:03:35
0:03:35
 0:06:25
0:06:25
 0:04:08
0:04:08
 0:04:23
0:04:23
 0:29:05
0:29:05
 0:04:21
0:04:21
 0:04:53
0:04:53
 0:01:04
0:01:04
 0:00:59
0:00:59
 0:09:04
0:09:04
 0:05:39
0:05:39