filmov
tv
Bounded Sequences and Sets in a Metric Space | Metric Spaces | Introduction to Real Analysis
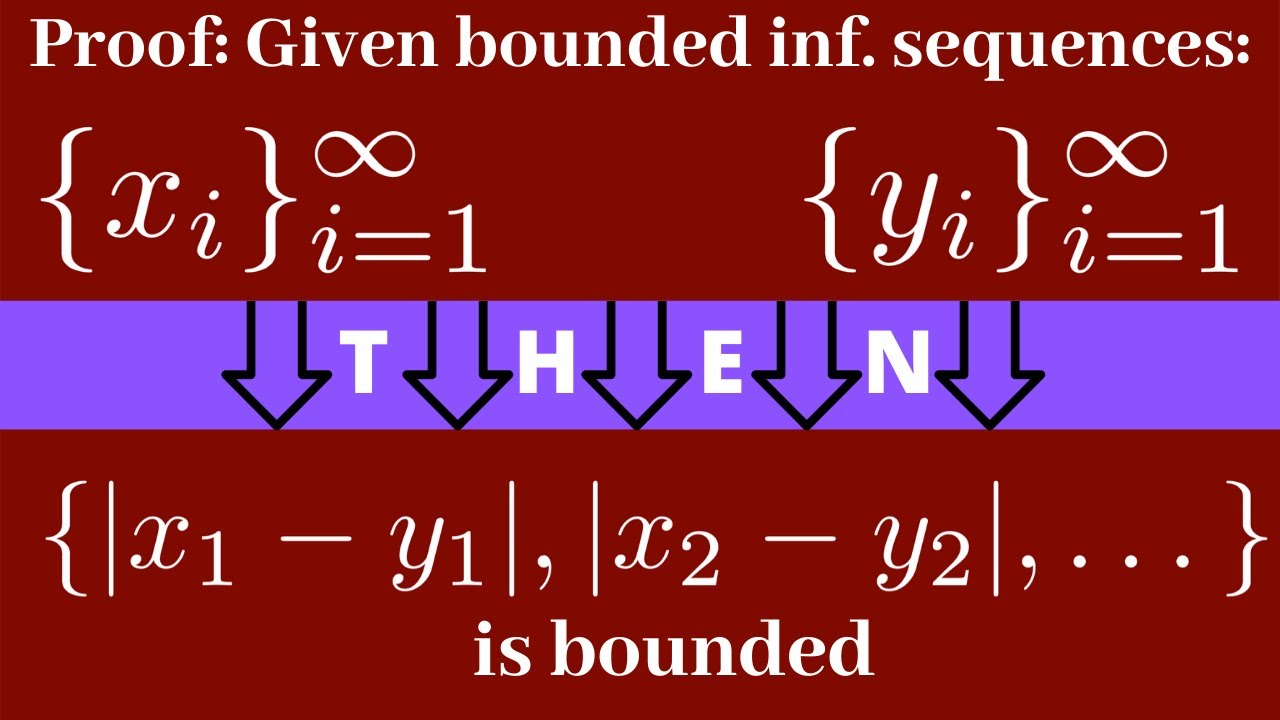
Показать описание
This video proves that if x = (x_1,x_2,...) and y=(y_1,y_2,...) are bounded infinite sequences, then the set {|x_1-y_1|, |x_2-y_2|, |x_3-y_3|,...} is bounded.
Proof: Bounded Sets Can Be Contained In Open or Closed Balls Centered Anywhere in a Metric Space
Proof that if (a-b) is less than epsilon and (b-a) is less than epsilon then |a-b| is less than epsilon:
Proof of: "a" less than "b" and "c" less than or equal to "d" implies (a+c) is strictly less than (b+d):
Proof: Bounded Sets Can Be Contained In Open or Closed Balls Centered Anywhere in a Metric Space
Proof that if (a-b) is less than epsilon and (b-a) is less than epsilon then |a-b| is less than epsilon:
Proof of: "a" less than "b" and "c" less than or equal to "d" implies (a+c) is strictly less than (b+d):
Monotonic Sequences and Bounded Sequences - Calculus 2
How to Determine if a Sequence is Bounded using the Definition: Example with a_n = 1/(2n + 3)
Bounded Sequences
What are Bounded Sequences? | Real Analysis
Bounded Sequences and Sets in a Metric Space | Metric Spaces | Introduction to Real Analysis
Bounded sequences (KristaKingMath)
Proof: Convergent Sequence is Bounded | Real Analysis
Ch 9-1 Monotonic and Bounded Sequences (Ex 8-9)
Calculus 2 - Sequences & Series: Bounded Sequences
How to Determine if a Sequence is Monotonic and Bounded: Example with n/(n^2 + 1)
Calculus 2, Section 8.1, part 5: Bounded and Unbounded Sequences
Intro to Monotonic and Bounded Sequences, Ex 1
How to Determine Whether a Sequence Bounded: Example with a_n = (2n - 3)/(3n + 4)
When is a sequence bounded? - Week 1 - Lecture 12 - Sequences and Series
Monotonic and Bounded Sequences | Calculus 2 | Math with Professor V
Converging and Diverging Sequences Using Limits - Practice Problems
Sequences - Video 5 - Bounded Sequences
Sequences (Examples on bounded sequence)
Types of bounded sequence
Calculus II - 9.1.3 Monotonic and Bounded Sequences
Real Analysis 3 | Bounded Sequences and Unique Limits
Bounded set #Bounded above and bounded below #mathematics#maths tricks for mcqs#fpsc ppsc
Bounded Sequence #sequence
Sequences - Increasing, decreasing, bounded and monotonic sequences
Комментарии
 0:31:23
0:31:23
 0:02:13
0:02:13
 0:04:13
0:04:13
 0:07:43
0:07:43
 0:09:27
0:09:27
 0:12:18
0:12:18
 0:05:47
0:05:47
 0:09:04
0:09:04
 0:06:57
0:06:57
 0:08:02
0:08:02
 0:06:16
0:06:16
 0:03:42
0:03:42
 0:01:22
0:01:22
 0:08:28
0:08:28
 0:41:57
0:41:57
 0:30:13
0:30:13
 0:05:31
0:05:31
 0:09:10
0:09:10
 0:00:07
0:00:07
 0:11:51
0:11:51
 0:09:35
0:09:35
 0:01:00
0:01:00
 0:00:15
0:00:15
 0:08:40
0:08:40