filmov
tv
Simplify Zero and Negative Exponents in these Few Steps
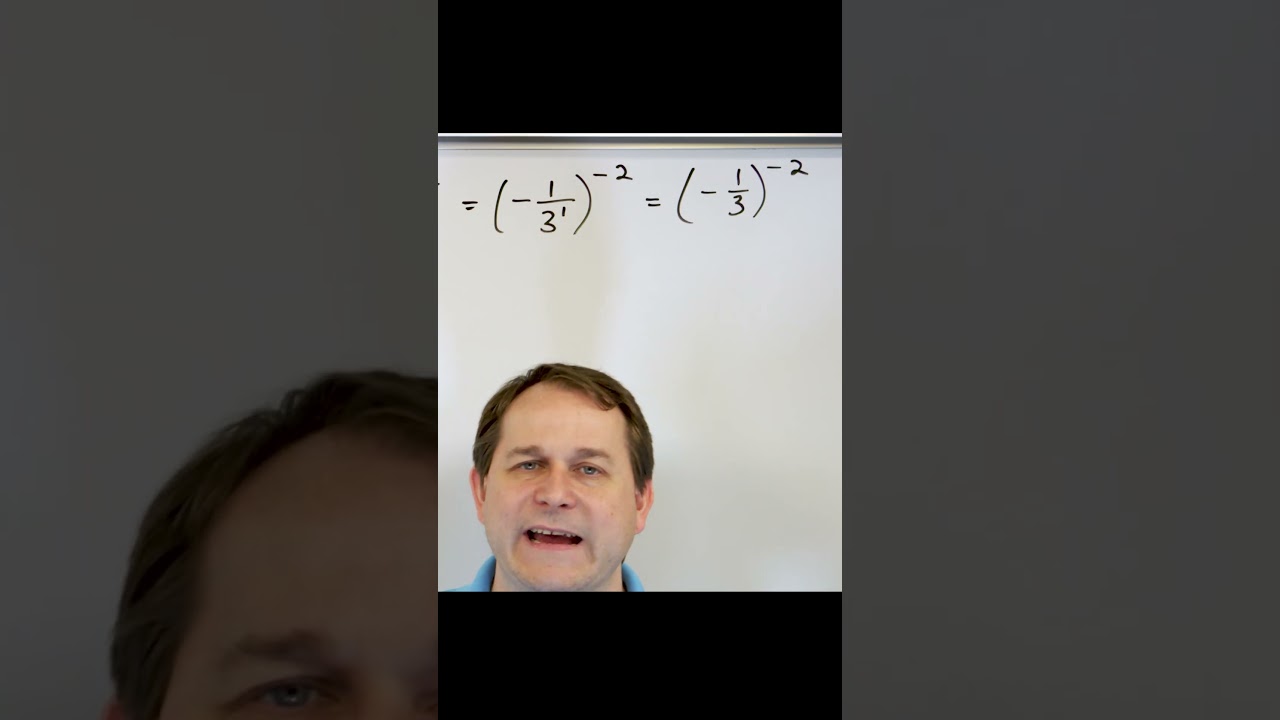
Показать описание
Zero and negative exponents are crucial concepts in algebra. A zero exponent signifies any non-zero number raised to the power of zero, resulting in the value of 1. Understanding this property aids in simplifying expressions and solving equations. Negative exponents, on the other hand, indicate the reciprocal of the corresponding positive exponent. For instance, x^(-n) is equivalent to 1/x^n. These rules facilitate handling expressions involving powers efficiently. Mastery of zero and negative exponents is foundational for more advanced algebraic manipulations, providing a solid understanding of the relationships between exponential expressions and their values.
Negative Exponents Explained!
Simplify zero and negative exponents
Simplify Zero and Negative Exponents in these Few Steps
03 - Negative Exponents & Powers of Zero (Laws of Exponents), Part 1
How to Simplify Negative Exponents - Rules of Exponents with Zero Power
Simplifying Exponents With Fractions, Variables, Negative Exponents, Multiplication & Division, ...
Negative Exponents | How to Solve Negative Exponents
Zero and Negative Exponents
Mathematics Grade 10 NOVEMBER 2022 PAPER 1 Memo @mathszoneafricanmotives
Simplifying Expressions with Zero and Negative Exponents
Simplify || Simplifying Exponent With Negative Exponents, Multiplication
Simplifying Expressions with Zero and Negative Exponents
Zero and Negative Exponents
How to Simplify Negative Exponents - Rules of Exponents with Zero Power
Simplify Each Expression with Exponents and Write without Negative Exponents
Mr. McNair Simplifying Zero and Negative Exponents
Zero, negative, and fractional exponents | Pre-Algebra | Khan Academy
Zero & Negative Exponents
How to simplify zero & negative exponents 3.6 gr 10 academic
❖ Simplifying Expressions with Negative Exponents - Ex 3 ❖
Algebra Basics: Laws Of Exponents - Math Antics
Define and Use Zero and Negative Exponents
Exponents (Negative & Zero)- Rules Explained & Examples Worked
Using multiple properties of exponents simplify the expression
Комментарии
 0:12:38
0:12:38
 0:04:09
0:04:09
 0:00:55
0:00:55
 0:32:47
0:32:47
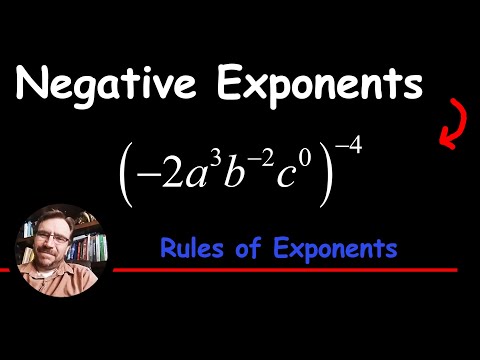 0:01:20
0:01:20
 0:11:48
0:11:48
 0:06:45
0:06:45
 0:06:27
0:06:27
 2:44:41
2:44:41
 0:08:51
0:08:51
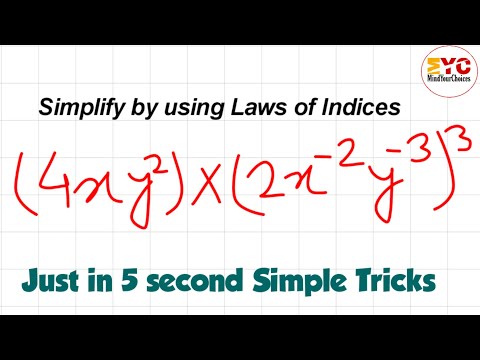 0:03:11
0:03:11
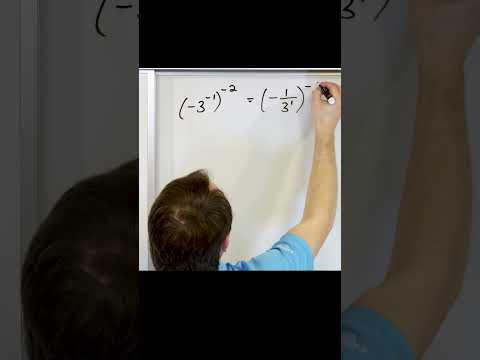 0:01:00
0:01:00
 0:01:57
0:01:57
 0:01:41
0:01:41
 0:02:52
0:02:52
 0:07:01
0:07:01
 0:14:04
0:14:04
 0:15:09
0:15:09
 0:14:24
0:14:24
 0:06:44
0:06:44
 0:13:46
0:13:46
 0:09:55
0:09:55
 0:32:54
0:32:54
 0:05:40
0:05:40