filmov
tv
How many squares are in different sizes of grids? #shorts

Показать описание
#shorts #short #math #mathematics #squares #grid #square #puzzle #formula #pattern
Combo Class is a crazy educational show where you can have fun learning rare things about math, science, language, and more! This is the SHORTS channel for faster-paced videos. Make sure you're also subscribed to the main Combo Class channel where full episodes go!
DISCLAIMER: any use of fire, tools, or other science experiments in this series is always done in a safe and professional way. Do not try to copy any actions you see in this series yourself.
Combo Class is a crazy educational show where you can have fun learning rare things about math, science, language, and more! This is the SHORTS channel for faster-paced videos. Make sure you're also subscribed to the main Combo Class channel where full episodes go!
DISCLAIMER: any use of fire, tools, or other science experiments in this series is always done in a safe and professional way. Do not try to copy any actions you see in this series yourself.
How Many Squares do you See?
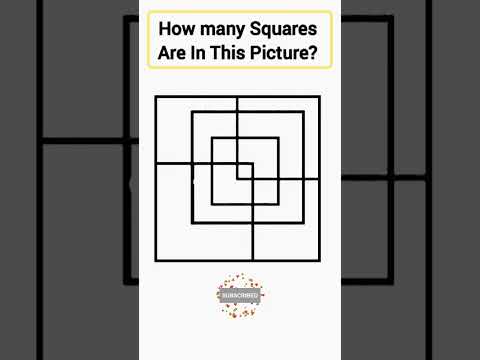
How To Solve 'How Many Squares Are In This Picture' - Learn The Formula!
How many squares are there?
How Many Squares (Solution)
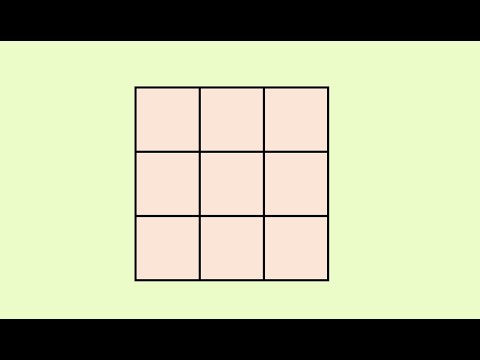
Singapore Math: How many squares can you find ?
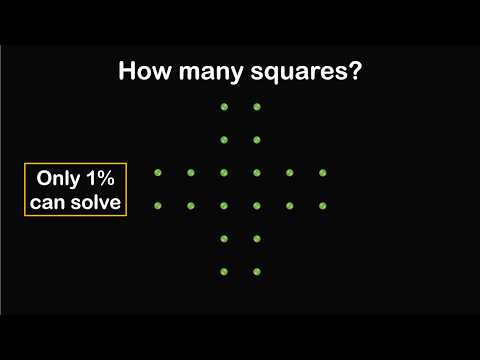
Only 1% of people can solve. How many squares?
The How Many Squares Puzzle
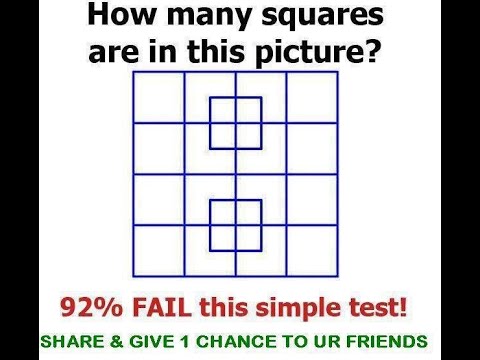
How Many Squares - Whatsapp Puzzle with Best Answer | How many squares are there in this picture ?
How many Squares are in the picture ? #shortfeed #shorts #eyetest #puzzle
Brain Test How many squares are there
How many squares so you see answer video - Here is my answer How many do you see?
👀 Eye Test - How Many Squares?
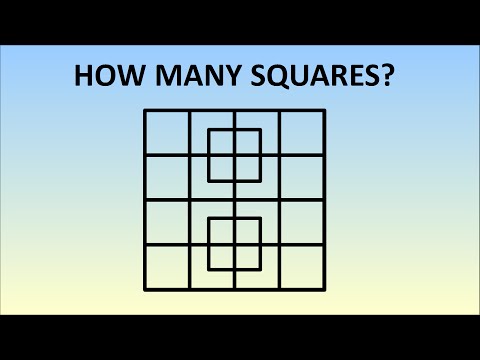
How many squares are in different sizes of grids? #shorts
How Many Squares/Rectangles/Triangles Are in Different Grids?
HOW MANY SQUARES ARE THERE?! #shorts #braintest #brainteaser #brainteasers
How Many Squares Are On A Chessboard?
How many squares on dots?
How many Squares Are In This Picture ? #shorts #subscribe #puzzle
How many squares are there in the following figure
How Many Squares?
How many squares in the picture?
How Many Squares in the Grid?
How many Squares are in this Picture?
How Many Squares on a Grid Hint
Комментарии
 0:00:43
0:00:43
 0:04:42
0:04:42
 0:00:26
0:00:26
 0:02:04
0:02:04
 0:12:00
0:12:00
 0:09:03
0:09:03
 0:00:42
0:00:42
 0:01:44
0:01:44
 0:00:13
0:00:13
 0:00:29
0:00:29
 0:00:21
0:00:21
 0:01:06
0:01:06
 0:00:49
0:00:49
 0:26:03
0:26:03
 0:00:30
0:00:30
 0:00:24
0:00:24
 0:00:43
0:00:43
 0:00:05
0:00:05
 0:01:09
0:01:09
 0:02:00
0:02:00
 0:10:01
0:10:01
 0:01:18
0:01:18
 0:01:02
0:01:02
 0:01:32
0:01:32