filmov
tv
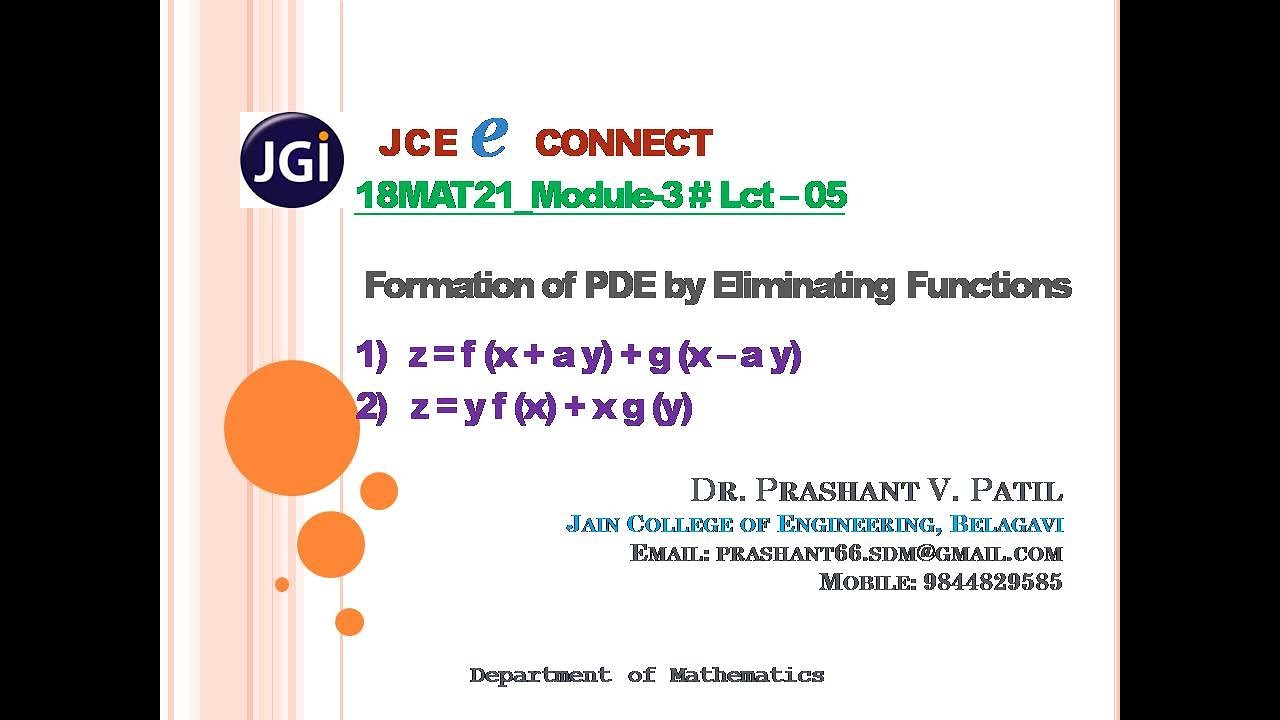
18MAT21_Module-3 #LCT 05 {PDE by eliminating functions 1)z=f(x+ay)+g(x-ay); 2)z=yf(x)+xg(y) }
Показать описание
This video describes easily how to eliminate two functions and to form second-order PDE.
0:08:08
18MAT21 module-3(PDE)
0:30:17
18MAT21 : Module 3 Partial Differential Equations Class 5
0:12:38
18MAT21_Module-3 #LCT 05 {PDE by eliminating functions 1)z=f(x+ay)+g(x-ay); 2)z=yf(x)+xg(y) }
0:12:01
18MAT21 Module-3 Formation of PDE by eliminating arbitrary functions
0:11:28
Solution of Wave Eqation @18MAT21 Module 3 # LCT 17
0:11:26
Module-3: 18MAT21 (PDE)
0:15:44
18MAT21, Module 3 PDE_Part8
0:21:04
18MAT21:Derivation and solution of one dimensional heat equation
0:24:57
Formation of PDEs by eliminating arbitrary functions | VTU Mathematics | Module 3
0:08:42
Solution of one dimensional Heat equation || PDE || 21MAT21 || 18MAT21 || Dr Prashant Patil
0:10:43
Module -3 : PDE video -1
0:14:00
LCT 01@18mat21 _Module 03 # Formation of PDE by eliminating arbitrary constants.
0:10:42
Solution of Nonhomogeneous || Differential Equations || 18mat21 || Dr Prashant Patil
0:27:12
18MAT21 Module no 5
0:15:13
18MAT21_Module 3 # LCT 03 {Formation of PDE by eliminating arbitrary functions}
0:03:14
#8 || Problem#4 || Form a PDE by eliminating the arbitrary function of the equation𝒛=𝒆^𝒚 𝒇(𝒙+𝒚) ||...
0:15:09
vector integration 3 18MAT21 VTU SYLLABUS
0:38:17
Formation of PDE by eliminating arbitrary function | Part 3
0:02:47
#9 || Problem#5 || PDE by eliminating the arbitrary function of the equation 𝒛=𝒙+𝒚+𝒇(𝒙𝒚) || 18MAT21...
0:11:40
Orthogonality of analytic function in polar form || Complex Analysis || 18mat21 || Dr Prashant Patil
0:07:09
18MAT21 mod 5 numerical integration by Prof Akshata
0:18:17
18mat21/ module 2 / class 4
0:16:57
Derivation of 1-D Heat Equation
0:18:21
Example on One Dimensional Heat Equation || Numerical Methods || Dr Prashant Patil
Комментарии