filmov
tv
Problem 1.3 | Griffiths' Introduction to Quantum Mechanics | 3rd Edition

Показать описание
Problem 1.3
Consider the gaussian distribution ρ(x) = Ae^(−λ(x−a)^2)), where A, a, and λ are positive real constants. (The necessary integrals are inside the back cover.)
(a) Use Equation 1.16 to determine A.
(b) Find {x}, {x^2}, and σ.
(c) Sketch the graph of ρ(x).
In this video, we solve Problem 1.3 in Griffiths' Introduction to Quantum Mechanics (3rd Edition) as part of a series of solutions to the textbook's questions.
Consider the gaussian distribution ρ(x) = Ae^(−λ(x−a)^2)), where A, a, and λ are positive real constants. (The necessary integrals are inside the back cover.)
(a) Use Equation 1.16 to determine A.
(b) Find {x}, {x^2}, and σ.
(c) Sketch the graph of ρ(x).
In this video, we solve Problem 1.3 in Griffiths' Introduction to Quantum Mechanics (3rd Edition) as part of a series of solutions to the textbook's questions.
Problem 1.3 | Griffiths' Introduction to Quantum Mechanics | 3rd Edition
Problem 1.3a | Introduction to Quantum Mechanics (Griffiths)
Problem 1.1 | Griffiths' Introduction to Quantum Mechanics | 3rd Edition
Griffiths QM Problem 3.1
Griffiths Electrodynamics Problem 1.3
Griffiths Electrodynamics 3.1 Problem Solution
David Griffiths Electrodynamics | Problem 3.1 Solution
Griffiths Electrodynamics Problem 1.3
Griffiths QM Problem 1.3: Gaussian Distribution
Problem 1.3b, c | Introduction to Quantum Mechanics (Griffiths)
Problem 2.11a (Part 1) | Introduction to Quantum Mechanics (Griffiths)
Solution to Griffiths Introduction to QM Problem 6.31 (3rd edition) 6.36 (2nd edition) Stark effect
Griffiths, Quantum Mechanics, Problems 1.1-1.4
Griffiths Introduction to QM Problem 6.30: Using Kramer's relation to find expectation value of...
Problem 1.3 Solution | Griffiths Introduction to Electrodynamics | Prof. Dr. Mudassir Ali Shah
NEWYES Calculator VS Casio calculator
Problem 1.10 | Griffiths' Introduction to Quantum Mechanics | 3rd Edition
Griffiths Intro to QM Problem 9.1: Hydrogen Atom in Time dependent Electric field
Problem 2.33 (Part 1) | Introduction to Quantum Mechanics (Griffiths)
Problem 1.11 | Griffiths' Introduction to Quantum Mechanics | 3rd Edition
Jeff Bezos Quit Being A Physicist
Griffiths QM Problem 4.13 (3rd ed.) Finding Expectation Values for Hydrogen Atom
Problem 6.1 | Introduction to Quantum Mechanics (Griffiths)
Problem 2.38b | Introduction to Quantum Mechanics (Griffiths)
Комментарии
 0:21:16
0:21:16
 0:02:50
0:02:50
 0:11:58
0:11:58
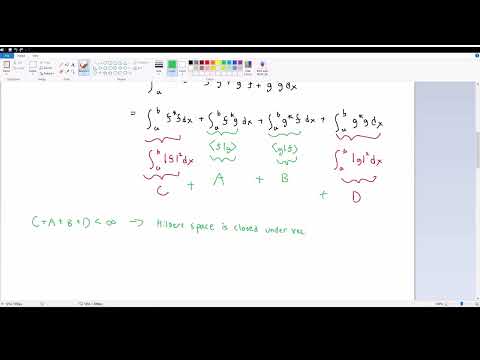 0:19:34
0:19:34
 0:14:23
0:14:23
 0:08:22
0:08:22
 0:13:33
0:13:33
 0:04:27
0:04:27
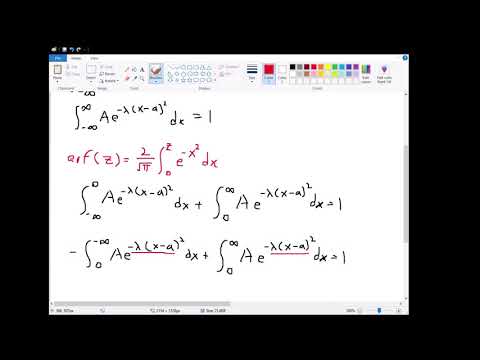 0:13:33
0:13:33
 0:10:30
0:10:30
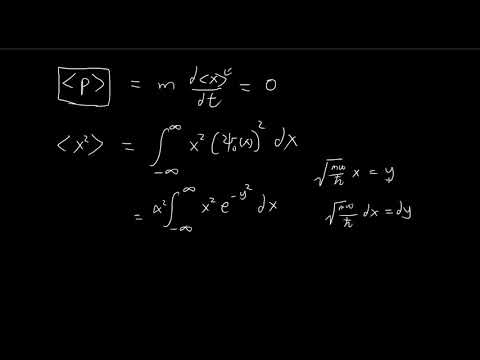 0:08:11
0:08:11
 1:13:00
1:13:00
 0:10:54
0:10:54
 0:23:30
0:23:30
 0:06:20
0:06:20
 0:00:14
0:00:14
 0:08:09
0:08:09
 0:26:48
0:26:48
 0:06:43
0:06:43
 0:27:17
0:27:17
 0:00:56
0:00:56
 0:23:31
0:23:31
 0:13:46
0:13:46
 0:02:06
0:02:06