filmov
tv
One Man's Modus Ponens (Is Another Man's Modus Tollens)

Показать описание
An explanation of the phrase "One Man's Modus Ponens is another Man's Modus Tollens" as well as definitions of the logical forms of modus ponens and modus tollens and an example from G.E. Moore.
Sponsors: Joshua Furman, Joshua Opell, NBA_Ruby, Eugene SY, Antoinemp1, Antibody, Ismail Fagundes, Adrien Ecoffet, Tom Amedro, Christopher McGevna, Joao Sa, and Dennis Sexton. Thanks for your support!
Information for this video gathered from The Stanford Encyclopedia of Philosophy, The Internet Encyclopedia of Philosophy, The Cambridge Dictionary of Philosophy, The Oxford Dictionary of Philosophy and more!
(#Logic, #ModusPonens)
Sponsors: Joshua Furman, Joshua Opell, NBA_Ruby, Eugene SY, Antoinemp1, Antibody, Ismail Fagundes, Adrien Ecoffet, Tom Amedro, Christopher McGevna, Joao Sa, and Dennis Sexton. Thanks for your support!
Information for this video gathered from The Stanford Encyclopedia of Philosophy, The Internet Encyclopedia of Philosophy, The Cambridge Dictionary of Philosophy, The Oxford Dictionary of Philosophy and more!
(#Logic, #ModusPonens)
One Man's Modus Ponens (Is Another Man's Modus Tollens)
Logical Arguments - Modus Ponens & Modus Tollens
Argument Schemes: Modus Ponens
Argument Schemes: Modus Tollens
Analyzing an argument for validity
How to Argue - Philosophical Reasoning: Crash Course Philosophy #2
Modus Ponens, or, Affirming the Antecedent (valid)
Boolean Proof Example 1: Using Modus Ponens
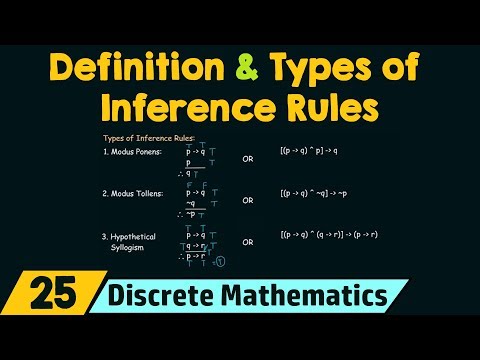
Rules of Inference - Definition & Types of Inference Rules
Modus Ponens, Affirming the Consequent, Modus Tollens, Denying the Antecedent
Conditionals, Modus Ponens, and Modus Tollens
Modus Tollens & Affirming the Consequent
Lecture: Unit 6 Single Place Derivations in PL Part I
Valid & Invalid Arguments — informal logic part 3
Curry's Paradox
CRITICAL THINKING - Fallacies: Formal and Informal Fallacies
14. Rules of Inference ||Modus Ponens, Modus Tollens, Hypothetical Syllogism, Disjunctive Syllogism
Logic 101 (#32): Destructive Dilemma
What is an Argument? – Informal Logic part 1
Common Deductive Forms & Necessary/Sufficient Conditions
Logic Lesson 4: Rules of Inference for Dilemmas
Natural Deduction - Using Assumptions
Introduction to Formal Logic (Part 1)
Rules for Disjunctive and Hypothetical Syllogisms
Комментарии
 0:05:21
0:05:21
 0:08:44
0:08:44
 0:02:30
0:02:30
 0:01:40
0:01:40
 0:02:13
0:02:13
 0:09:43
0:09:43
 0:00:16
0:00:16
 0:04:00
0:04:00
 0:07:44
0:07:44
 0:19:02
0:19:02
 0:56:54
0:56:54
 0:07:38
0:07:38
 0:30:32
0:30:32
 0:06:52
0:06:52
 0:15:15
0:15:15
 0:07:05
0:07:05
 0:09:43
0:09:43
 0:05:05
0:05:05
 0:05:48
0:05:48
 0:33:21
0:33:21
 0:21:54
0:21:54
 0:22:15
0:22:15
 0:25:29
0:25:29
 0:06:11
0:06:11