Schwarzschild geodesics - 12

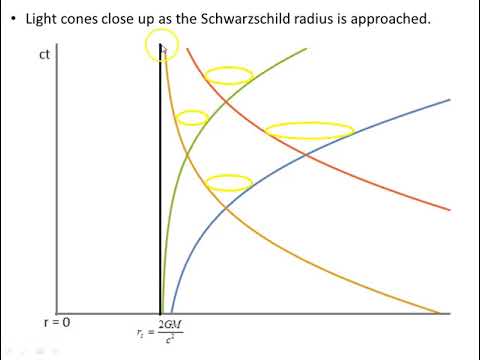
This video investigates the radial motion of photons in a Schwarzschild space-time for both the ingoing and outgoing directions. It does this using plots of these geodesics and shows how the local light cones behave in this geometry. The region within the Schwarzschild radius is investigated where the light cones tip over and point towards the singularity.

[vicentematricardi]

Siempre estoy pendiente de Sus videos , que tenga un excelente 2019 , Muchas Gracias, thank you very much

[Cosmalano]

When the light cone closes as the observer approaches the horizon, does this correspond to their “future” containing less and less space? As in, less and less things are contained within their future lightcone as they approach, which would’ve otherwise been in their light come were the lighcone not squished? Or does it warp the geometry to keep these objects causally connected normally?

What is the difference between the horizon and the singularity for an infalling observer? To an outside observer the horizon is present but to an infalling one it’s never felt, it’s a coordinate horizon. So the distant observer sees the other observer fall in but never really “fall in”. They will just inch ever closer until the end of time (or the end of the blackhole). So doesn’t this mean falling into the blackhole’s horizon would look the same as falling into the true singularity? Or is the “true singularity” also not real and actually has proper physical characteristics?

Finally, doesn't the information this model produces about interior null geodesics show a blatant contradiction with general covariance? If locally Lorentz covariance still holds in regions inside the horizon relative to outside observers (which it must to preserve the principle of equivalence, something this model seems to also violate) then how could the “speed” of these geodesics explode to infinity unless these geodesics were indicating that locally spacetime was expanding? And also how could this behavior actually happen at the horizon if the horizon is just a coordinate singularity?

Great videos, I’m about to go leave a comment on another in this miniseries.